In the figure, □P=90o. Sides of triangle APC are extended to B and D.
Prove that, the circles drawn with the sides of a quadrilateral with perpendicular diagonals, will meet at a common point.
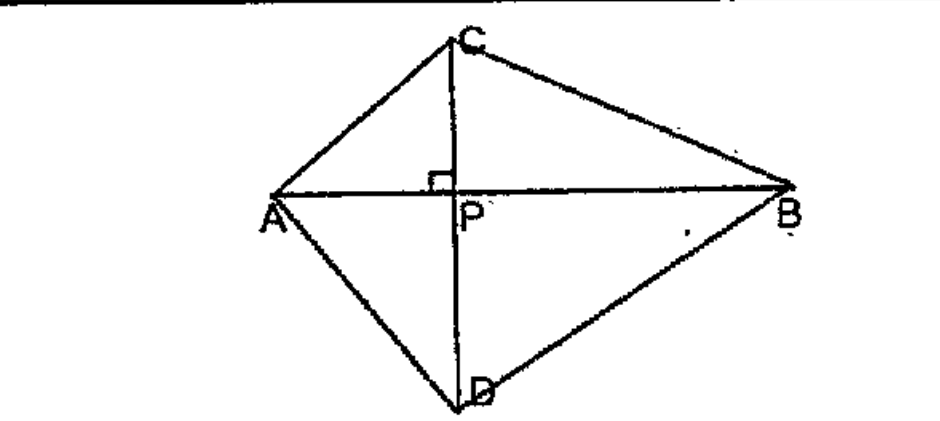 .Solution in Malayalam
.Solution in Malayalam
Step by step video & image solution for In the figure, squareP=90^o. Sides of triangle APC are extended to B and D. Prove that, the circles drawn with the sides of a quadrilateral with perpendicular diagonals, will meet at a common point. . by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In the figure, O is the centre of the circle and A,B,C,D are points on it.
If ∠B=110o, then
What is the measure of ∠D?
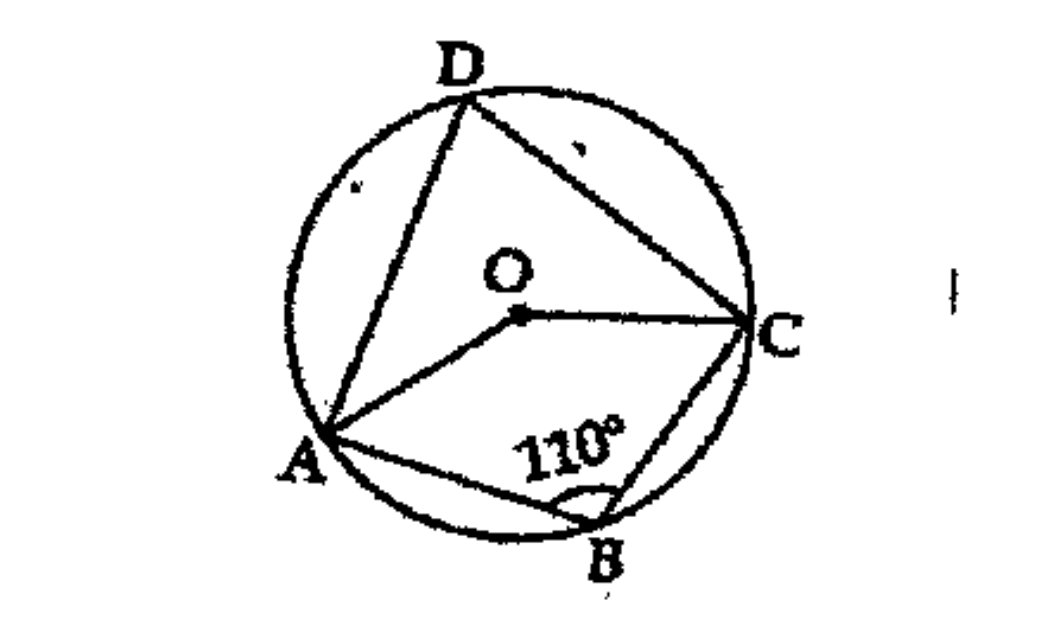 .
.
View SolutionIn the figure O is the centre of circle. □AOC=80o
What is the measure of □ABC?
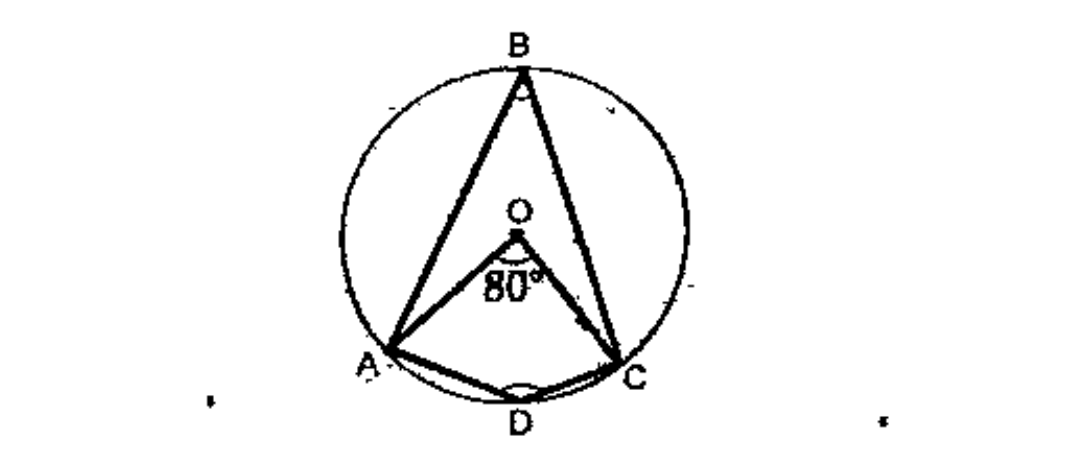 .
.
View SolutionIn the given figure (not to scale), O is the centre of the circle. A,B,C and D are concyclic and AB=CD . If /_MON =120^(@) , then find /_ OPN .
View SolutionHow many lines of symmetry does the above figure have ?
View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionDtermine the images of the following figure about the given line :
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionFind the area of the figure given below.

View SolutionThe area of the shaded part in the figure given below is ______.

View SolutionThe inequation represented by the graph given below is :
View SolutionThe inequation that best describes the graph given below is _________
View SolutionThe inequation that best describes the following graph is _________
View SolutionWhich inequations represent the shaded region in the given figure
View SolutionIn the following figure, if O is the center of the circle and ¯¯¯¯¯¯AB is the diameter, then find ∠ACB.
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C12_E02_009_Q01.png" width="80%"gt
View Solution
