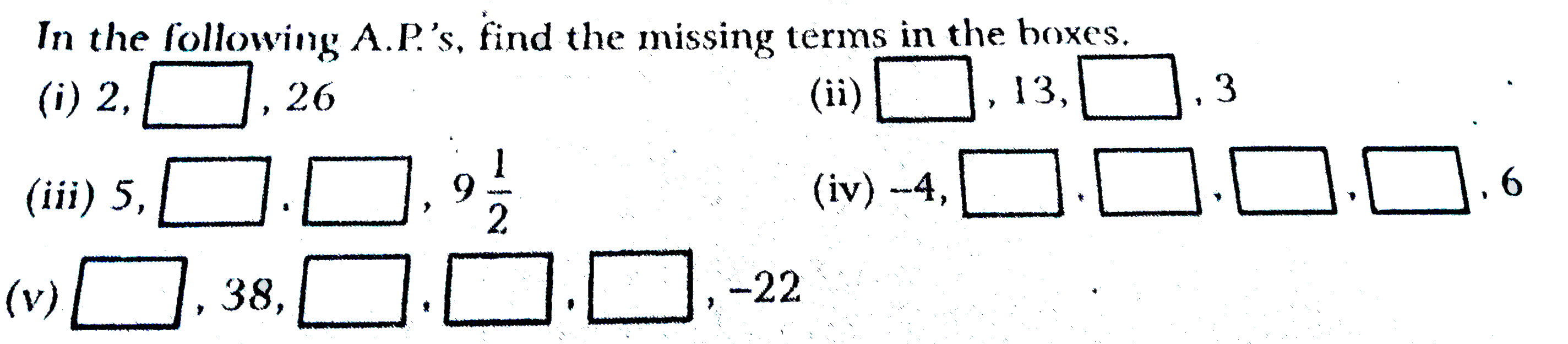
In the following A.P.'s, find the missing terms in the boxes.

Video Solution

Text Solution

Verified by Experts

(i) $2, □, 26$
$\Rightarrow 2, x, 26$ are in A.P. (say)
$\Rightarrow x = \frac{2 + 26}{2} = 14$
(ii) $□, 13, □, 3$
$\Rightarrow x, 13, y, 3$ are in A.P. (say)
$∴ Y = \frac{13 + 3}{2} = 8$
and $\frac{x + y}{2} = 13$
$\Rightarrow x + y = 26$
$\Rightarrow x + 8 = 26$
$\Rightarrow x = 18$
$\Rightarrow x = 18, y = 8$
(ii) $5, □, □, 9 \frac{1}{2}$
$\Rightarrow 5, x, y, \frac{19}{2}$ are in A.P.. (say)
Here, a=5
and $a_{4} = \frac{19}{2}$
$\Rightarrow 5 + 3 d = \frac{19}{2}$
$\Rightarrow 3 d = \frac{19}{2} - 5 = \frac{9}{2} \Rightarrow d = \frac{3}{2}$
$∴ x = 5 + d = 5 + \frac{3}{2} = \frac{13}{2}$
and $y = 5 + 2 d = 5 + 2 \times \frac{3}{2} = 8$
$∴ x = \frac{13}{2}, y = 8$
(iv) $- 4 □, □, □, □, 6$
$\Rightarrow - 4, p, q, r, s, 6$ are in A.P. (say)
Here, $a = - 4$ and $a_{6} = 6$
$\Rightarrow - 4 + 5 d = 6$
$\Rightarrow 5 d = 10 \Rightarrow d = 2$
$∴ p = - 4 + d = - 4 + 2 = - 2$
$q = - 4 + 2 d = - 4 + 2 \times 2 = 0$
$r = - 4 + 3 d = - 4 + 3 \times 2 = 2$
$s = - 4 + 4 d = - 4 + 4 \times 2 = 4$
$p = - 2, q = 0, r = 2, s = 4$
(v) $□, 38, □, □, □, - 22$
$\Rightarrow p, 38, q, r, s, - 22$ are in A.P. (say)
Here, $a_{2} = 38$
$\Rightarrow p + d = 38 ... (1)$
and $a_{6} = - 22$
$\Rightarrow p + 5 d = - 22 ... (2)$
Subtract equation (1) from (2), we get
$\begin{matrix} p + 5 d = - 22 - p + - d = 38 - ¯ ¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯ ¯ 4 d = - 60 \end{matrix}$
$\Rightarrow d = - 15$
put d=-15 in equation (1), we get
p+(-15)=38
$\Rightarrow p = 53$
$∴ q = 38 + d = 38 + (- 15) = 23$
$r = q + d = 23 + (- 15) = 8$
$s = r + d = 8 + (- 15) = - 7$
$∴ p = 53, q = 23, r = 8, s = - 7$

Updated on:21/07/2023