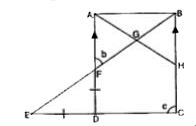
In the given figure , if a= b = c then ∠AOC= ______ ??
The correct Answer is:60 degree
To solve the problem, we need to find the value of angle AOC given that angles A, B, and C are equal (a = b = c). Here’s a step-by-step solution:
Step 1: Understand the relationship between the angles
We know that angles A, B, and C are equal. Therefore, we can denote them as:
- Angle A = a
- Angle B = a
- Angle C = a
Step 2: Use the property of angles
From the problem, we also know that the sum of angles A, B, and C is equal to 90 degrees because the lines OD and AO are perpendicular to each other. Thus, we can write:
Angle A+Angle B+Angle C=90∘
Substituting the values we have:
a+a+a=90∘
This simplifies to:
3a=90∘
Step 3: Solve for angle A
To find the value of angle A, we divide both sides of the equation by 3:
a=90∘3=30∘
Thus, we have:
- Angle A = 30 degrees
- Angle B = 30 degrees
- Angle C = 30 degrees
Step 4: Find angle AOC
Now, we need to find angle AOC, which is the sum of angles A and B:
Angle AOC=Angle A+Angle B
Substituting the values we found:
Angle AOC=30∘+30∘=60∘
Final Answer
Therefore, the value of angle AOC is:
Angle AOC=60∘
---
We know that angles A, B, and C are equal. Therefore, we can denote them as:
- Angle A = a
- Angle B = a
- Angle C = a
From the problem, we also know that the sum of angles A, B, and C is equal to 90 degrees because the lines OD and AO are perpendicular to each other. Thus, we can write:
Angle A+Angle B+Angle C=90∘
Substituting the values we have:
a+a+a=90∘
This simplifies to:
3a=90∘
To find the value of angle A, we divide both sides of the equation by 3:
a=90∘3=30∘
Thus, we have:
- Angle A = 30 degrees
- Angle B = 30 degrees
- Angle C = 30 degrees
Now, we need to find angle AOC, which is the sum of angles A and B:
Angle AOC=Angle A+Angle B
Substituting the values we found:
Angle AOC=30∘+30∘=60∘
Therefore, the value of angle AOC is:
Angle AOC=60∘
Topper's Solved these Questions
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - TRIANGLESEXERCISE - PART-A (Fill in the blanks )10videos
TRIANGLES
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - TRIANGLESEXERCISE - PART-A (State which of the following statements are true and false )5videos
TRIANGLES
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - Practice Test8videos
SURFACE AREAS AND VOLUMES
Similar Questions
Knowledge Check
- Question 1 - Select One
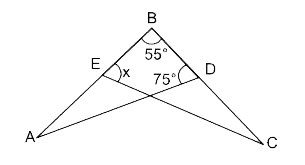
In the given figure, AD||BC, ∠AFG=band∠BCD=c. Express b in terms of c.
 Ac2B90∘+c2C180∘−c2D90∘−c2
Ac2B90∘+c2C180∘−c2D90∘−c2 - Question 2 - Select One
In the given figure , x in terms of a , b and c is
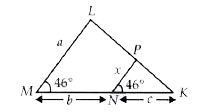 Ax=acb+cBx=abb+cCx=aca+bDx=bca+c
Ax=acb+cBx=abb+cCx=aca+bDx=bca+c - Question 3 - Select One
In the given figure,the angle of reflection is
 A30∘B60∘C45∘DNone of these
A30∘B60∘C45∘DNone of these
In the given figure, prove that x=a+b+c.
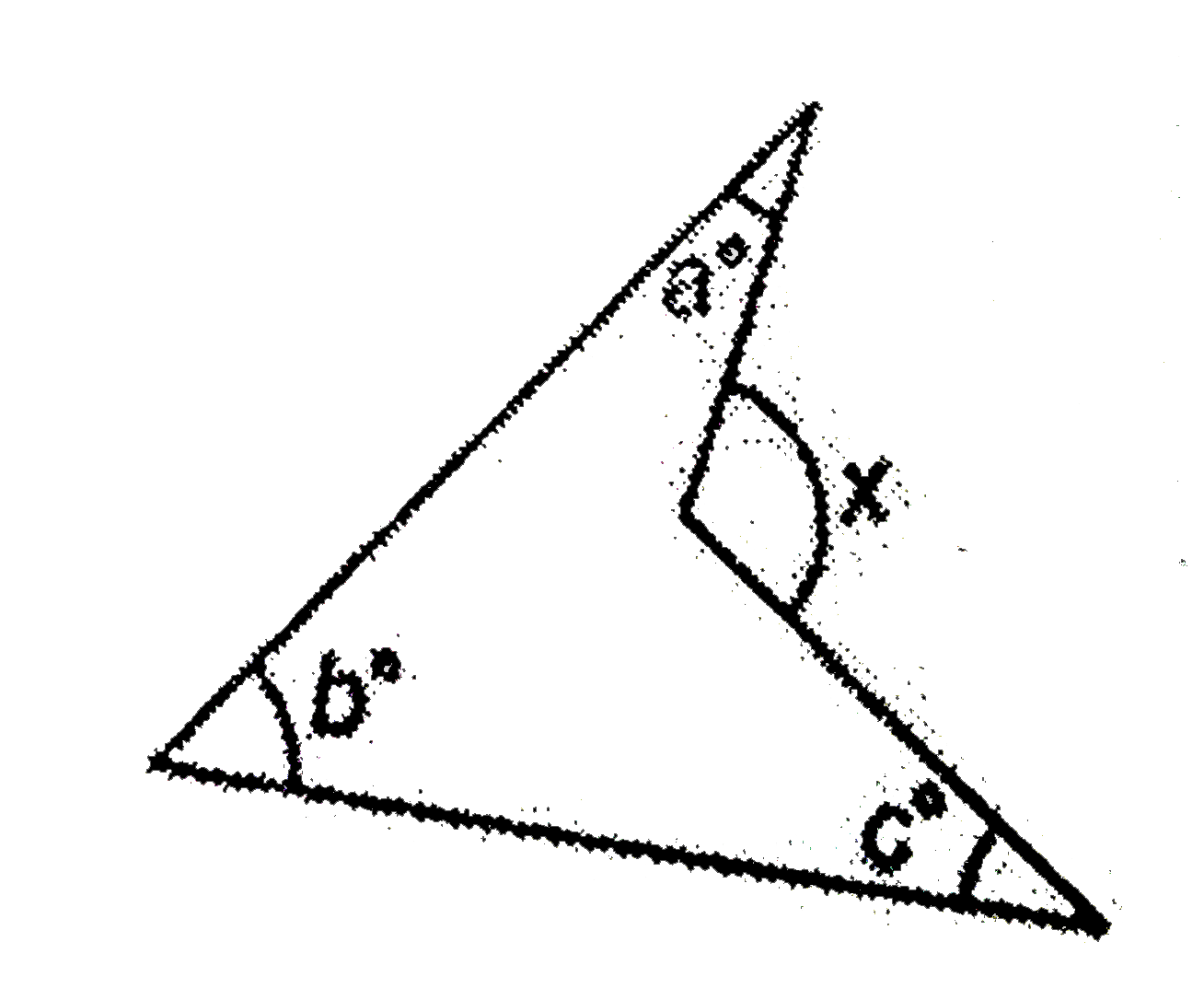 View Solution
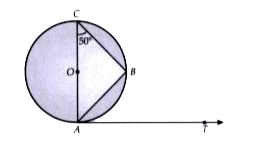
View SolutionIn the given figure A,B is a chord of the circle and AOC is its diameter ,such that ∠ACB=50∘ .If AT is the tangent to the circle at the point A, then ∠BAT is equal to :
 View Solution
View SolutionIn the given figure, OC.OD = OA.OB
Show that : ∠A=∠D and ∠C=∠B.
 View Solution
View SolutionIdentify the given figures A,B,C:-
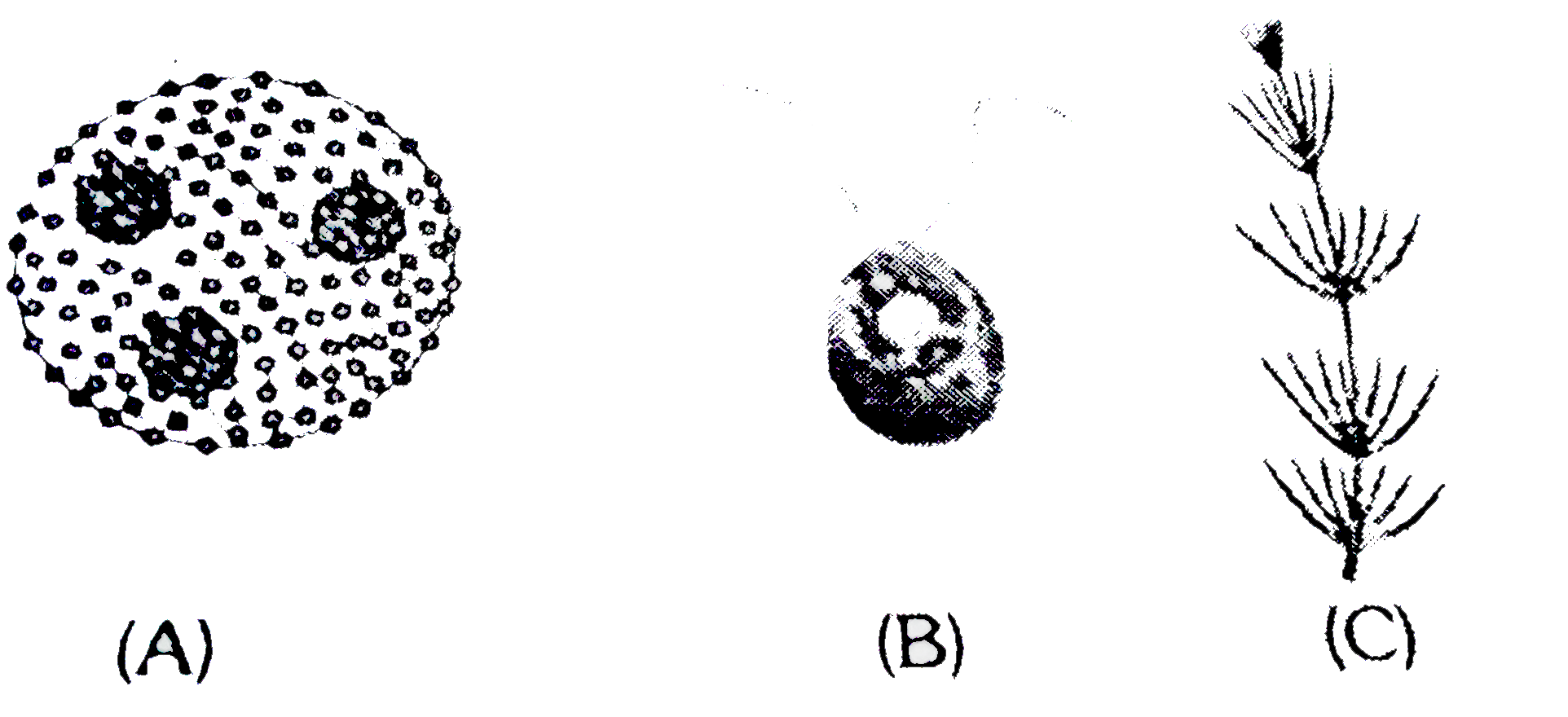 View Solution
View SolutionIn the given figure if find, ∠AOC=45∘, find ∠BOD
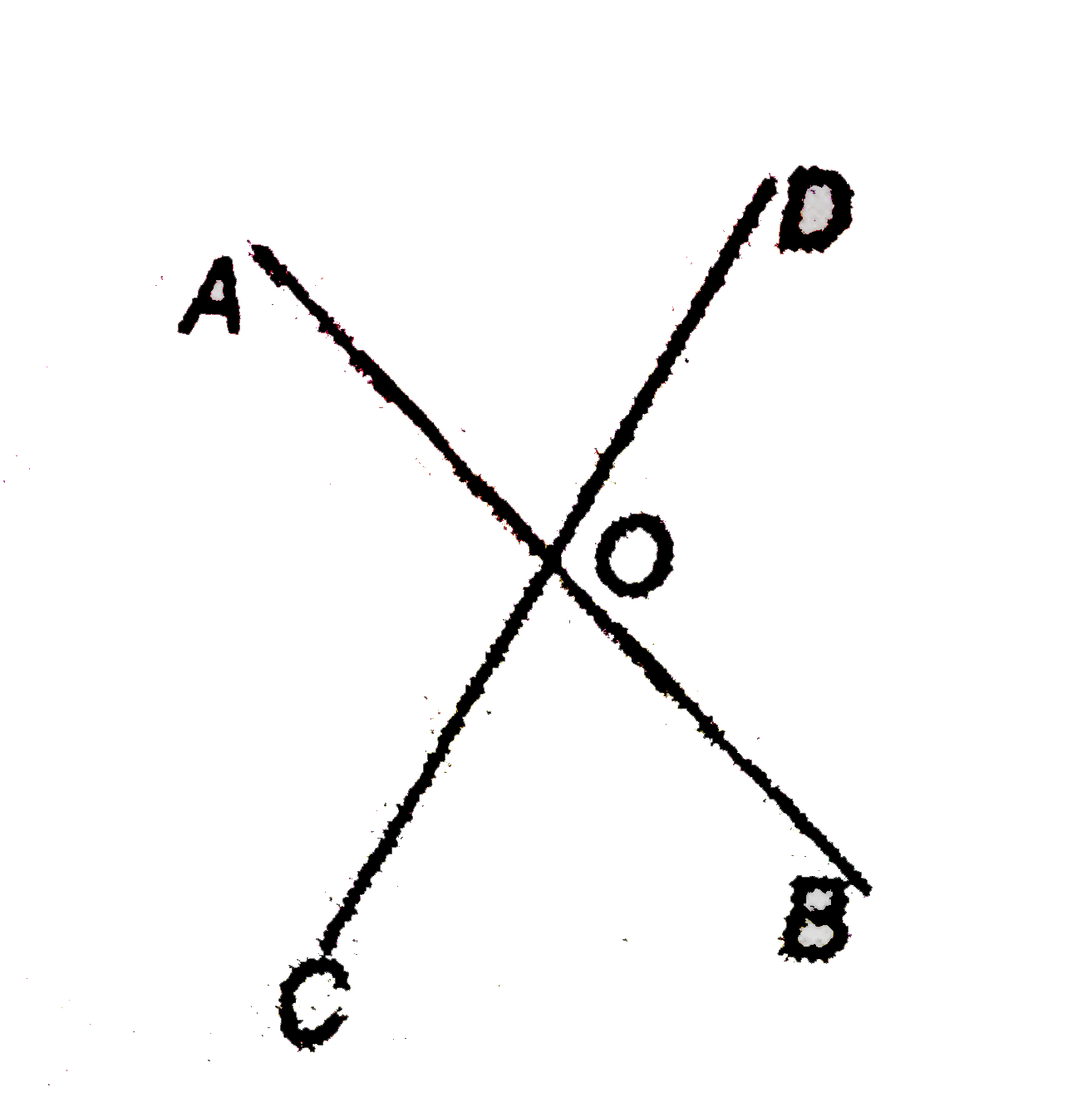 View Solution
View Solution