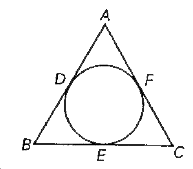
In the given figure, if AB=AC, prove that BE=EC.
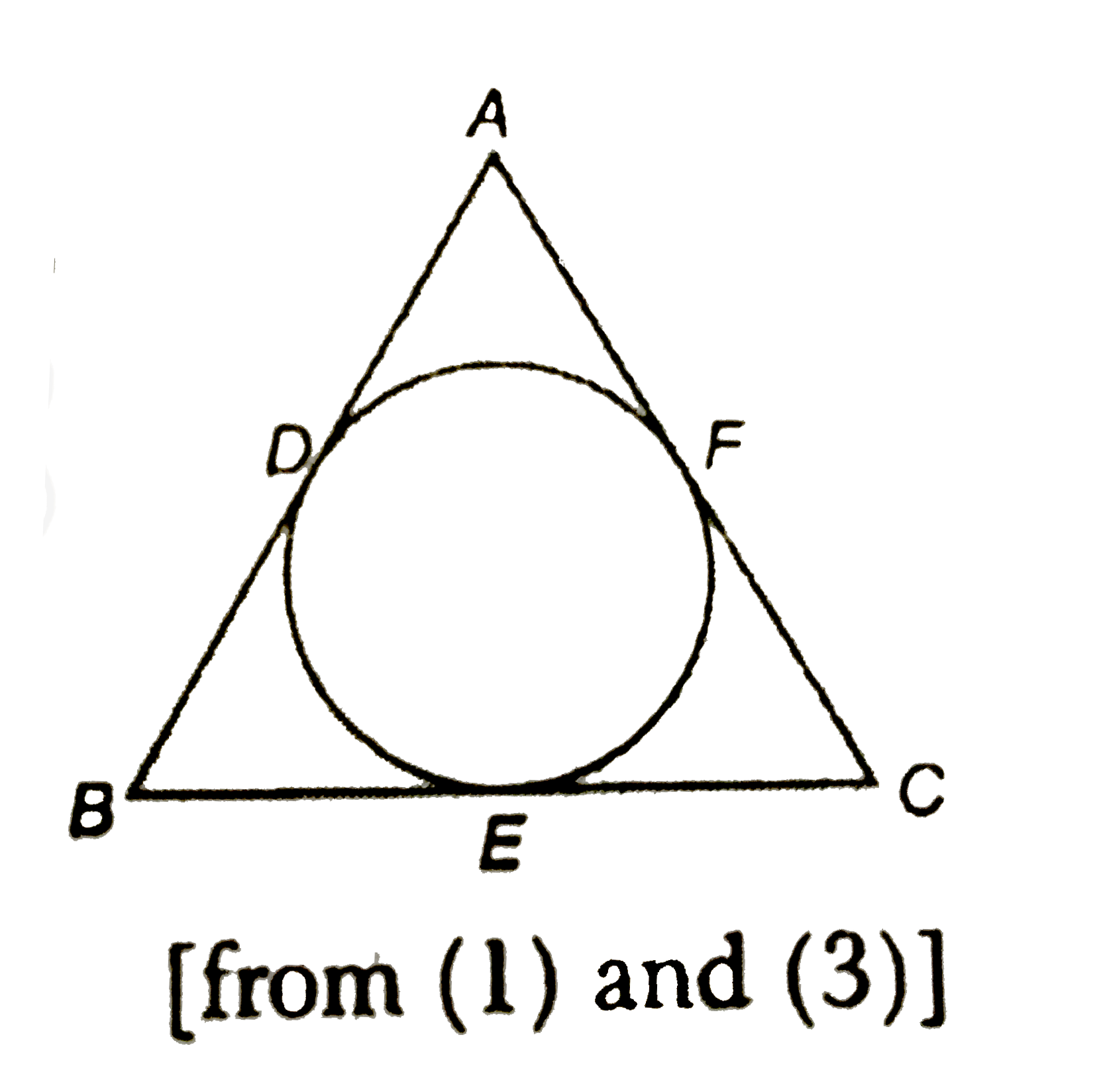
Video Solution
Text Solution
We know that, length of tangents from an external point are equal.
∴ AD=AF …(1)
DB=BE …(2)
EC=FC …(3)
Now, it is given that
AB=AC
⇒ AD+DB=AF+FC
implies" "cancel(AD)+DB=cancel(AD)+EC" "[from (1)and(3)]
⇒ DB=EC
∴ BE=EC [from (2), DB=BE] Hence Proved.
|
Topper's Solved these Questions
- CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Problems From NCERT/exemplar
11 Videos
- CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Exercise
25 Videos
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHANCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Revision Exercise Long Answer Question
5 Videos
- CO-ORDINATE GEOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Revision Exercise Short Answer Questions
9 Videos
Similar Questions
In the given figure, if AB=AC, prove that BE=EC.
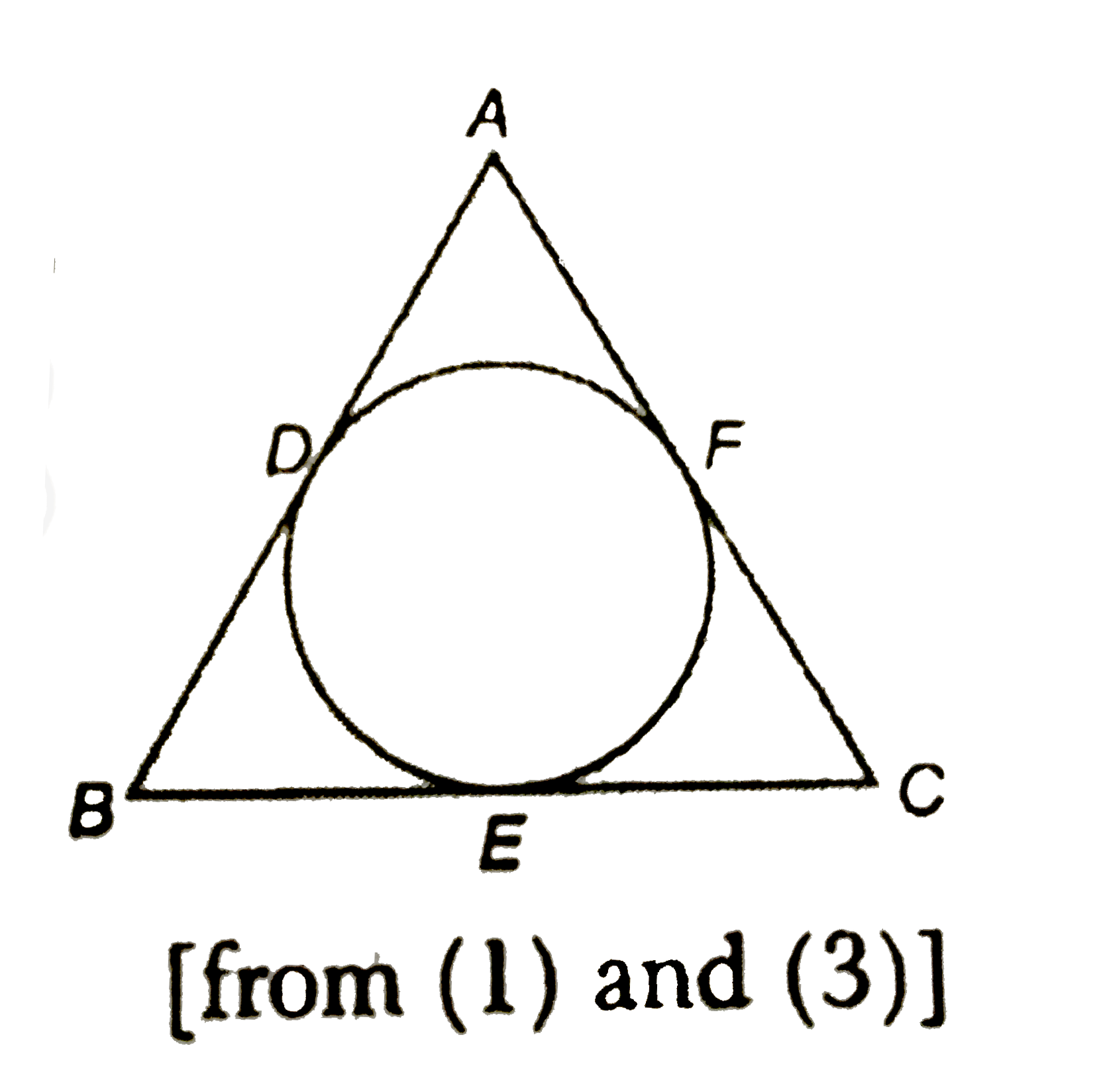
View SolutionIn the figure, if AB=AC, prove that: BE=EC
View SolutionIn the given figure, AB=AC. prove that :
View SolutionIn Fig , if AB = AC , prove that BE = EC .
View SolutionIn the given figure if AC=BD then prove that AB=CD
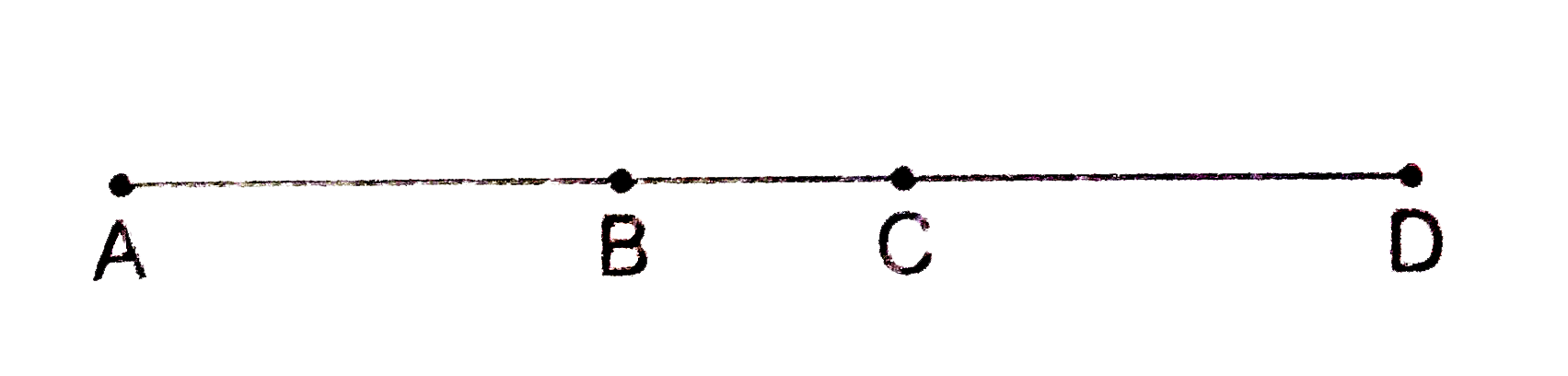
View SolutionIn the given figure, if AC = BD, then prove that AB = CD.

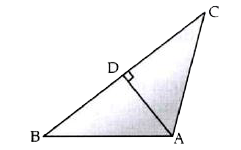
View SolutionIn the given figure if AD ⊥ BC prove that AB2+CD2=BD2+AC2
View SolutionIn the given figure of AD = CB then prove that AC = BD

View SolutionIn the following figure, if AB = CD, prove that AC = BD. State the axioms used in proving the result.
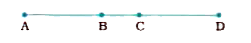
View SolutionIn the given, if AB = AC. prove the BE = EC.
View Solution
