In triangles ABC, the measures of angles a, b, and c, respectively, are in the ratio 2:3:4.What is the measure of angle b?
20
40
60
80
The correct Answer is:C
To solve the problem, we need to find the measure of angle B in triangle ABC, where the angles are in the ratio 2:3:4.
1. Understand the properties of triangles:
The sum of the angles in any triangle is always 180 degrees.
2. Set up the ratio:
Given the angles A, B, and C are in the ratio 2:3:4, we can express them in terms of a variable x:
- Let angle A = 2x
- Let angle B = 3x
- Let angle C = 4x
3. Write the equation for the sum of angles:
According to the property of triangles, we can write the equation:
A+B+C=180
Substituting the expressions for A, B, and C, we get:
2x+3x+4x=180
4. Combine like terms:
Combine the terms on the left side:
9x=180
5. Solve for x:
Divide both sides by 9 to find x:
x=1809=20
6. Find the measure of angle B:
Now that we have x, we can find angle B:
B=3x=3×20=60 degrees
Conclusion:
The measure of angle B is 60 degrees.
The sum of the angles in any triangle is always 180 degrees.
Given the angles A, B, and C are in the ratio 2:3:4, we can express them in terms of a variable x:
- Let angle A = 2x
- Let angle B = 3x
- Let angle C = 4x
According to the property of triangles, we can write the equation:
A+B+C=180
Substituting the expressions for A, B, and C, we get:
2x+3x+4x=180
Combine the terms on the left side:
9x=180
Divide both sides by 9 to find x:
x=1809=20
Now that we have x, we can find angle B:
B=3x=3×20=60 degrees
The measure of angle B is 60 degrees.
Topper's Solved these Questions
- PROBLEM SETSBOOK - PRINCETONCHAPTER - PROBLEM SETSEXERCISE - PROBLEM SET 18: MORE MIXED BAG
11 Videos
- PROBLEM SETSBOOK - PRINCETONCHAPTER - PROBLEM SETSEXERCISE - PROBLEM SET 16: MORE GRID-INS
11 Videos
- PRACTICE TEST 4BOOK - PRINCETONCHAPTER - PRACTICE TEST 4EXERCISE - Math Test-Calculator
38 Videos
- SAT MATH: THE BIG PICTUREBOOK - PRINCETONCHAPTER - SAT MATH: THE BIG PICTUREEXERCISE - Example
4 Videos
Similar Questions
Knowledge Check
In the figure, the angles A, B, C of the triangle are in the ratio 5 : 12 : 13. What is the measure of angle A?
 A15B27C30D34
A15B27C30D34The angles of trinalge are in the ratio 2:3:4. What is the degree measures of the largest angle?
A40B80C90D120What is the angle measure of 4 radians ?
A114.591∘B141.372∘C229.183∘D282.743∘
In a quadrilateral ABCD , the angles A, B, C and D are in the ratio 1:2:3:4. Find the measure of each angles of the quadrilateral.
View SolutionIn a quadrilateral ABCD, the angles A, B, C and D are in the ratio 1:2:3:4 . Find the measure of each angle of the quadrilateral.
View SolutionIf the angle of a triangle are in the ratio 2:1:3, then find the measure of smallest angle.
View SolutionIn a quadrilateral ABCD, the angles A, B, C and D are in the ratio 1:2:4:5. Find the measure of each angle of the quadrilateral.
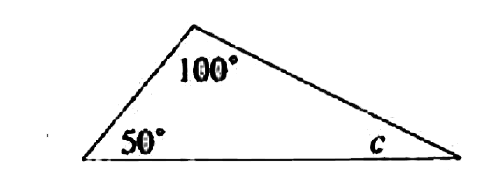
View SolutionIn this triangle, what is the degree measure of angle c ?
View Solution
