It is true to say that the mean, mode and median of grouped data always be different? Justify your answer.
To determine whether the mean, mode, and median of grouped data are always different, we need to analyze the definitions and characteristics of these statistical measures.
1. Understanding Mean:
- The mean (average) is calculated by summing all the observations and dividing by the number of observations.
- Formula: Mean=Sum of all observationsNumber of observations
2. Understanding Mode:
- The mode is the value that appears most frequently in the data set.
- If a number occurs more times than any other, it is the mode.
3. Understanding Median:
- The median is the middle value when the data is arranged in ascending order.
- For an odd number of observations, the median is the value at position n+12 where n is the number of observations.
- For an even number of observations, it is the average of the two middle numbers.
4. Example with Data:
- Consider the data set: 3, 3, 3, 3, 3 (5 observations).
- Calculate Mean:
Mean=3+3+3+3+35=155=3
- Calculate Mode:
- The number 3 appears most frequently (5 times), so the mode is 3.
- Calculate Median:
- Since there are 5 observations (odd), the median is the 3rd observation in the ordered list, which is 3.
5. Conclusion from Example:
- In this example, we find that the mean, mode, and median are all equal to 3.
- This shows that it is not always true that these measures are different; they can be the same depending on the data set.
6. General Statement:
- The mean, mode, and median can be the same or different based on the nature of the data.
- For certain distributions (like uniform or symmetric distributions), they can be equal, while in skewed distributions, they can differ.
Final Justification:
- Therefore, it is incorrect to say that the mean, mode, and median of grouped data are always different. Their relationship depends on the specific data set being analyzed.
- The mean (average) is calculated by summing all the observations and dividing by the number of observations.
- Formula: Mean=Sum of all observationsNumber of observations
- The mode is the value that appears most frequently in the data set.
- If a number occurs more times than any other, it is the mode.
- The median is the middle value when the data is arranged in ascending order.
- For an odd number of observations, the median is the value at position n+12 where n is the number of observations.
- For an even number of observations, it is the average of the two middle numbers.
- Consider the data set: 3, 3, 3, 3, 3 (5 observations).
- Calculate Mean:
Mean=3+3+3+3+35=155=3
- Calculate Mode:
- The number 3 appears most frequently (5 times), so the mode is 3.
- Calculate Median:
- Since there are 5 observations (odd), the median is the 3rd observation in the ordered list, which is 3.
- In this example, we find that the mean, mode, and median are all equal to 3.
- This shows that it is not always true that these measures are different; they can be the same depending on the data set.
- The mean, mode, and median can be the same or different based on the nature of the data.
- For certain distributions (like uniform or symmetric distributions), they can be equal, while in skewed distributions, they can differ.
- Therefore, it is incorrect to say that the mean, mode, and median of grouped data are always different. Their relationship depends on the specific data set being analyzed.
Topper's Solved these Questions
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - SHORT ANSWERS QUESTIONS
42 Videos
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ASWERS QUESTIONS
14 Videos
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ASWERS QUESTIONS
14 Videos
- REAL NUMBERSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - REAL NUMBERSEXERCISE - LONG ANSWER TYPE QUESTIONS
5 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - LONG ANSWER TYPE QUESTIONS
20 Videos
Similar Questions
Will the median class and modal class of grouped data always by different? Justify your answer.
View SolutionCan Q and S, both be metals ? Justify your answer.
View SolutionWould you expect the first ionisation enthalpies for two isotopes of the same element to be the same or different? Justify your answer.
View SolutionFind the median and mode of the data:
View SolutionWrite the empirical relation between mean, mode and median.
View SolutionFind the mean, median and mode of the following data
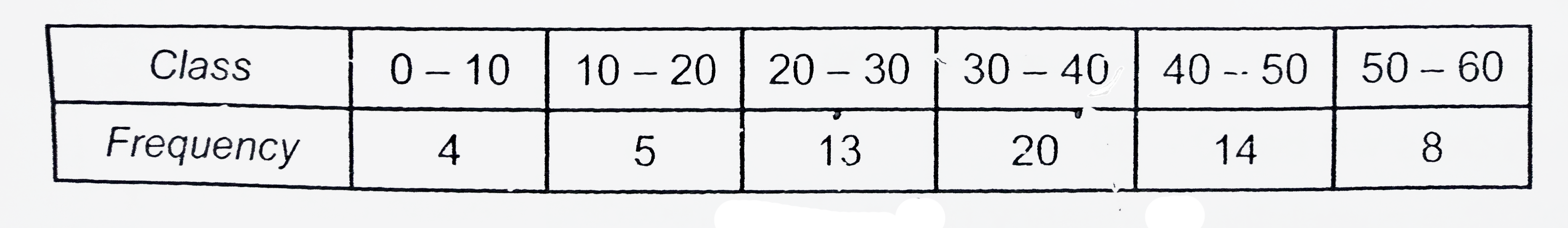
View SolutionIs it correct to say that any two right angles are congruent? Give reasons to justify your answer.
View SolutionWhat happens to the image distance in the normal human eye when we decrease the
distance of an object, say 10 m to 1 m? Justify your answer.
View SolutionFind the mean, median and mode of the following data :
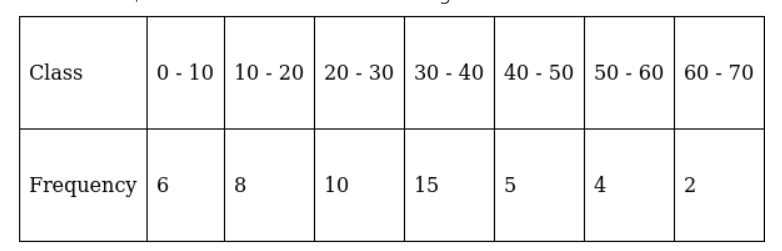
View SolutionGive an example of an ecological pyramid which is always upright. Justify your answer.
View Solution
