Let A={x:−1≤x≤1}=B for each of the following functions from A to B. Find whether it is surjective, injective or bijective
(i) f(x)=x2
(ii) g(x)=|x|
The correct Answer is:B, C
A={x:−1≤x≤}
B={x:−1≤x≤}
(i) f(x)=x2
Let x1,x2∈A
∴f(x1)=f(x2)⇒x12=x22
⇒x1=x2
∴ f is one-one function
Now, let y=x2⇒x=2y
⇒−1≤y≤1
⇒−2≤2y≤2⇒−2≤x≤2
Let x∈[−1,1]
∴ There are some value of y for which x does not exist. So, f not onto.
(ii) g(x) = |x|
For x=-1, g(-1) = 1
and for x = 1, g(1) = 1
∴ f is not one-one function
Let y = |x|, then y≥0
∴ f is not onto.
(iii) h(x) = x|x| = {x2x≥0−x2x<0
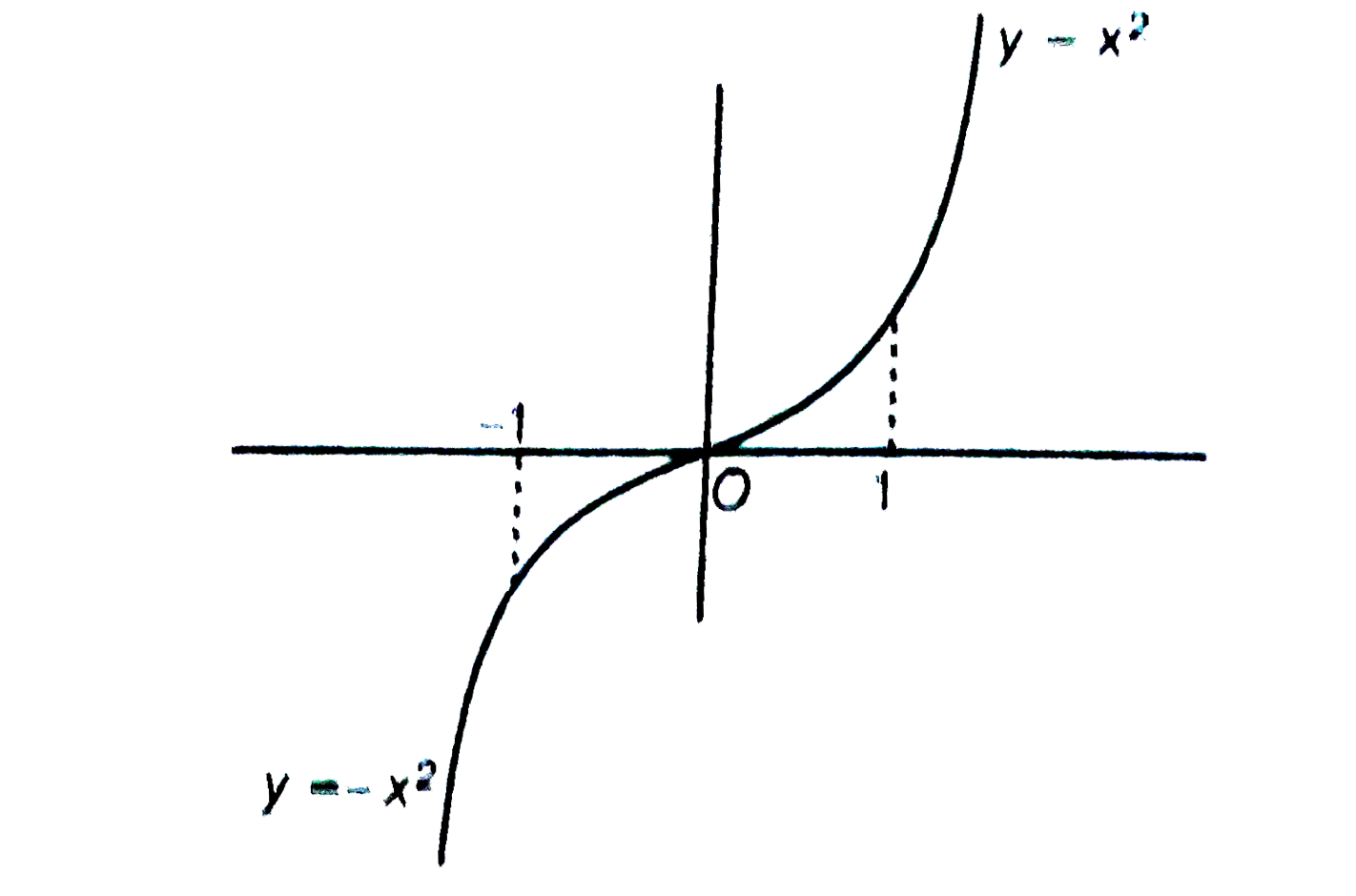
From figure, it is clear tat h is one-one and onto i.e., bijective.
(iv) k(x)=x2
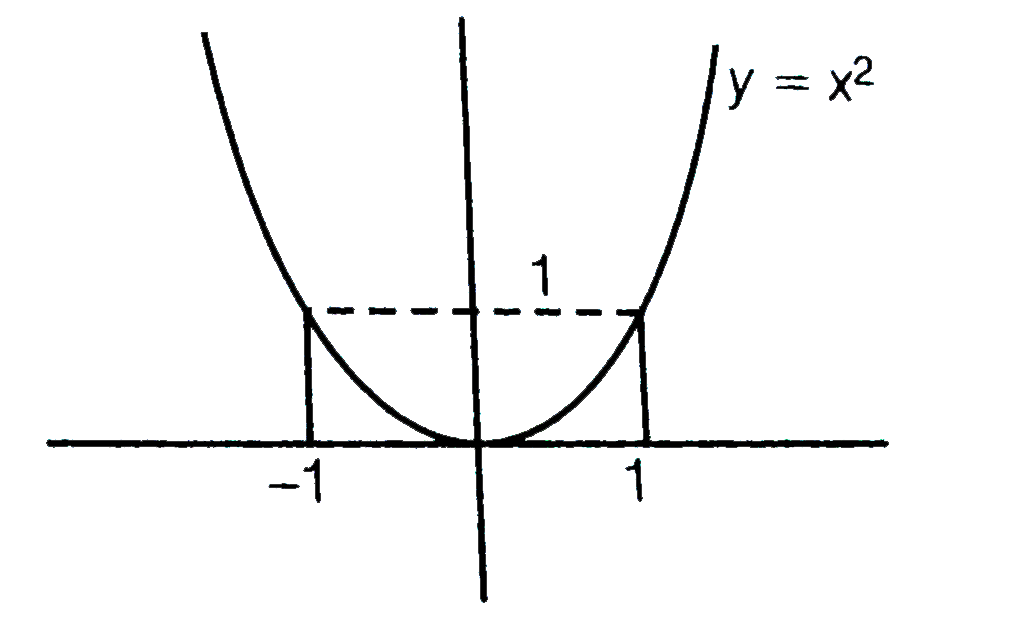
k(1) = 1
and k(-1) = 1
So, k is many-one function.
From figure, y∈(0,1)
∴ y is not onto function.
(v) y=l(x)=sinπx
for x = 1, l(1)=sinπ=0
for x = - 1, l(-1) = sin (−π) = 0
∴l is not one-one.
Now, −1≤x≤1
⇒−π≤πx≤π
⇒−1≤sinπx≤1
∴ y is onto function.
Hence, l is surjective function.
Topper's Solved these Questions
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHS ENGLISHCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHS ENGLISHCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Statement I And Ii Type Questions)
4 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
- THE STRAIGHT LINESBOOK - ARIHANT MATHS ENGLISHCHAPTER - THE STRAIGHT LINESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
17 Videos
Similar Questions
Let A=[−1, 1] . Then, discuss whether the following functions from A to itself are one-one, onto or bijective: f(x)=x2 (ii) g(x)=|x| (iii) h(x)=x2
View SolutionLet f:A→A where A={x:−1≤x≤1}. Find whether the following function are bijective
x|x|
View SolutionLet f:A→A where A={x:−1≤x≤1}. Find whether the following function are bijective
x|x|
View SolutionLet f:A→A where A={x:−1≤x≤1}. Find whether the following function are bijective
x4
View SolutionLet f:A→A where A={x:−1≤x≤1}. Find whether the following function are bijective
x4
View SolutionLet A=[−1,1]. Then, discuss whether the following functions from A to itself are one-one onto or bijective: f(x)=x2 (ii) g(x)=|x| (iii) h(x)=x2
View SolutionLet A=[−1, 1] . Then, discuss whether the following functions from A to itself are one-one, onto or bijective: f(x)=x2 (ii) g(x)=|x| (iii) h(x)=x2
View SolutionLet A=[−1,1]. Then, discuss whether the following functions from A to itself are one-one onto or bijective: f(x)=x2 (ii) g(x)=|x| (iii) h(x)=x2
View SolutionLet A=[−1,1]. Then, discuss whether the following functions from A to itself are one-one onto or bijective: f(x)=x2 (ii) g(x)=|x| (iii) h(x)=x2
View SolutionLet A=[−1,1]. Then, discuss whether the following functions from A to itself are one-one onto or bijective: f(x)=x2 (ii) g(x)=|x| (iii) h(x)=x2
View Solution
