let A(x1,0) and B(x2,0) be the foci of the hyperbola x29−y216=1 suppose parabola having vertex at origin and focus at B intersect the hyperbola at P in first quadrant and at point Q in fourth quadrant.Solution in Bengali
(−910,0)
(23,0)
(910,0)
(23,√6)
a=3,e=13
∴F1≡(−1,0)andF2≡(1,0)
SO, equation of parbola is y2=4x
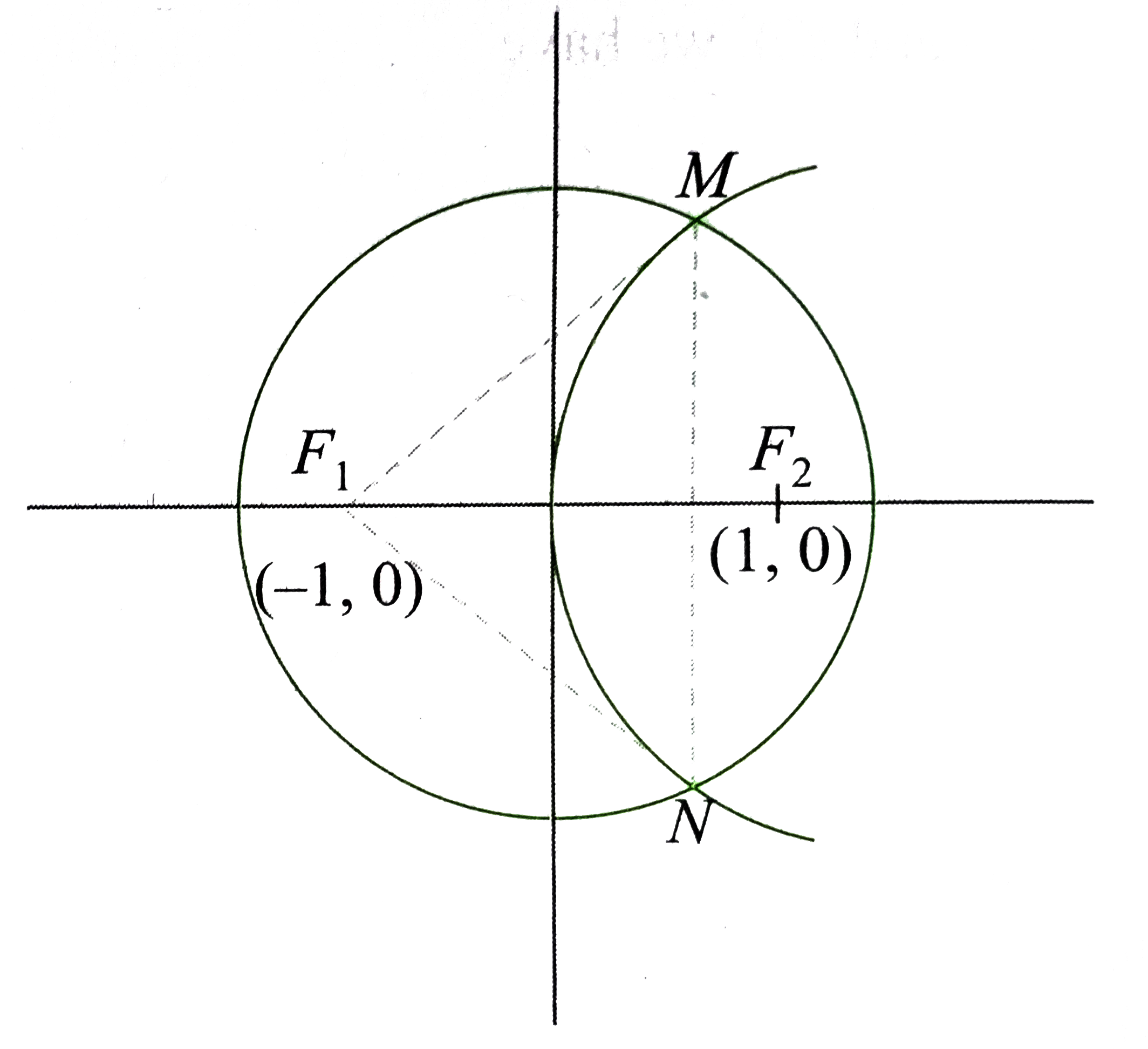
Solving ellipse and parabola, we get points of intersection as
M(32,√6)1andM(32,√6)
Orthocentre of ΔF1MN lioes onb x-axis.
Slope of
F1N=−√6−032+1=−2√65
Putting y=0 or orhtocentre, we get y=−910
So, orthocenter is (−910,0)
Similar Questions
Let a and b be positive real numbers such that a>1andb<a . Let be a point in the first quadrant that lies on the hyperbola x2a2−y2b2=1 . Suppose the tangent to the hyperbola at P passes through the oint (1,0) and suppose the normal to the hyperbola at P cuts off equal intercepts on the coordinate axes . Let Δ denote the area of the triangle formed by the tangent at P , the normal at P and the x - axis . If a denotes the eccentricity of the hyperbola , then which of the following statements is/are TRUE ?
10:00View SolutionFrom the point (2, 2) tangent are drawn to the hyperbola x216−y29=1. Then the point of contact lies in the first quadrant (b) second quadrant third quadrant (d) fourth quadrant
04:32View SolutionFrom the point (2, 2) tangent are drawn to the hyperbola x216−y29=1. Then the point of contact lies in the (a) first quadrant (b) second quadrant (c) third quadrant (d) fourth quadrant
02:41View Solutionlet A(x1,0) and B(x2,0) be the foci of the hyperbola x29−y216=1 suppose parabola having vertex at origin and focus at B intersect the hyperbola at P in first quadrant and at point Q in fourth quadrant.
02:44View Solutionमाना वृत्त x2+y2=9 तथा परवलय y2=8x है। यह प्रथम तथा चतुर्थ चतुर्थाश में क्रमशः P तथा Q पर प्रतिच्छेद करते है। वृत्त की P तथा Q पर स्पर्श रेखाएं x-अक्ष को R पर प्रतिच्छेद करती है तथा परवलय की P तथा Q पर स्पर्श रेखा x-अक्ष को S पर प्रतिच्छेद करती है।
त्रिभुज PRS के परिवृत्त की त्रिज्या है-15:38View Solutionमाना वृत्त x2+y2=9 तथा परवलय y2=8x है। यह प्रथम तथा चतुर्थ चतुर्थाश में क्रमशः P तथा Q पर प्रतिच्छेद करते है। वृत्त की P तथा Q पर स्पर्श रेखाएं x-अक्ष को R पर प्रतिच्छेद करती है तथा परवलय की P तथा Q पर स्पर्श रेखा x-अक्ष को S पर प्रतिच्छेद करती है।
त्रिभुज PQR के अन्तःवृत्त की त्रिज्या है-08:02View SolutionLet F1(x1,0) and F2(x2,0), for x1<0 andx2>0, be the foci of the ellipse x29+y28=1. Suppose a parabola having vertex at the origin and focus at F2 intersects the ellipse at point M in the first quadrant and at point N in the fourth quadrant.
If the tangents to the ellipse at M and N meet at R and the normal to the parabola at M meets the x-axis at Q, then the ratio of the area of the triangle MQR to area of the quadrilateral MF1NF2 is :13:27View SolutionFrom the point (2, 2) tangent are drawn to the hyperbola x216−y29=1. Then the point of contact lies in the (a)first quadrant (b) second quadrant (c)third quadrant (d) fourth quadrant
03:53View Solution

