Let f (x) be a polynomial function of degree 3 where a<b<candf(a)=f(b)=f(c). If the graph of f (x) is as shown, which of the following statements are INCORRECT ? (Where c>|a|)
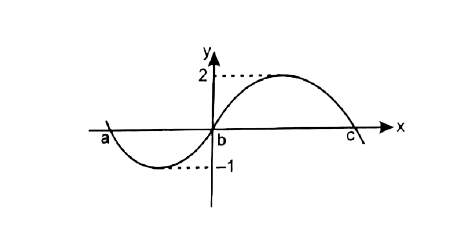
A
∫caf(x)dx=∫cbf(x)dx+∫bcf(x)dx
B
∫caf(x)dx<a
C
∫baf(x)dx<∫bcf(x)dx
D
1b−a∫baf(x)dx>1c−b∫cbf(x)dx
Text Solution
The correct Answer is:B, C, D
|
Topper's Solved these Questions
- AREA UNDER CURVESBOOK - VK JAISWALCHAPTER - AREA UNDER CURVESEXERCISE - EXERCISE (COMPREHENSION TYPE PROBLEMS)
6 Videos
- AREA UNDER CURVESBOOK - VK JAISWALCHAPTER - AREA UNDER CURVESEXERCISE - EXERCISE (MATCHING TYPE PROBLEMS)
1 Videos
- AREA UNDER CURVESBOOK - VK JAISWALCHAPTER - AREA UNDER CURVESEXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
8 Videos
- APPLICATION OF DERIVATIVES BOOK - VK JAISWALCHAPTER - APPLICATION OF DERIVATIVES EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
22 Videos
- BIONMIAL THEOREMBOOK - VK JAISWALCHAPTER - BIONMIAL THEOREMEXERCISE - Exercise-4 : Subjective Type Problems
15 Videos
Similar Questions
Knowledge Check
Let f(x) be a polynomial function of second degree such that f(1) = f(-1). If a, b, c are in A.P., then f'(a), f'(b) and f'(c) are in :
AA.P.BG.P.CH.P.DA.G.P.Let f(x)=(x−a)(x−b)(x−c) be a ral vlued function where a<bc(a,b,c∈R) such that f''(α)=0. Then if α∈(c1,c2), which one of the following is correct ?
Aα<c1<bandb<c2<cBα<c1,c2<bCb<c1,c2<cDNone of theseIf f(c) < 0 and f'(c) > 0 , then at x = c , f (x) is -
AmaximumBminimumCneither maximum nor minimumDeither maximum or minimum
If f(x) is a polynomial of degree n(>2) and f(x)=f(α−x),
(where α is a fixed real number ), then the degree of f'(x) is
View SolutionIf f(x) is a polynomial of degree n(>2) and f(x)=f(α−x),
(where α is a fixed real number ), then the degree of f'(x) is
View SolutionIf a<b<c∈R and f(a+b+c−x)=f(x) for all, x then ∫bax(f(x)+f(x+c))dxa+b is:
View SolutionIf a<b<c∈R and f(a+b+c−x)=f(x) for all, x then ∫bax(f(x)+f(x+c))dxa+b is:
View SolutionIf a<b<c∈R and f(a+b+c−x)=f(x) for all, x then ∫bax(f(x)+f(x+c))dxa+b is:
View Solution
