Let f={(2,4),(5,6),(8,−1),(10,−3)
and g={(2,5),(7,1),(8,4),(10,13),(11,5)}
be two real functions. Then, match the following .
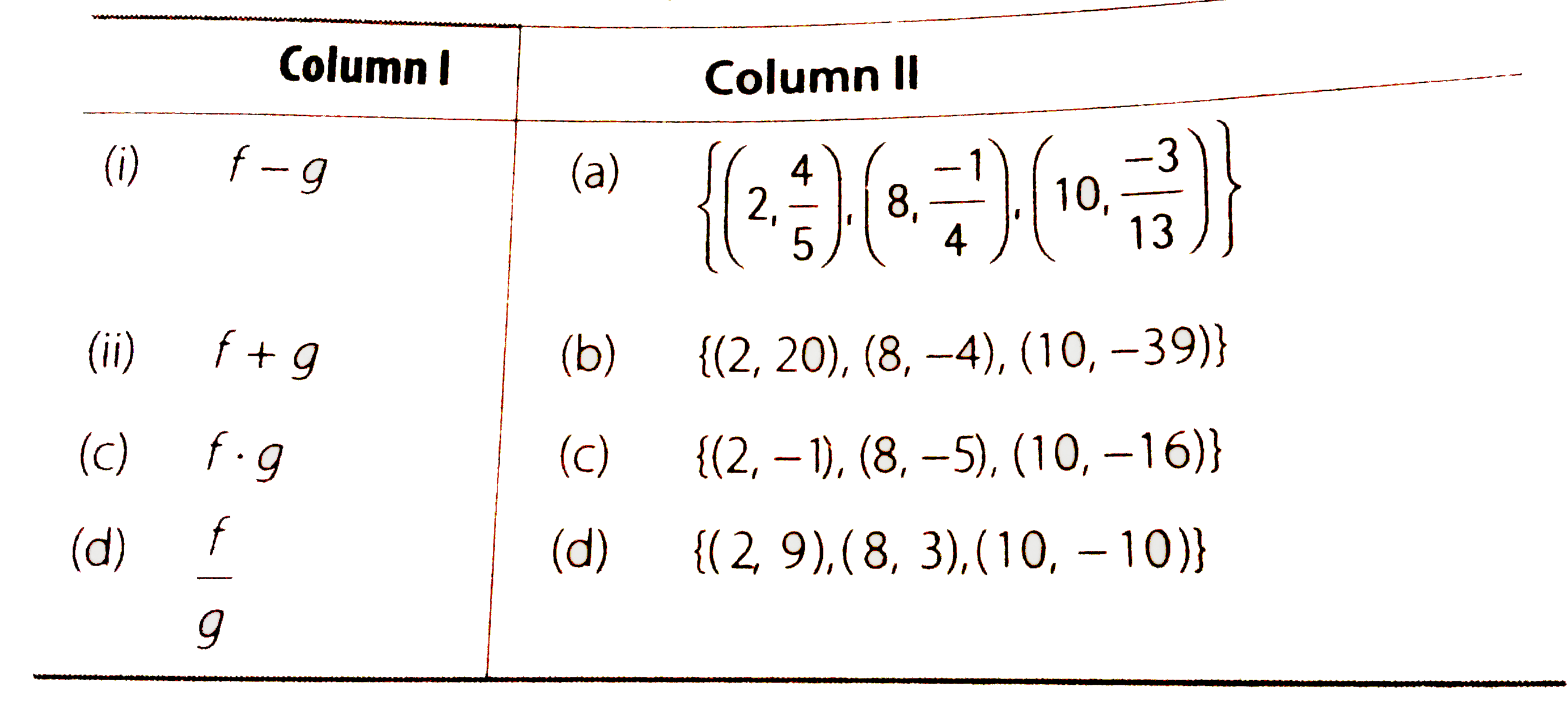
Let f={(2,4),(5,6),(8,-1),(10,-3) and g={(2,5),(7,1),(8,4),(10,13),(11,5)} be two real functions. Then, match the following . The domain of f-g,f+g,f*g,(f)/(g) is domain of f nn domain of g. Then, find their images.
The domain of f−g,f+g,f⋅g,fg is domain of f ∩ domain of g. Then, find their images.

Text Solution
Verified by Experts

We have
f={(2,4),(5,6),(8,1),(10,−3)
and g={(2,5),(7,1),(8,4),(10,13),(11,5)}
So, f−g,f+g,f⋅g,fg are defined in the domain (domain of f∩ domain of g)
i.e.,{2,5,8,10}∩{2,7,8,10,11}⇒{2,8,10}
(i) (f−g)(2)=f(2)−g(2)=4−5=−1
(f−g)(8)=f(8)−g(8)=−1−4=−5
(f−g)(10)=f(10)−g(10)=−3−13=−16
f−g={(2,−1),(8,−5),(10,−16)}
(ii) (f+g)(2)=f(2)=f(2)+g(2)=4+5=9
(f+g)(8)=f(8)+g(8)=−1+4=3
(f+g)(10)=f(10)+g(10)=−3+13=10
∴f−g={(2,9).(8,3),(10,10)}
(iii) (f−g)(2)=f(2)⋅g(2)=4×5=20
(f⋅g)(8)=f(8)⋅(8)=−1×4=−4
(f⋅g)(10)=f(10)⋅g(10)=−3×13=−39
∴fg={(2,20),(8,−4),(10,−39)}
(iv) (fg)(2)=f(2)g(2)=45
(fg)(8)=f(8)g(8)=−14
(fg)(10)=f(10)g(10)=−313
∴fg={(2,4d),(8,−14),(10,−313)}
Hence , the correct matches are (i)→(c),(ii)→(d),(iii)→(b),(iv)→(a).

Updated on:21/07/2023
Doubtnut Promotions Banner
|
Class 11MATHSRELATIONS AND FUNCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • If f={(1,2),(2,3),(3,5),(5,8)}andg={(2,1),(3,2),(5,3),(8,5)} then f∘g=

    A{(1,2),(2,3),(3,5),(5,8),(2,1),(3,2),(5,3),(8,5)}
    B{(2,2),(3,3),(5,5),(8,8,)}
    C{(1,1),(2,2),(3,3),(5,5)}
    Dnone of these.

  • Let f(x) be a function whose domain is [−5,7] and g(x)=|2x+5|. Then, the domain of fog (x) is .

    A[−5,1]
    B[−4,0]
    C[−6,1]
    Dnone of these

  • Let f be a function with domain [-3, 5] and let g (x) = |3x+4|, Then the domain of (fog) (x) is

    A(−3,13)
    B[−3,13]
    C[−3,13)
    DNone of these