Let f:R→[1,∞),f(x)=x2−4x+5. Then find the largest possible intervals for which f−1(x) is defined and find corresponding f−1(x).
Video Solution
Text Solution
We have f:R→[1,∞),f(x)=x2−4x+5
or f(x)=(x−2)2+1.
Graph of the function is as shown in the following figure.
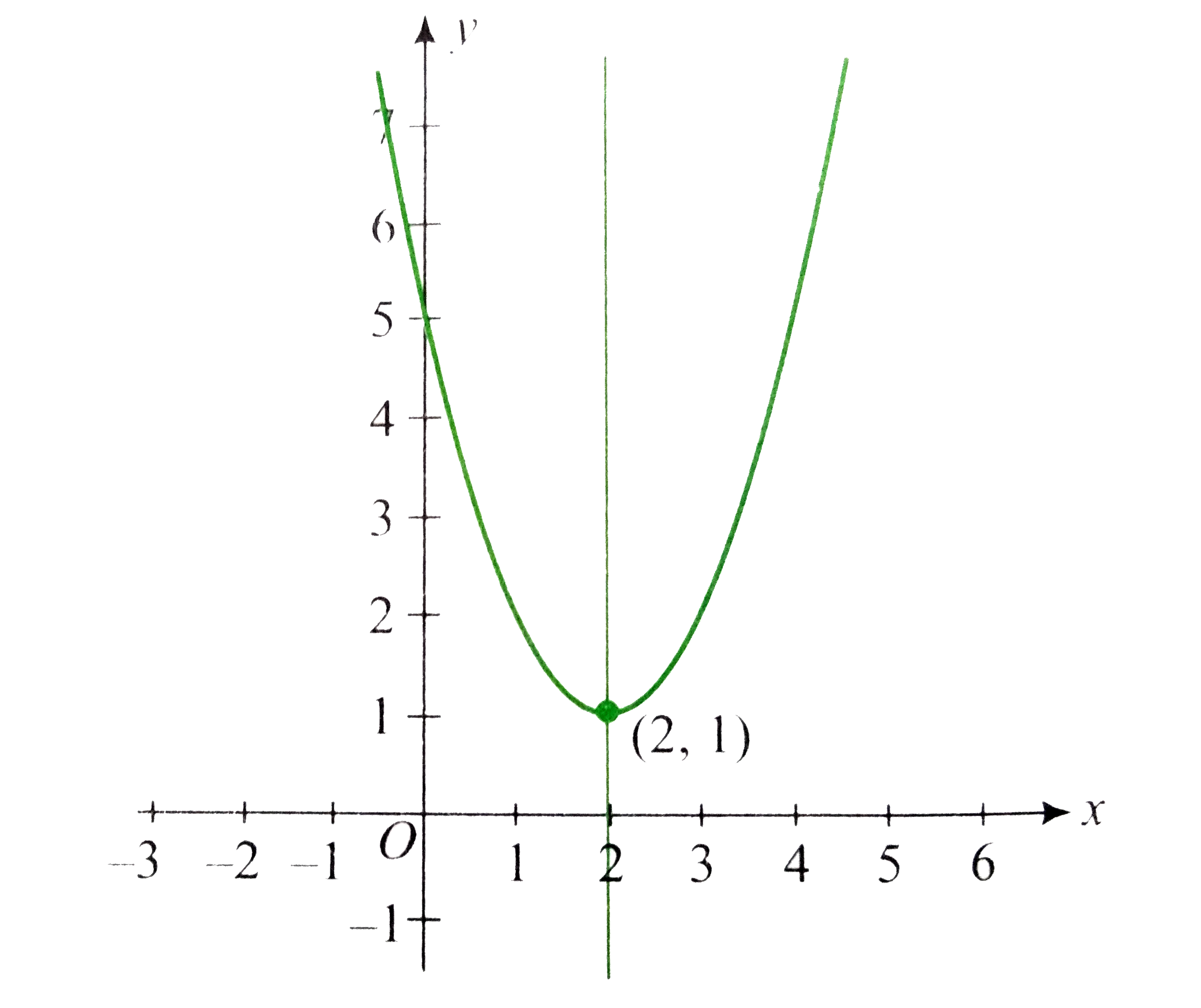
Clearly f(x) is many-one function.
However, in the interval (−∞,2]or[2,∞),f(x) is one-one.
For f:(−∞,2]→[1,∞),y=(x−2)2+1
∴(x−2)2=y−1
⇒x−2=√y−1
⇒x=2−√y−1
Thus f−1:[1,∞)→(−∞,2],f−1(x)=2−√x−1
For f:[2,∞)→[1,∞),y=(x−2)2+1
⇒x=2+√y−1
Thus f−1:[1,∞)→[2,∞),f−1(x)=√x−1+2
|
Topper's Solved these Questions
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.1
15 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.2
5 Videos
- Quadratic Equations, Inequalities, Modulus and LogarithmsBOOK - CENGAGECHAPTER - Quadratic Equations, Inequalities, Modulus and LogarithmsEXERCISE - Question Bank
31 Videos
- SCALER TRIPLE PRODUCTS BOOK - CENGAGECHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.3
11 Videos
Similar Questions
Knowledge Check
Let f(x)=x3+6x2+ax+2,if(−3,−1) is the largest possible interval for which f(x) is decreasing function, then a=
A3B9C−2D1Let f:(2,∞)→X be defined by f(x)=4x−x2. Then f is invertible, if X=
A[2,∞]B(−∞,2]C(−∞,4]D[4,∞)Let f:R→R be defined by f(x)=3x-4. Then, f−1(x) is
Ax+43Bx3−4C3x+4Dnone of these
5.Let f:R→R be defined by f(x)=x2−1 .Then find fof(2)
View SolutionLet f:R→R,f(x)=x3+x−1. Find the solution of f(x)=f−1x
View SolutionLet f:(−∞,−1)→R−{1} is defined as f(x)=xx+1 Find f−1(x)
View SolutionIf f(x)=x2+1 defined on R→R, find value of x for which f(x)=−5.
View SolutionIf f:[1,∞)→[2,∞) is defined by f(x)=x+1x, find f−1(x)
View Solution
