Let f(x)=⎧⎪ ⎪⎨⎪ ⎪⎩tan2{x}x2−[x]2forx>01√{x}cot{x}forx<0 where [x] is the step up function and {x} is the fractional part function of x thenSolution in Punjabi
The correct Answer is:A
Step by step video & image solution for Let f(x)={((tan^(2){x})/(x^(2)-[x]^(2)),"for"xgt0),(1/(sqrt({x}cot{x})),"for"xlt0):} where [x] is the step up function and {x} is the fractional part function of x then by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LIMITSBOOK - ARIHANT MATHSCHAPTER - LIMITSEXERCISE - SOLVED EXAMPLES
1 Videos
- LIMITSBOOK - ARIHANT MATHSCHAPTER - LIMITSEXERCISE - Exercise (Single Option Correct Type Questions)
38 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - ARIHANT MATHSCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
8 Videos
- LOGARITHM AND THEIR PROPERTIESBOOK - ARIHANT MATHSCHAPTER - LOGARITHM AND THEIR PROPERTIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
4 Videos
Similar Questions
To evaluate limx→a[f(x)], we must analyse the f(x) in right hand neighbourhood as well as in left hand neighbourhood of x=a. E.g. In case of continuous function, we may come across followign cases.
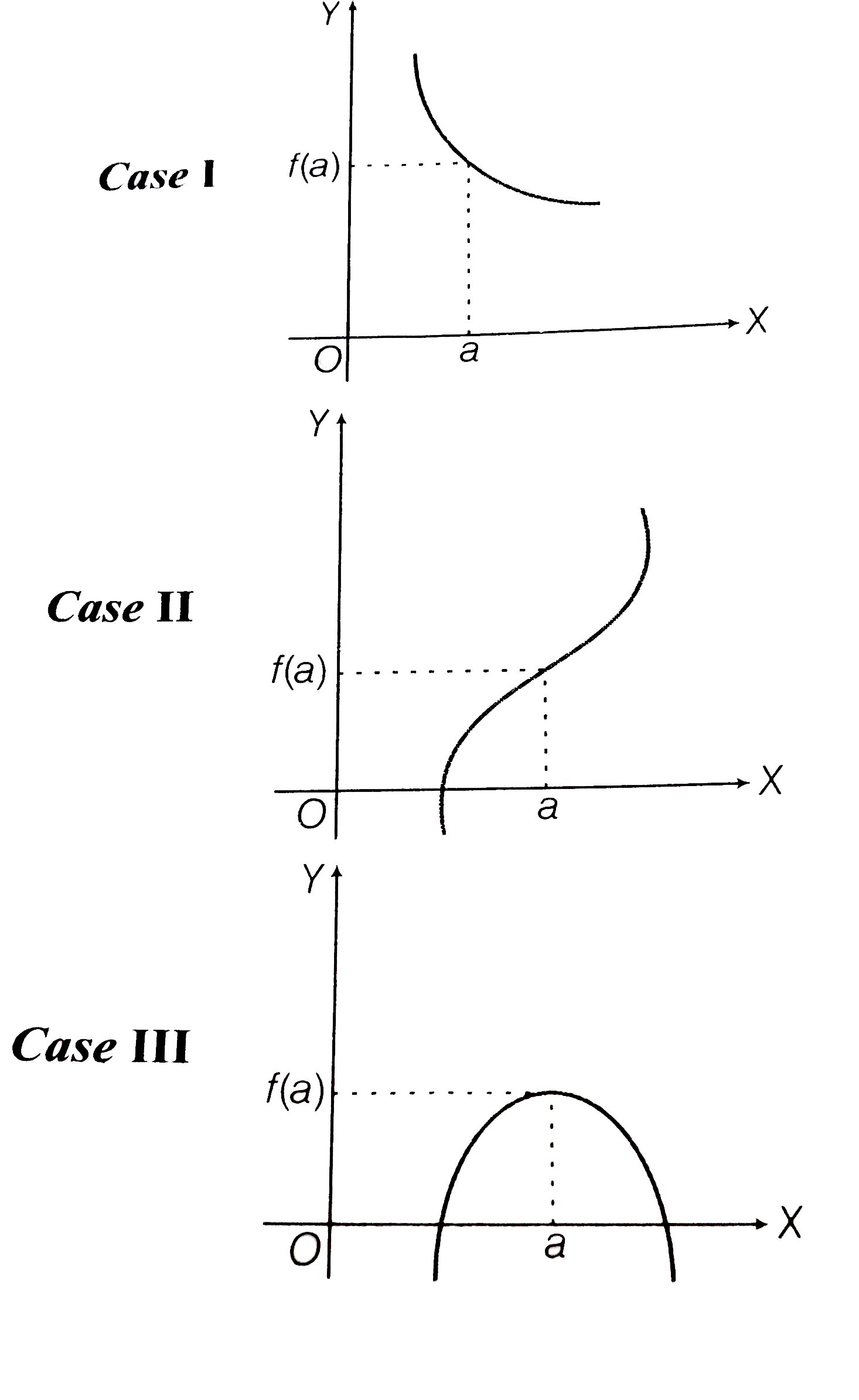
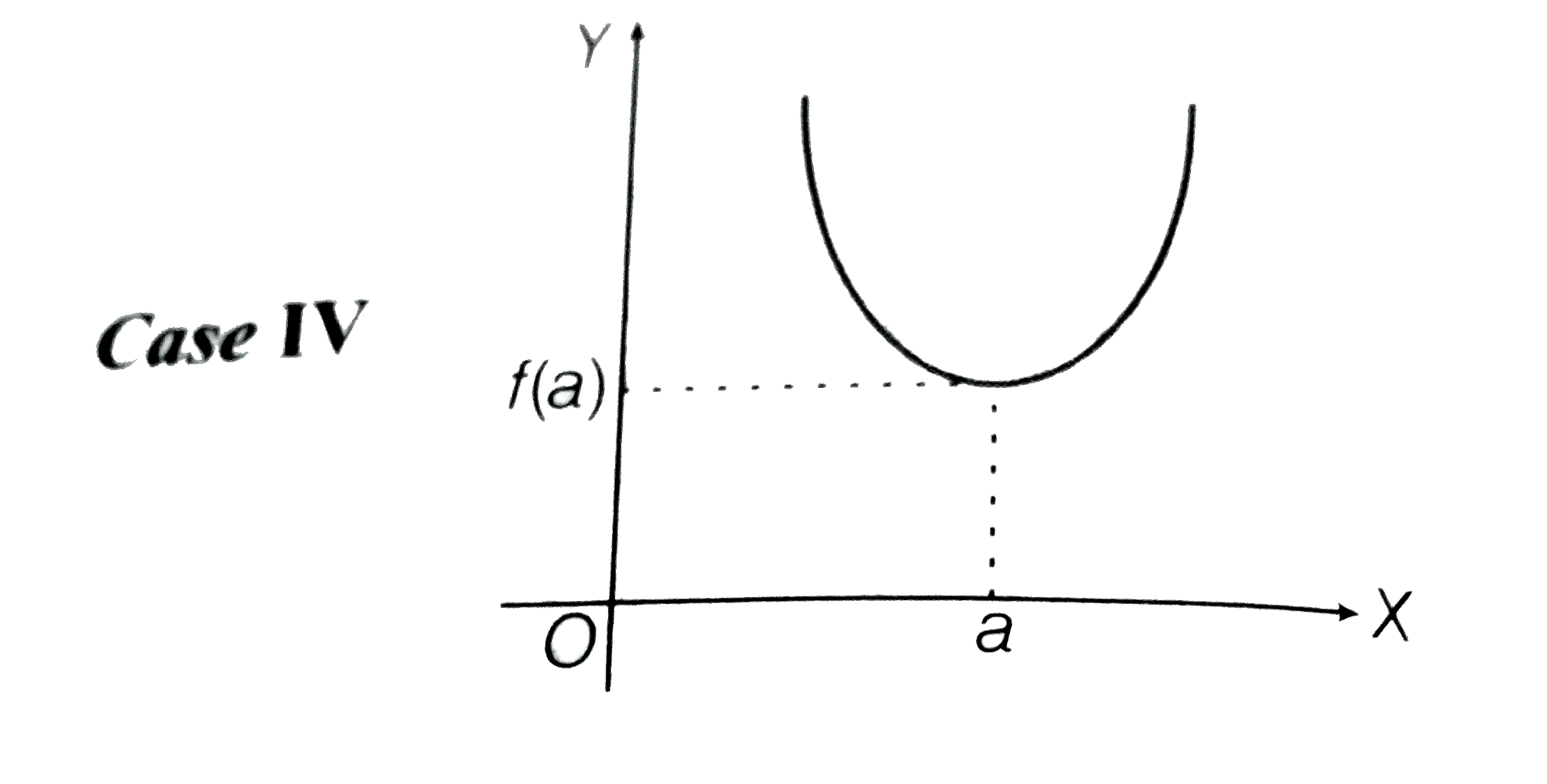
Iff(a) is an integer, the limit will exist in Case III and Case IV but not in Case I and Case II. If f(a) is not an integer, the limit exists in all the cases. ltbgt If f'(1)=−3 and limx→1[f(x)−12] does not exist, (where [.] denotes the greatest integer function), then
View Solutionlimx→0sin(πcos2(tan(sinx)))x2 is equal to
View SolutionIf α=3sin−1(611andβ=3cos−1(49), where the inverse trigonometric functions take only the principal values, then the correct options (s) is (are)
View SolutionIn Which quadrant Point (−2,−3) will be lie.
View SolutionDiscuss the continuity of f(x)={x{x}+1,0≤x<1and2−{x},1≤x<2 where {x} denotes the fractional part function.
View SolutionDiscuss the continuity of f(x)=[(x{x}+1,0<=x<=1),(2-{x}),1<=x<=2)] where {x} denotes the fractional part function.
View SolutionLet f (x)= tan/x, then the value of lim_(x->oo) ([f(x)]+x^2)^(1/({f(x)})) is equal to (where [.] , {.} denotes greatest integer function and fractional part functions respectively) -
View SolutionConsider the function f(x)=[(x)/([x]) if 1<=x<=2 and if x=2 and sqrt(6-x) if 2
View SolutionIf f(x)=[x^(2)]+sqrt({x}^(2)), where [] and {.} denote the greatest integer and fractional part functions respectively,then
View SolutionConsider the function f(x)=(cos^(-1)(1-{x}))/(sqrt(2){x}); where {.} denotes the fractional part function,then
View SolutionFind the range of each of the following functions: (where {.} and [.] represents fractional part and greatest integer part functions respectively)
f(x)=sinx√1+tan2x+cosx√1+cot2x
View SolutionLet f(x)=⎧⎪ ⎪⎨⎪ ⎪⎩tan2[x]x2−[x]21√|x|cot|x|forx>0forx=0forx<0where[x] and {x} denote respectively the greatest integer less than equal to x and the fractional part of x, then
View SolutionFor the function f(x)={{:(x+2", "xgt0),(x-2", "xlt0):}
View SolutionIs the function f(x)={{x}x≥0{-x}x<0 (where {.} denotes the fractional part of x is even?
View SolutionShow that the function f(x) defined by :
f(x)=⎧⎪ ⎪ ⎪⎨⎪ ⎪ ⎪⎩sinxx+cosx, x>02, x=04(1−√1−x)x, x<0
is continuous at x = 0.
View SolutionLet g be a function defined by g(x)=√{x}+√1−{x} .where {.} is fractional part function, then g is
View Solution
