Let f(x)=|x−x1|+|x−x2| where x1 and x2 are disinct real numbers of points at which f(x) is minimum is
A
More than 3
B
1
C
2
D
3
Video Solution
Text Solution
The correct Answer is:A
The graph of f(x) is shown in Fig 1. It is evident from the graph of f(x) that it attains mimimum values at every point in [x1,x2]
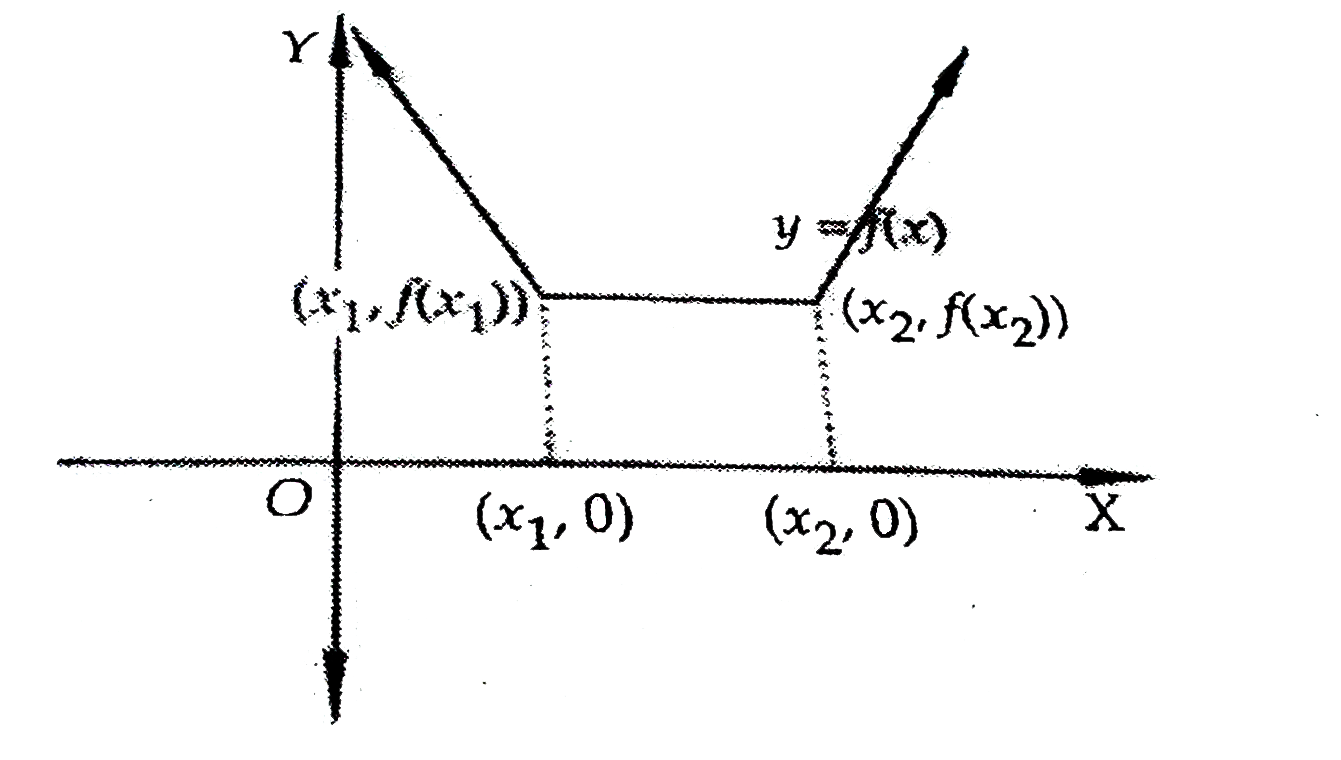
|
Topper's Solved these Questions
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMACHAPTER - MAXIMA AND MINIMA EXERCISE - Section I - Solved Mcqs
72 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMACHAPTER - MAXIMA AND MINIMA EXERCISE - Section II - Assertion Reason Type
7 Videos
- MATHEMATICAL REASONING BOOK - OBJECTIVE RD SHARMACHAPTER - MATHEMATICAL REASONING EXERCISE - Chapter Test
20 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMACHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
Similar Questions
Knowledge Check
Let f(x)=|x−x1|+|x−x2|, where x1andx2 are distinct real numbers. Then the number of points at which f(x) is minimum
A1B2C3Dmore than 3Let f(x)=x−∣∣x−x2∣∣,x∈[−1,1].Then the number of points at which f(x) is discontinuous is:
A0B1C2DnoneLet f(x) be given that f(x)={xif x is rational1−xif x is irrational
The number of points at which f(x) is continuous, isA∞B1C0Dnone of these
Let f(x)=x2−2x−1∀x∈R,Letf,(−∞,a]→[b,∞), where 'a' is the largest real number for which f(x) is bijective.
The value of (a+b) is equal to
View SolutionLet f(x)=2x+1x , x≠0, where
View SolutionLet f(x)=|x−2|+|2.5−x|+|3.6−x|, where x is a real number, attains a minimum at?
View SolutionLetf(x)=⎧⎨⎩[x]−2≤x≤−122x2−1−12<x≤2andg(x)=f(|x|)+|f(x)|, where [.] represents the greatest integer function .
the number of point where |f(x)| is non - differentiable is
View SolutionLetf(x)=⎧⎨⎩[x]−2≤x≤−122x2−1−12<x≤2andg(x)=f(|x|)+|f(x)|, where [.] represents the greatest integer function .
the number of point where g(x) is non - differentiable is
View Solution
