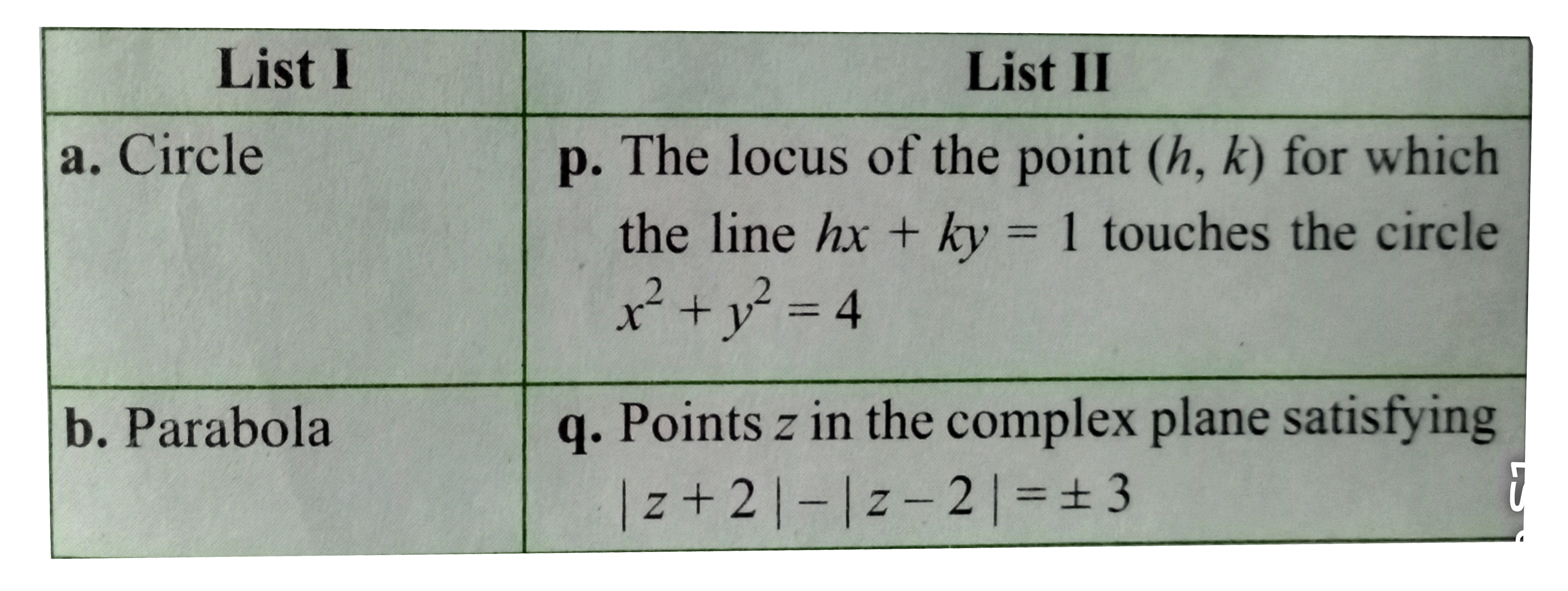
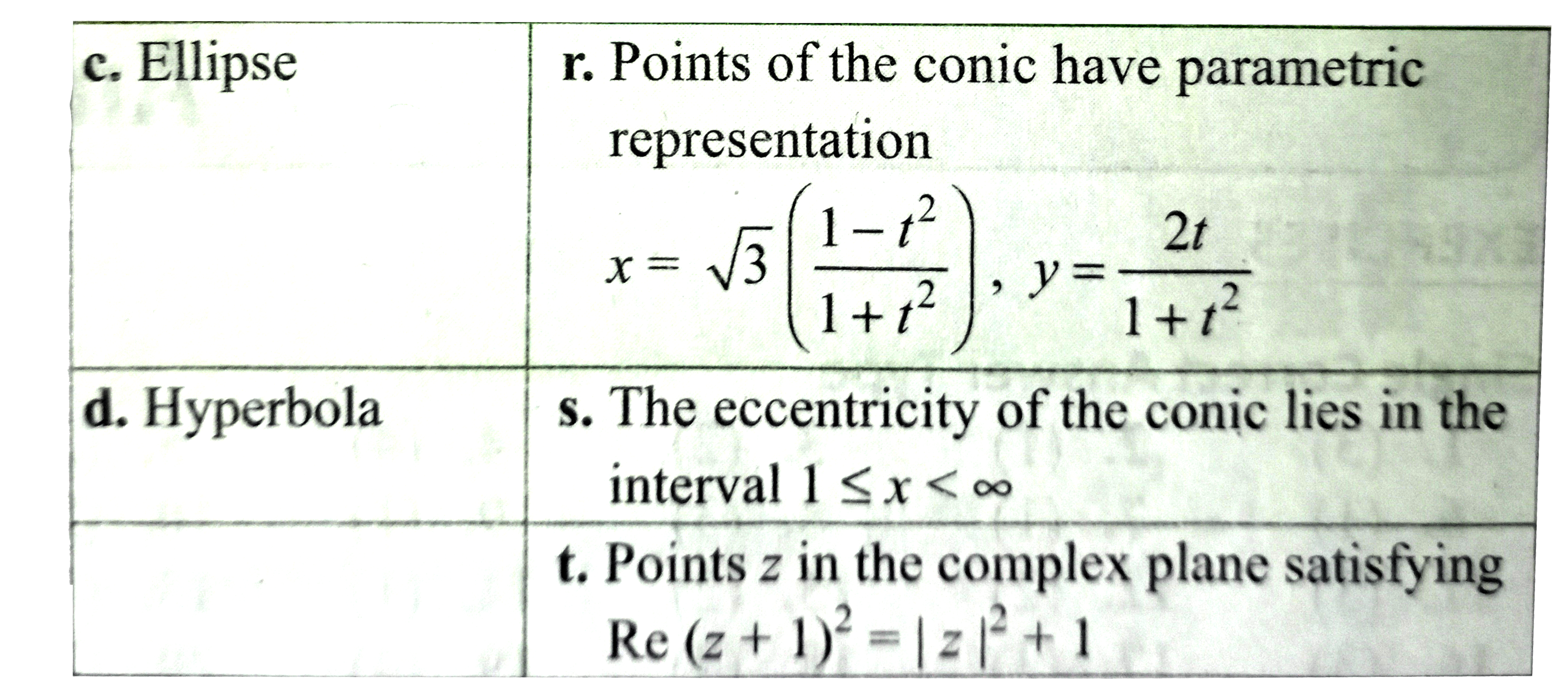
Let f(x)=x2−6x+5x2−5x+6. Then match the expressions/statements in List I with expression /statements in List II.

Video Solution
Text Solution
The correct Answer is:a→p,r,s;b→q,s;c→q,s;d→p,r,s.
We have f(x)=x2−6x+5x2−5x+6=(x−5)(x−1)(x−2)(x−3)
a→p,r,s.
If −1<x<1, then f(x)=(−ve)(−ve)(−ve)(−ve)=+ve
∴f(x)>0
Also, f(x)−1=−x−1x2−5x+6=−(x+1)(x−2)(x−3)
For −1<x<1,f(x)−1=−(+ve)(−ve)(−ve)=−ve
or f(x)−1<0orf(x)<1
∴0<f(x)<1
b→q,s.
If 1<x<2, then f(x)=(−ve)(+ve)(−ve)(−ve)=−ve
Therefore, f(x)<0 and, so, f(x)<1.
c→q,s.
If 3<x<5, then
f(x)=(−ve)(+ve)(+ve)(+ve)=−ve
Therefore, f(x)<0 and , so, f(x)<1.
d→p,r,s.
For x>5,f(x)>0, Also,
f(x)−1=−(x+1)(x−2)(x−5)<0 for x>5
orf(x)<1,
∴0<f(x)<1
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Numerical value Type31videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Archives(single correct Answer Type)9videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Linked Comprehension Type32videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (Numerical Value Type)3videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGE ENGLISHCHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.311videos
SCALER TRIPLE PRODUCTS
Similar Questions
Knowledge Check
- Question 1 - Select One
(3x4+2x3−7)+(4x6−5x3+9)
Which of the following expressions is equivalent to the expressions above?A4x6+3x4−3x3+2B4x6+3x4+7x3+2C4x6+3x4−5x3+2D7x10−3x3+2 - Question 2 - Select One
Which of the following statemens are true regarding the expression f(x)=x2−6x+11?
I. The expression has a least value of 11
II. The value of the expression is always positive for any value of x
III. The roots of f(x) =0 are realAOnly IBOnly IICBoth I and IIDBothII and III - Question 3 - Select One
3xx+5÷64x+20
Which of the following is equivalent to the expression above, given that x≠−5?A2xBx2C9x2D2x+4