Let l be the lower class limit of a class-interval in a frequency distribution and m be the mid-point of the class. Then, the upper class limit of the class is (a) m+l+m2 (b) l+m+12 (c) 2m−l (d) (m−2l)
To find the upper class limit of a class interval in a frequency distribution given the lower class limit and the midpoint, we can follow these steps:
1. Identify the Variables:
- Let l be the lower class limit.
- Let m be the midpoint of the class.
- We need to find the upper class limit, which we will denote as u.
2. Use the Formula for Midpoint:
The formula for the midpoint m of a class interval is given by:
m=l+u2
where l is the lower class limit and u is the upper class limit.
3. Rearrange the Formula:
To find u, we can rearrange the formula:
2m=l+u
4. Isolate u:
Now, we isolate u by subtracting l from both sides:
u=2m−l
5. Conclusion:
Therefore, the upper class limit u is given by:
u=2m−l
6. Select the Correct Option:
From the options provided:
- (a) m+l+m2
- (b) l+m+12
- (c) 2m−l
- (d) m−2l
The correct answer is option (c) 2m−l.
- Let l be the lower class limit.
- Let m be the midpoint of the class.
- We need to find the upper class limit, which we will denote as u.
The formula for the midpoint m of a class interval is given by:
m=l+u2
where l is the lower class limit and u is the upper class limit.
To find u, we can rearrange the formula:
2m=l+u
Now, we isolate u by subtracting l from both sides:
u=2m−l
Therefore, the upper class limit u is given by:
u=2m−l
From the options provided:
- (a) m+l+m2
- (b) l+m+12
- (c) 2m−l
- (d) m−2l
Topper's Solved these Questions
Similar Questions
Knowledge Check
If the upper and the lower limits of a class interval are 16 and 10, the class-interval is ...
A18−12B10−16C16−10D17−11
The width of each of five continuous classes in a frequency distribution is 5 and the lower class limit of the lowest class is 10. The upper class limit of the highest class is
View SolutionConsider the following frequency distribution

The upper limit of the median class in
View SolutionThe width of each of nine classes in a frequency distribution is 2.5 and the lower class boundary of the lowest class 10.6. Then the upper class boundary of the highest class is (a) 35.6 (b) 33.1 (c) 30.6 (d) 28.1
View SolutionIf m is the mid-point and l is the upper limit of a class in a continuous frequency distribution, then lower class limit of the class is
View SolutionFor the following frequency distribution

The upper limit of median class is
View SolutionConsider the data
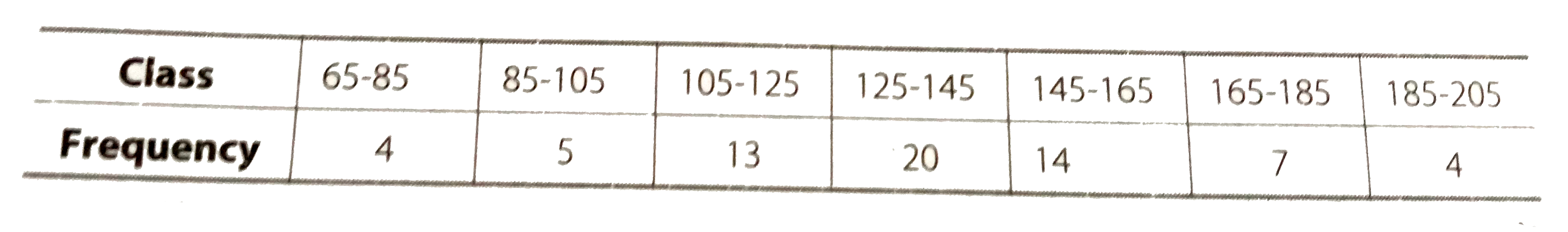
The frequency of the upper limit of the median class and the lower limit of the modal class is
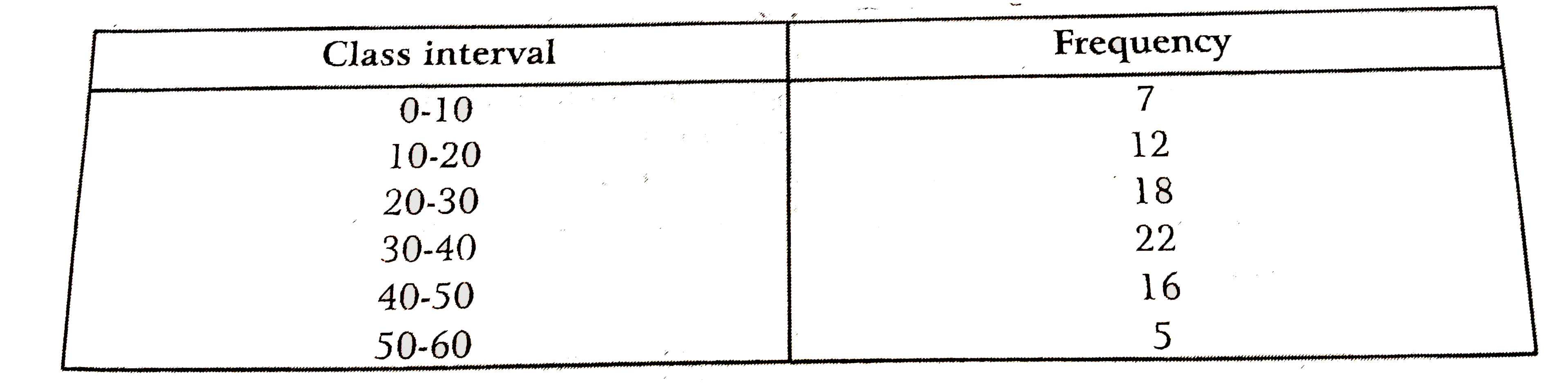
View SolutionThe table given below shows a frequency distribution table, find
(a) Upper limit of third class (b) Lower limit of fifth class
(c) Class size (d) Class mark of third class
View Solution
