Let P be any point on a directrix of an ellipse of eccentricity e,S be the corresponding focus, and C the center of the ellipse. The line PC meets the ellipse at A. The angle between at A is α. Then α is equal toSolution in Tamil
a)tan−1e
b)π/2
c)tan−1(1−e2)
d)none of these
The correct Answer is:B
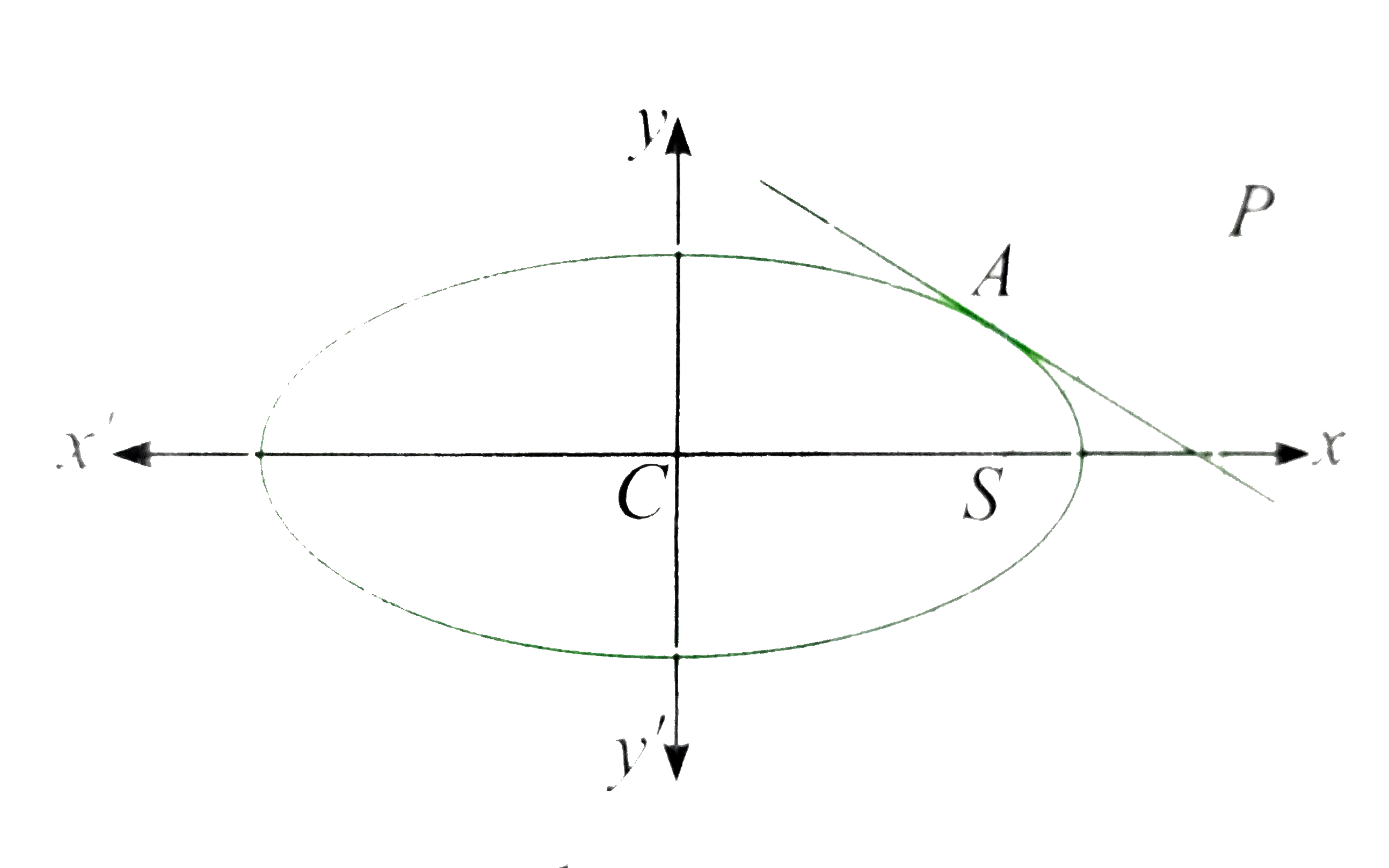
Let the ellipse be
x2a2+y2b2=1
and let A≡(acosθ,bsinθ)
The equation of AC will be
y=batanθx
Solving with x=a/e, we get
P≡(aebetanθ)
Slop of tangent at A=−batanθ
Slope of PS=betanθae−ae=btanθa(1−e2)=abtanθ
So, α=π2
Similar Questions
Prove that in an ellipse, the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact meet on the corresponding directrix.
View SolutionLet P be any point on a directrix of an ellipse of eccentricity e,S be the corresponding focus, and C the center of the ellipse. The line PC meets the ellipse at A. The angle between PS and tangent a A is α . Then α is equal to tan−1e (b) π2 tan−1(1−e2) (d) none of these
View SolutionLet P be any point on any directrix of an ellipse. Then the chords of contact of point P with respect to the ellipse and its auxiliary circle intersect at (a)some point on the major axis depending upon the position of point P. (b)the midpoint of the line segment joining the center to the corresponding focus (c)the corresponding focus (d)none of these
View Solutionमानक दीर्घवृत्त के बिन्दु α पर स्पर्श रेखा, सहायक वृत्त को दो बिन्दुओं पर मिलती है, जो केन्द्र पर समकोण अन्तरित करते हैं। तो प्रदर्शित कीजिए कि दीर्घवृत्त की उत्केन्द्रता (1+sin2α)−1/2 होगी।
View SolutionAn ellipse with eccentricity e=12 has a focus at (0,0) and the corresponding directrix x+6=0 . The equation of the ellipse is
View SolutionProve that the portion of the tangent to an ellipse intercepted between the ellipse and the directrix subtends a right angle at the corresponding focus.
View SolutionProve that in an ellipse, the perpendicular from a focus upon any tangent and the line joining the centre of the ellipse to the point of contact meet on the corresponding directrix.
View SolutionLet P be any point on a directrix of an ellipse of eccentricity e,S be the corresponding focus, and C the center of the ellipse. The line PC meets the ellipse at A. The angle between PS and tangent a A is α . Then α is equal to (a)tan−1e (b) π2 (c)tan−1(1−e2) (d) none of these
View Solution
