Let PQ be the perpendicular to the line segment XY. Let PQ and XY intersect in the point A. What is the measure of ?PAY?
To solve the problem step by step, we will follow the given information and draw the necessary conclusions.
1. Understanding the Problem:
- We have a line segment XY.
- There is a line PQ that is perpendicular to XY.
- The lines PQ and XY intersect at point A.
Hint: Visualize the scenario by drawing the lines XY and PQ.
2. Drawing the Figure:
- Draw a horizontal line segment XY. Label the endpoints as X and Y.
- Draw a vertical line PQ that intersects XY at point A. Label the points where PQ intersects XY as A, and the ends of PQ as P and Q.
Hint: Make sure to clearly mark the intersection point A where the two lines meet.
3. Identifying the Angles:
- Since PQ is perpendicular to XY, the angle formed between line PQ and line XY at point A is 90 degrees.
- We need to find the measure of angle PAY.
Hint: Remember that perpendicular lines create right angles.
4. Finding the Measure of Angle PAY:
- By definition of perpendicular lines, the angle between line PQ and line XY at point A (which is angle PAY) is 90 degrees.
Hint: Recall the properties of perpendicular lines to confirm your answer.
5. Conclusion:
- Therefore, the measure of angle PAY is 90 degrees.
Final Answer:
The measure of angle PAY is 90 degrees.
- We have a line segment XY.
- There is a line PQ that is perpendicular to XY.
- The lines PQ and XY intersect at point A.
- Draw a horizontal line segment XY. Label the endpoints as X and Y.
- Draw a vertical line PQ that intersects XY at point A. Label the points where PQ intersects XY as A, and the ends of PQ as P and Q.
- Since PQ is perpendicular to XY, the angle formed between line PQ and line XY at point A is 90 degrees.
- We need to find the measure of angle PAY.
- By definition of perpendicular lines, the angle between line PQ and line XY at point A (which is angle PAY) is 90 degrees.
- Therefore, the measure of angle PAY is 90 degrees.
The measure of angle PAY is 90 degrees.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
In the figure below, line m is perpendicular to line n, and both lines intersect line q at the same point. The measure of ∠1 is (3x−10)∘, and the measure of ∠2 is (2x+10)∘. What is the measure of ∠3?
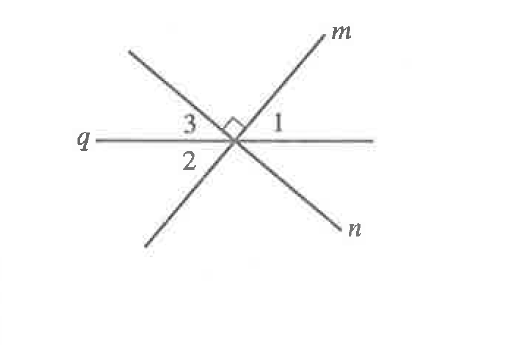 A36∘B40∘C44∘D45∘
A36∘B40∘C44∘D45∘
Draw a perpendicular to a given line segment though a point R on the line segment .
View SolutionLet PQ be the perpendicular form P(1,2,3) to xy-plane. If OP makes an angle theta with the positive direction of z-axis and OQ makes an angle ϕ with the positive direction of x-axis where O is the origin show that tanθ=√53andtanϕ=2.
View Solution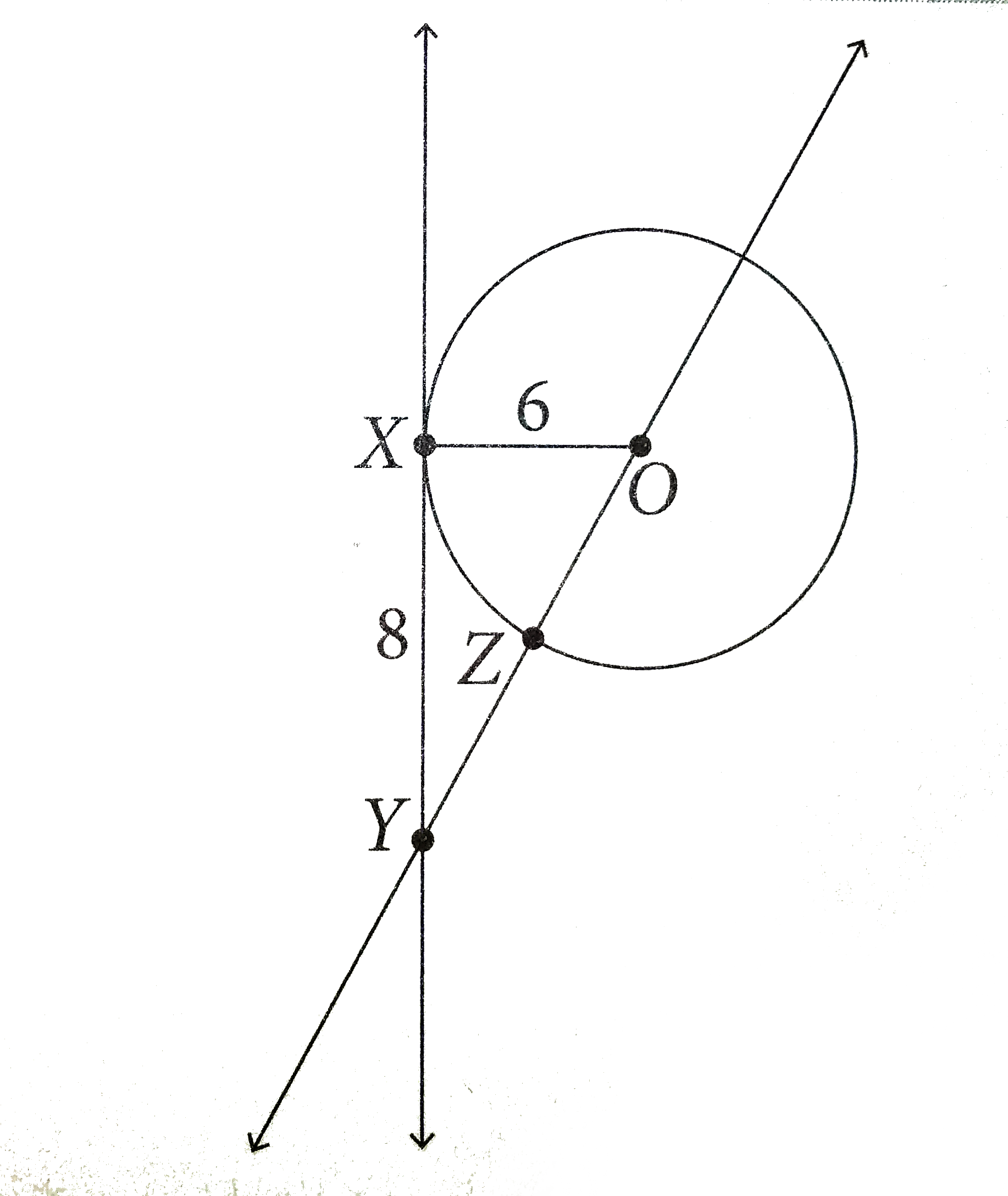
In the figure above, O is the center of the circle, the length of segment XY is 8, and the line passing through point X and Y is tangent to the circle at point X. What is the length of segment ZY?View SolutionLet a given line L1 intersect the X and Y axes at P and Q respectively. Let another line L2 perpendicular to L1 cut the X and Y-axes at Rand S, respectively. Show that the locus of the point of intersection of the line PS and QR is a circle passing through the origin
View Solution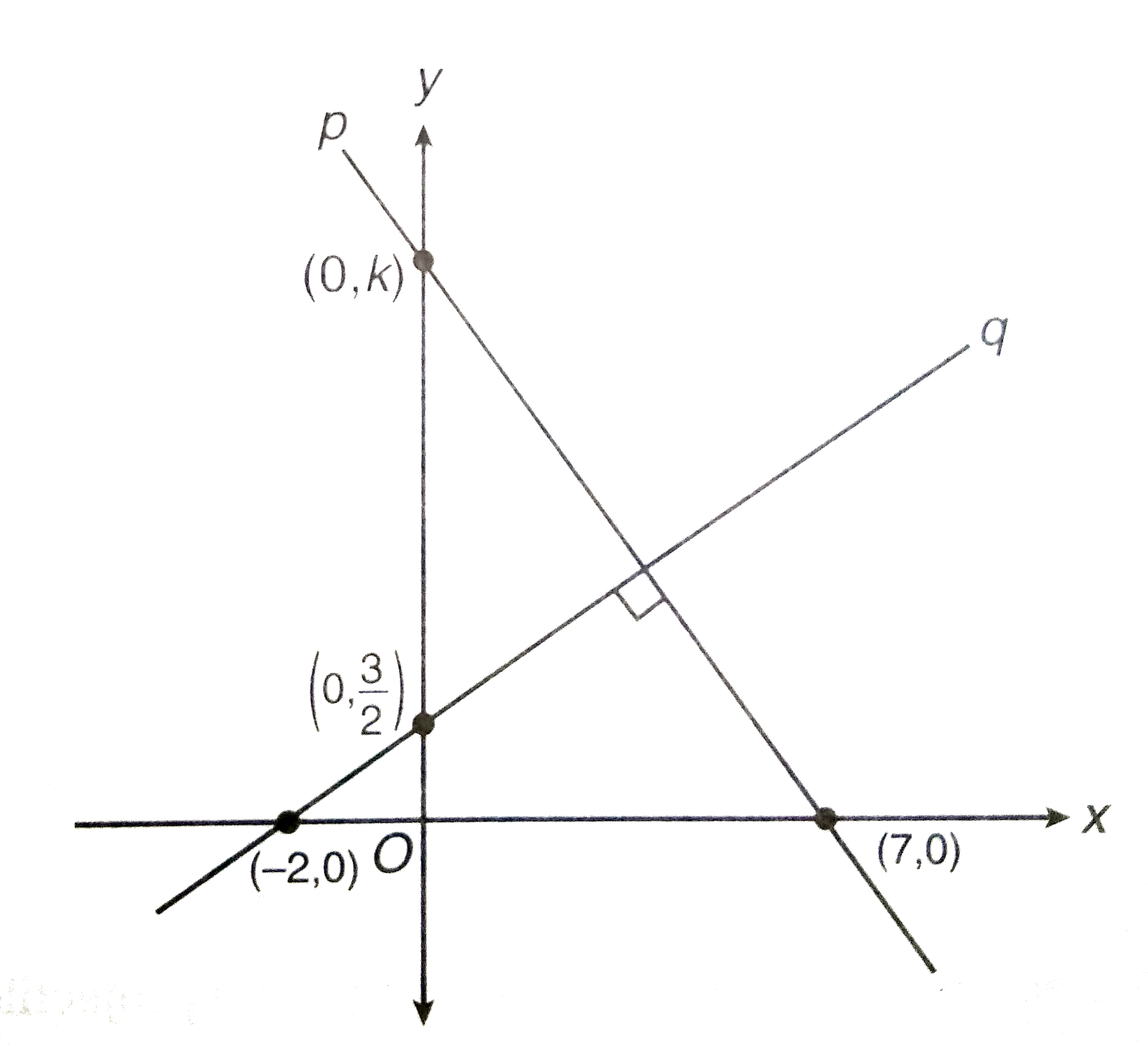
In the xy-plane above, line p is perpendicular to line q. What is the value of k?View SolutionIn the figure, BX bisects angle ABC and intersects AC at point D. Line segment CY is perpendicular to AB and intersects BX at point P. If Y is mid-point of AB, prove that : point P is equidistant from A and B.
View SolutionLet PQ and RS be tangents at the extremities of the diameter PR of a circle of radius r. If PS and RQ intersect at a point X on the circumference of the circle, then 2r equals
View Solution

