Let $P, Q, R$ and $S$ be the feet of the perpendiculars drawn from point $(1, 1)$ upon the lines $y = 3 x + 4, y = - 3 x + 6$ and their angle bisectors respectively. Then equation of the circle whose extremities of a diameter are $R$ and $S$ is

Text Solution

Verified by Experts

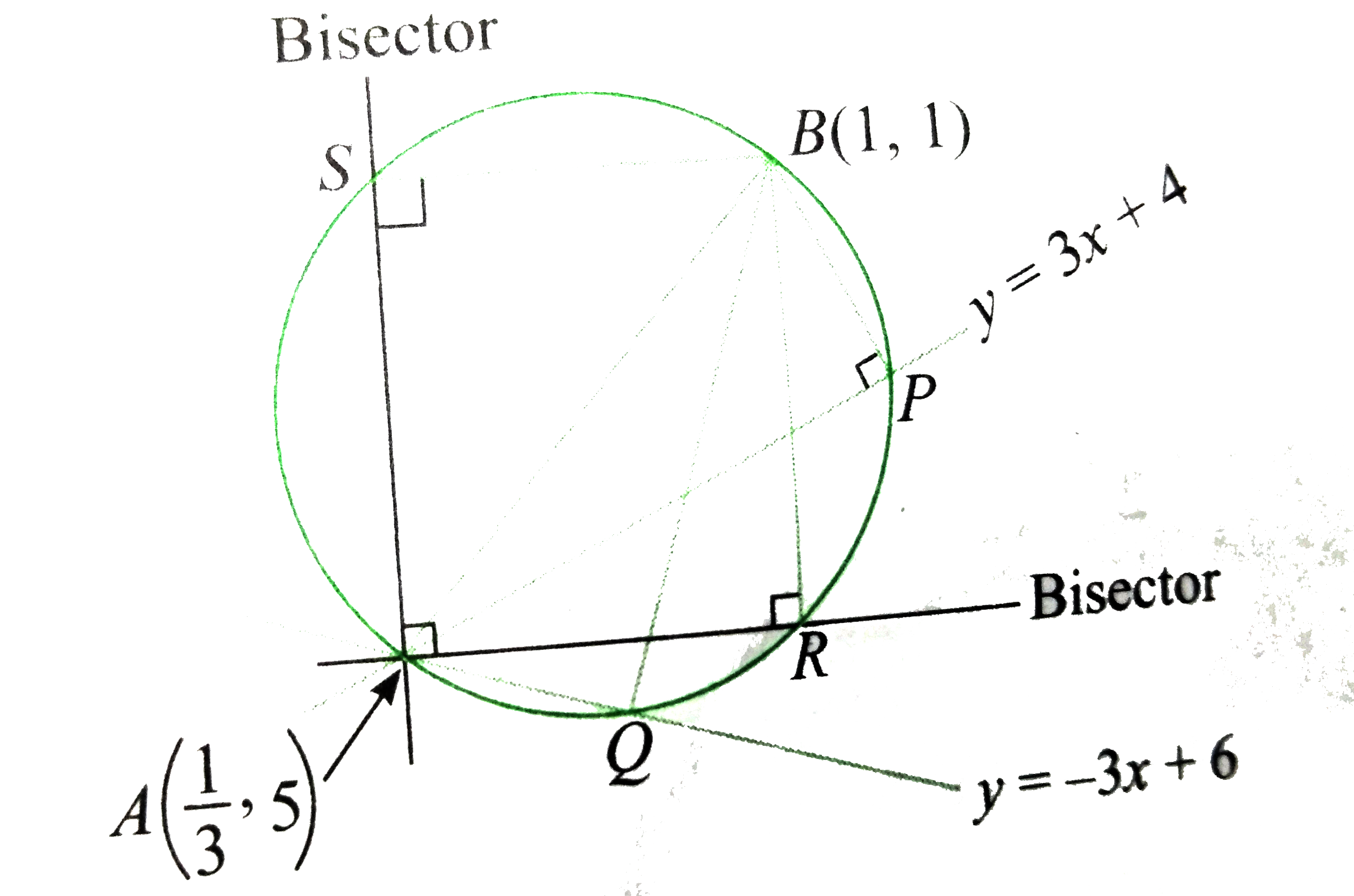$1 / 3, 5)$ .
Angle bisectors are always perpendicular.
So, if RS is diameter, then AB is also a diameter.
$∴$ Equation of the circle whose diameter is RS
$\equiv$ Equationof circle whose diameter is AB
$\equiv (x - 1) (x - 1 / 3) + (y - 5) (y - 1) = 0$
$\equiv 3 x^{2} + 3 y^{2} - 4 x - 18 y + 16 = 0$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Let P, Q, R, S be the feet of the perpendiculars drawn from a point (1, 1) upon the lines $x + 4 y = 12, x - 4 y + 4 = 0$ and their angle bisectors respectively, then equation of the circle which passes through Q, R, S is :
A $x^{2} + y^{2} - 5 x + 3 y - 6 = 0$
B $x^{2} + y^{2} - 5 x - 3 y + 6 = 0$
C $x^{2} + y^{2} - 5 x - 3 y - 6 = 0$
DNone of these
Question 2 - Select One
Let P, Q, R, S be the feet of the perpendiculars drawn from a point (1, 1) upon the lines $x + 4 y = 12, x - 4 y + 4 = 0$ and their angle bisectors respectively, then equation of the circle which passes through Q, R, S is :
A $x^{2} + y^{2} - 5 x + 3 y - 6 = 0$
B $x^{2} + y^{2} - 5 x - 3 y + 6 = 0$
C $x^{2} + y^{2} - 5 x - 3 y - 6 = 0$
DNone of these
Question 3 - Select One
What will be the length of the perpendicular drawn from the point (4, 5) upon the straight line 3x + 4y = 10?
A $\frac{12}{5}$
B $\frac{32}{5}$
C $\frac{22}{5}$
D $\frac{42}{5}$

CENGAGE PUBLICATION-CIRCLE -For problems 3 and 4

Home
Ask Doubt
Profile