Let P(x) denote the probability of the occurrence of event x. Plot all those point (x,y)=(P(A),P(B)) in a plane which satisfies the conditions, P(A∪B)≥3/4and1/8≤P(A∩B)≤3/8Solution in Bengali
P(A∪B)≥34and18≤P(A∩B)≤38
Hence,
P(A)+P(B)−P(A∩B)≤34
implies P(A)+P(B)≥34+P(A∩B)≥34+18=78
⇒x+y≥78
We know that
P(A∩B)≤1
⇒P(A)+P(B)≤1+P(A∩B)≤1+38=118
⇒x+y≤118
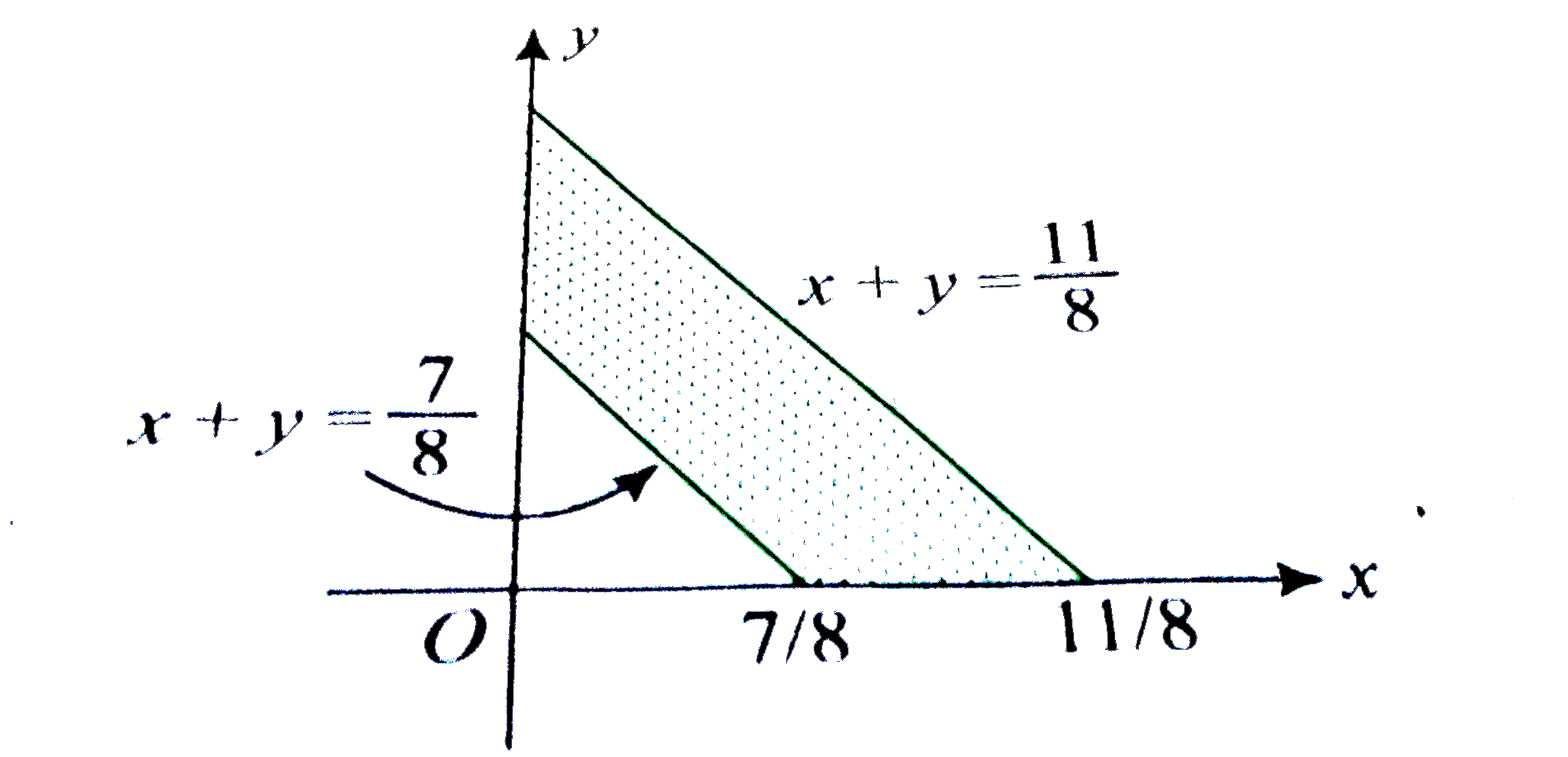
The shaded part in the figure is the required region.
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITY I EXERCISE - Exercise 9.16videos
PROBABILITY I
- BOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITY I EXERCISE - Exercise 9.219videos
PROBABILITY I
- BOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITY I EXERCISE - JEE Advanced7videos
PROBABILITY I
- BOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITYEXERCISE - All Questions470videos
PROBABILITY
- BOOK - CENGAGE PUBLICATIONCHAPTER - PROBABILITY IIEXERCISE - MULTIPLE CORRECT ANSWER TYPE6videos
PROBABILITY II
Similar Questions
Which of the following cannot be valid assignment of probabilities for outcomes of sample space S={W1,W2,W3,W4,W5,W6,W7}
 06:12View Solution
06:12View SolutionIf two fair dices are thrown and digits on dices are a and b, then find the probability for which ωab=1, (where ω is a cube root of unity).
05:11View SolutionLet ω be a complex cube root unity with ω≠1. A fair die is thrown three times. If r1,r2andr3 are the numbers obtained on the die, then the probability that ωr1+ωr2+ωr3=0 is 1/18 b. 1/9 c. 2/9 d. 1/36
03:01View SolutionA coin is tossed three times, consider the following events. A : ‘No head appears’, B: ‘Exactly one head appears’ and C: ‘Atleast two appear’. Do they form a set of mutually exclusive and exhaustive events?
01:32View SolutionLet EandF be two independent events. The probability that exactly one of them occurs is 1125 and the probability if none of them occurring is 225. If P(T) denotes the probability of occurrence of the event T, then (a) P(E)=45,P(F)=35 (b) P(E)=15,P(F)=25 (c) P(E)=25,P(F)=15 (d) P(E)=35,P(F)=45
05:53View SolutionFor a point P in the plane, let d1 (P) and d2 (P) be the distance of the point P from the lines x – y = 0 and x + y = 0 respectively. The area of the region R consisting of all points P lying in the first quadrant of the plane and satisfying 2 < d1(P) + d2 (P)< 4, is
02:33View SolutionLet P(x) denote the probability of the occurrence of event x. Plot all those point (x,y)=(P(A),P(B)) in a plane which satisfies the conditions, P(A∪B)≥3/4and1/8≤P(A∩B)≤3/8
03:30View SolutionLet AandB be two event such that P(A∪B)≥3/4 and 1/8≤P(A∩B)≤3/8. Statement 1: P(A)+P(B)≥7/8. Statement 2:P(A)+P(B)≤11/8.
06:25View SolutionIf A is an event and P(A) and P(vec A) denote the probabilities of occurrence and non occurrence respectively of event A, the P(A)+P(vec A)
View SolutionLet P(x) denote the probability of the occurrence of event x. Plot all those point (x y) = (P(A), P(B)) in a plane which satisfy the conditions, P(A∪B)≥3/4and1/8≤P(A∩B)≤3/8
04:18View Solutionमाना कि A और B दो घटनाएँ है तथा P(A)=0.3P(B)=0.4,P(A∪B)=0.5 तो P(A∩B) है।
04:00View SolutionP(A∪B)=P(A)−P(B)+P(A∩B)
07:01View SolutionIf A and B are any two events such that P(A)=25 and P(A∩B)=320, then the conditional probability, P(A/(A'∪B')), where A' denotes the complement of A, is equal to
05:55View SolutionIf A and B are two events such that P(A∪B)=56, P(A∩B)=13 and P(A)=23 then A and B are
03:26View SolutionLet P(x) denote the probability of the occurrence of event x. Plot all those point (x,y)=(P(A),P(B)) in a plane which satisfies the conditions, P(A∪B)≥3/4and1/8≤P(A∩B)≤3/8
06:25View SolutionLet AandB be two event such that P(A∪B)≥3/4 and 1/8≤P(A∩B)≤3/8. Statement 1: P(A)+P(B)≥7/8. Statement 2:P(A)+P(B)≤11/8.
04:07View Solution

