Let us consider the Example 3 of Section 3.2. Will the rails cross each other?
To determine whether the two rails represented by the equations x+2y−4=0 and 2x+4y−12=0 cross each other, we will follow these steps:
Step 1: Write down the equations
We have the following equations:
1. x+2y−4=0 (Equation 1)
2. 2x+4y−12=0 (Equation 2)
Step 2: Rearrange the equations to find y in terms of x
For Equation 1:
x+2y−4=0⟹2y=4−x⟹y=2−x2
For Equation 2:
2x+4y−12=0⟹4y=12−2x⟹y=3−x2
Step 3: Find points for each equation
We will find two points for each equation to plot them.
For Equation 1:
1. Let x=0:
y=2−02=2⟹(0,2)
2. Let x=4:
y=2−42=0⟹(4,0)
For Equation 2:
1. Let x=0:
y=3−02=3⟹(0,3)
2. Let x=6:
y=3−62=0⟹(6,0)
Step 4: Plot the points on a graph
- For Equation 1, plot the points (0,2) and (4,0).
- For Equation 2, plot the points (0,3) and (6,0).
Step 5: Draw the lines
- Draw a line through the points (0,2) and (4,0) for Equation 1.
- Draw a line through the points (0,3) and (6,0) for Equation 2.
Step 6: Analyze the lines
Upon plotting the lines, we observe that:
- The first line (from Equation 1) slopes downwards and intersects the y-axis at (0,2) and the x-axis at (4,0).
- The second line (from Equation 2) also slopes downwards but intersects the y-axis at (0,3) and the x-axis at (6,0).
Conclusion
The two lines are parallel because they have the same slope (both have a slope of −12). Therefore, they will never intersect or cross each other.
Final Answer
No, the rails will not cross each other as they are parallel lines.
---
We have the following equations:
1. x+2y−4=0 (Equation 1)
2. 2x+4y−12=0 (Equation 2)
For Equation 1:
x+2y−4=0⟹2y=4−x⟹y=2−x2
2x+4y−12=0⟹4y=12−2x⟹y=3−x2
We will find two points for each equation to plot them.
1. Let x=0:
y=2−02=2⟹(0,2)
2. Let x=4:
y=2−42=0⟹(4,0)
1. Let x=0:
y=3−02=3⟹(0,3)
2. Let x=6:
y=3−62=0⟹(6,0)
- For Equation 1, plot the points (0,2) and (4,0).
- For Equation 2, plot the points (0,3) and (6,0).
- Draw a line through the points (0,2) and (4,0) for Equation 1.
- Draw a line through the points (0,3) and (6,0) for Equation 2.
Upon plotting the lines, we observe that:
- The first line (from Equation 1) slopes downwards and intersects the y-axis at (0,2) and the x-axis at (4,0).
- The second line (from Equation 2) also slopes downwards but intersects the y-axis at (0,3) and the x-axis at (6,0).
The two lines are parallel because they have the same slope (both have a slope of −12). Therefore, they will never intersect or cross each other.
No, the rails will not cross each other as they are parallel lines.
Topper's Solved these Questions
- BOOK - NCERT ENGLISHCHAPTER - PAIR OF LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - EXERCISE 3.62videos
PAIR OF LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - NCERT ENGLISHCHAPTER - PAIR OF LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - EXERCISE 3.54videos
PAIR OF LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - NCERT ENGLISHCHAPTER - PAIR OF LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Exercise 3.27videos
PAIR OF LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 10.12videos
NCERT THEOREMS
- BOOK - NCERT ENGLISHCHAPTER - POLYNOMIALSEXERCISE - EXERCISE 2.45videos
POLYNOMIALS
Similar Questions
Let us consider Example 2 in Section 3.3, i.e., the cost of 2 pencils and 3 erasers is Rs 9 and the cost of 4 pencils and 6 erasers is Rs 18. Find the cost of each pencil and each eraser.
View SolutionTwo streamlines cannot cross each other. Why?
View SolutionWhy do the electric field lines never cross each other ?
View SolutionTwo equal and opposite point forces applied at mid- points of the ends of a rod of square cross shown. Consider the dotted section ABCD. If the rod is cut across this Gloss section, the force exerted by the right part of the rod on left part across this cross section is
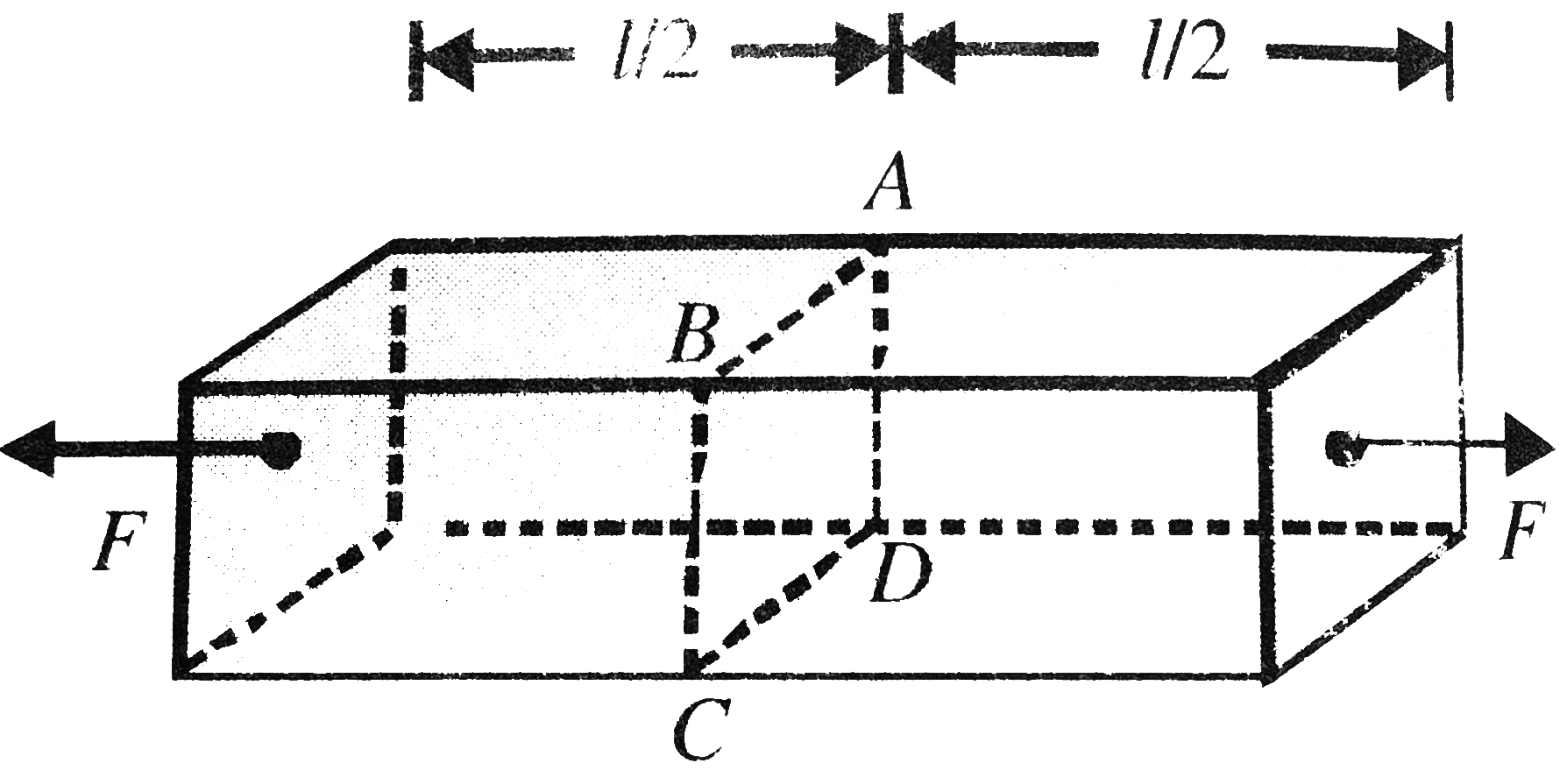 View Solution
View SolutionHow do the back cross and test cross from each other?
View SolutionCan two streamlines in a flowing liquid cross each other ?
View SolutionTwo trains running in opposite directions to each other, cross a man standing on the platform in 30 sec and 12 sec respectively and they cross each other in 20 seconds. Find the ratio of their speed.
View SolutionConsidering a wire of non-uniform cross-section as shown.If the area of cross-section at A is double of the area of cross-section at point B.Ratio of heat energy dissipated in a unit volume per unit time at points A and B is
_PHY_E05_279_Q01.png) View Solution
View SolutionNumber of genotypes produced when individuals of genotype 'YyRrTt' are crossed with each other
View SolutionA piston of cross-sectional area 100cm2 is used in a hydraulic pressure to exert a force of 107 dyne on the water. The cross-sectional area of the other piston which support a truck of mass 2000 kg is
View Solution
