Let X is a continuous random variable with probability density function
The value of k is equal to
The correct Answer is:A
Step by step video, text & image solution for Let X is a continuous random variable with probability density function f(x)={{:(x/6+k,0lexle3),(0," otherwise"):} The value of k is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROBABILITY DISTRIBUTIONBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - Competitive Thinking
9 Videos
- PROBABILITY DISTRIBUTIONBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - Evaluation test
5 Videos
- PROBABILITY DISTRIBUTIONBOOK - TARGET PUBLICATIONCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - Evaluation test
5 Videos
- PLANEBOOK - TARGET PUBLICATIONCHAPTER - PLANEEXERCISE - EVALUATION TEST
14 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - TARGET PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - Evaluation Test
9 Videos
Similar Questions
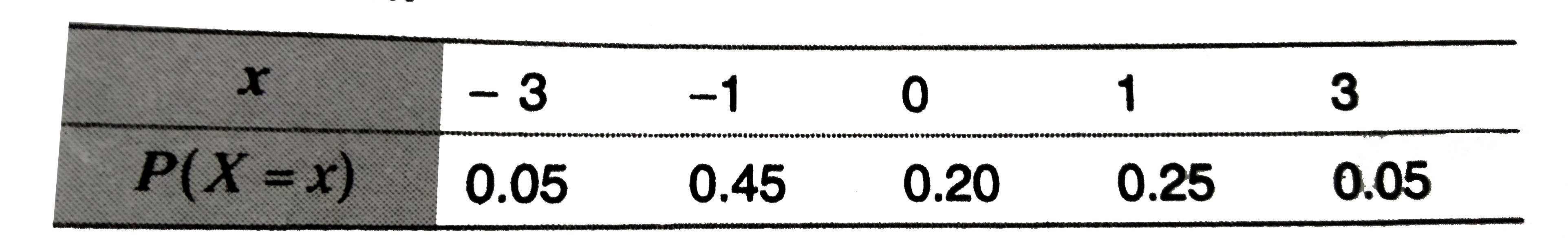
The random variable X has the following probability distribution
Then, F(0) is equal to
View SolutionIf a random variable waiting time in minutes for bus and probability density function of x is given by
f(x)={15,0≤x≤50,otherwise
Then probability of waiting time not more than 4 minutes is equal to
View Solution
Knowledge Check
X is continuous random variable with probability density function f(x)=x28,0≤x≤1. Then, the value of P(0.2≤X≤0.5) is
A0.11724B0.11224C0.11336D0.11236If a curve X has probability density function (pdf)
f(x)=⎧⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪⎩ax,0≤x≤1a,1≤x≤23a−ax,2≤x≤30,otherewise
Then, a is equal toA1B13C12D15If a curve X has probability density function (pdf)
f(x)=⎧⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪⎩ax,0≤x≤1a,1≤x≤23a−ax,2≤x≤30,otherewise
Then, a is equal toA1B13C12D15
If X is the a random variable with probability mass function P(x)=kx, for x = 1,2,3
= 0 , otherwise
then k = ……..
View SolutionThe pdf of a discrete random variable is defined as f(x)={kx2,0≤x≤60, elsewhere
Then the value of F(4) is
View SolutionIf the p.d.f of a random variable X is
F(x)={0.5x0≤x≤20otherwise
Then the value of P(0.5≤X≤1.5) is
View SolutionIf the probability density function of a continuous random variable X is f(x)={3+2x182≤x≤40x<2 orx>4 Then the mathematical expectation of X is
View SolutionIf the probability density function of a random variable X is f(x)=x2 in 0≤x≤2, then P(X>1.5∣X>1) is equal to
View Solution
