Let [x] represent the greatest integer less than or equal to x If [√n2+λ]=[n2+1]+2 , where λ,n∈N, then λ can assume (a) (2n+4)different values (b) (2n+5)different values (c) (2n+3)different values (d) (2n+6)different valuesSolution in Punjabi
Step by step video & image solution for Let [x] represent the greatest integer less than or equal to x If [sqrt(n^2+lambda)]=[n^2+1]+2 , where lambda,n in N , then lambda can assume (a) (2n+4)different values (b) (2n+5)different values (c) (2n+3)different values (d) (2n+6)different values by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FUNCTIONSBOOK - ARIHANT MATHSCHAPTER - FUNCTIONSEXERCISE - Exercise For Session 1
5 Videos
- FUNCTIONSBOOK - ARIHANT MATHSCHAPTER - FUNCTIONSEXERCISE - Exercise For Session 2
6 Videos
- ESSENTIAL MATHEMATICAL TOOLS BOOK - ARIHANT MATHSCHAPTER - ESSENTIAL MATHEMATICAL TOOLS EXERCISE - Exercise (Single Integer Answer Type Questions)
3 Videos
- GRAPHICAL TRANSFORMATIONSBOOK - ARIHANT MATHSCHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
10 Videos
Similar Questions
Which of the following graphs are graphs of a function?

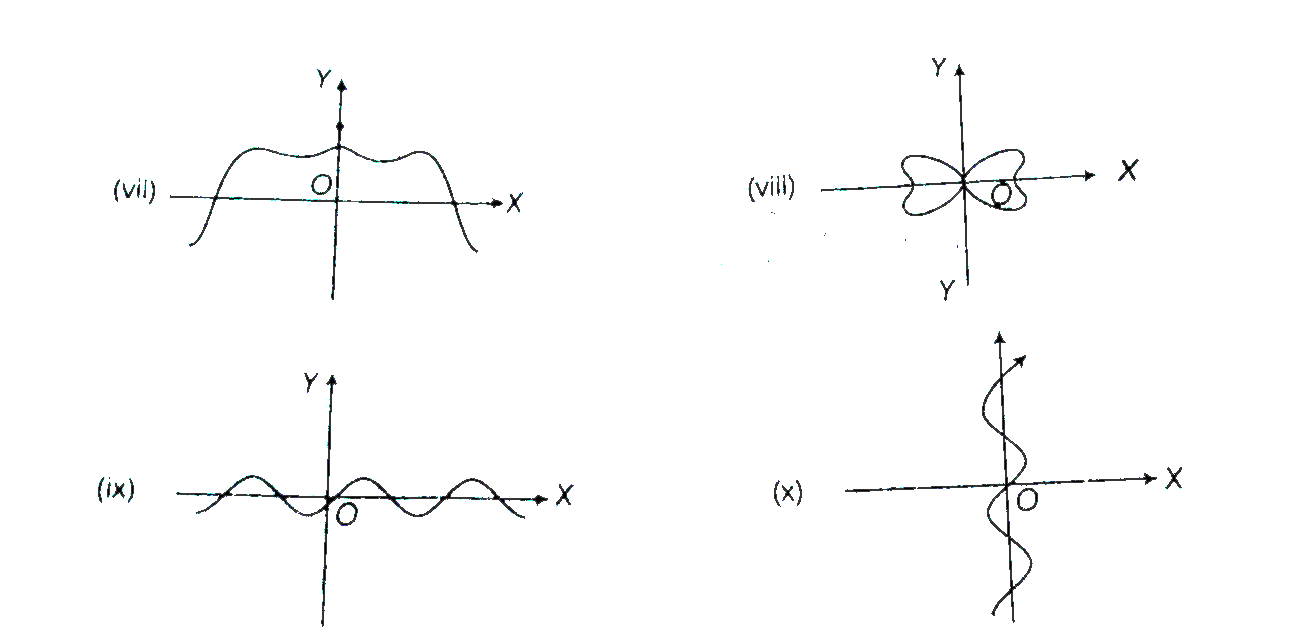
View SolutionThe domain of the function
f(x)=√x2−5x+6+√2x+8−x2 , is
View SolutionThe number of solutions of the equation |x−1|−|2x−5|=2x
View SolutionFind the number of solutions of the equation x21−|x−2|=1, graphically.
View SolutionLet [x] represent the greatest integer less than or equal to x If [√n2+λ]=[√n2+1]+2 , where λ,n∈N, then λ can assume (a) 2n+4 different values (b)2n+5 different values (c)2n+3 different values (d)2n+6 different values
View SolutionThe value of lim_(x rarr oo)(5^(n+1)+3^(n)-2^(2n))/(5^(n)+2^(n)+3^(2n+1))
View SolutionIf (sqrt(2n^(2)+n)-lambda sqrt(2n^(2)-n))=(1)/(sqrt(2))( where lambda is real number),then
View SolutionThe value of limn→∞[2n2n2−1cosn+12n+1−n1−2n.nn2+1] is
View SolutionThe value of sum ∞∑n=1cot−1((n2+2n)(n2+2n+1)+12n+2) is equal to If f(x+y)=f(x)+f(y)−xy−1, ∀x,y in R andf(1)=1,thentheνmberofsolutionoff(n)=n,n in N , is
View Solution(i) यदि n!2⋅(n−2)!:n!4!⋅(n−4)!=2:1, तो n का मान ज्ञात कीजिए ।
(ii) यदि (2n)!3!(2n−3)!:n!2!(n−2)!=44:3, तो n का मान ज्ञात कीजिए ।
View SolutionIf n is positive integer then 2.42n+1+33n+1 is always divisible by a positive integer whose values is λ then λ−2=
View Solution2n.1.3.5.7....(2n−1) का मान है
View SolutionLet [x] represent the greatest integer less than or equal to x If [√n2+λ]=[√n2+1]+2 , where λ,n∈N, then λ can assume (a) 2n+4 different values (b)2n+5 different values (c)2n+3 different values (d)2n+6 different values
View SolutionThe value of limn→∞[2n2n2−1cosn+12n−1−n1−2n.nn2+1] is
View SolutionThe value of sum ∞∑n=1cot−1((n2+2n)(n2+2n+1)+12n+2) is equal to
View SolutionLet x=(2n+1)(2n+3)(2n+5)…(4n−3)(4n−1) and y=(12)n(4n)!n!(2n)!(2n)!, then x−y+2n is equal to
View Solution
