Let x1,x2,…………,xn be n observations and ¯x be their arithmetic mean. The formula for the standard deviation is given by ……….. .Solution in Gujrati
∑(xi=¯x)2
∑(xi−¯x)2n
√∑(xi−¯x)2n
√∑x2in−(¯x)−2
The correct Answer is:C
Step by step video, text & image solution for Let x_1, x_2 ,…………,x_n be n observations and barx be their arithmetic mean. The formula for the standard deviation is given by ……….. . by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - STATISTICSEXERCISE - NCERT EXEMPLAR PROBLEMS (FILLERS)6videos
STATISTICS
- BOOK - KUMAR PRAKASHANCHAPTER - STATISTICSEXERCISE - QUESTION OF MODULE (KNOWLEDGE TEST)9videos
STATISTICS
- BOOK - KUMAR PRAKASHANCHAPTER - STATISTICSEXERCISE - NCERT EXEMPLAR PROBLEMS (SHORT ANSWER TYPE QUESTIONS)23videos
STATISTICS
- BOOK - KUMAR PRAKASHANCHAPTER - SETSEXERCISE - QUESTION OF MODULE28videos
SETS
- BOOK - KUMAR PRAKASHANCHAPTER - STRAIGHT LINESEXERCISE - Question of Module (Knowledge Test :)18videos
STRAIGHT LINES
Similar Questions
Coefficient of variation = ………….Mean×100
01:10View SolutionFind average deviation of 1,2,3,4,5
03:09View SolutionFind the mean deviation about the mean of the distribution:
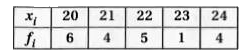 02:43View Solution
02:43View SolutionA{x∈R∣x2+3=0} is a set of………type.
02:28View SolutionFind distance between the points A(3,4)andB(−4,3).
02:32View SolutionIf x1,x2……..xn be n observation and ¯x be their arithmetic mean .Then formula of the standard deviation is given by
04:49View Solutionमाना x1,x2,…,xn का माध्य ¯x है ,तब a≠0 के लिए ax1,ax2,..,axn,x1a,x2a,….,xnaका माध्य है -
01:16View SolutionMean of n observations x1,x2,….,xn is ¯x. If an observation xq is replaced by x'q, then the new mean is :
03:34View Solutionयदि n स्वतन्त्र चरों x1,x2,x3,…,xn का समान्तर माध्य ¯x तथा मानक विचलन σ हो, तो प्रसरण (¯x) होगा
01:58View Solutionn प्रेक्षणों x1,x2,…,xn का उनके माध्य के सापेक्ष माध्य विचलन है
04:08View SolutionIf the mean of n observation ax1,ax2,ax3,,axn is a¯¯¯X , show that (ax1−a¯¯¯X)+(ax2−a¯¯¯X)++(axn−a¯¯¯X)=0
02:27View SolutionMean deviation for n observation x1,x2,……….,xn from their mean ¯x is given by …….
02:24View SolutionLet ¯x be the mean of x1,x2,……,xn and ¯y the mean of y1,y2……,yn ¯z is the mean of x1,x2,….,xn,y1,y2 is equal to :
01:54View SolutionLet x1,x2,……,xn be n observations and ¯x be their arithmetic mean. The formula for the standard deviation is
03:30View SolutionIf the mean of observationsx1.x2,x3…..xnis¯x then the mean of x1+a,x2+a,…...xn+a will be
02:50View Solution
