Let be a quadratic equation . Find the values of m for which the roots are (ix)one root is smaller than 2 & other root is greater than 2 (x) both the roots are greater than 2 (xi) both the roots are smaller than 2 (xii)exactly one root lies in the interval (1;2) (xiii) both the roots lies in the interval (1;2) (xiv) atleast one root lies in the interval (1;2) (xv) one root is greater than 2 and the other root is smaller than 1
Let
Here
and
and -x coordinate of vertex
(i) Both the roots are smaller than 2
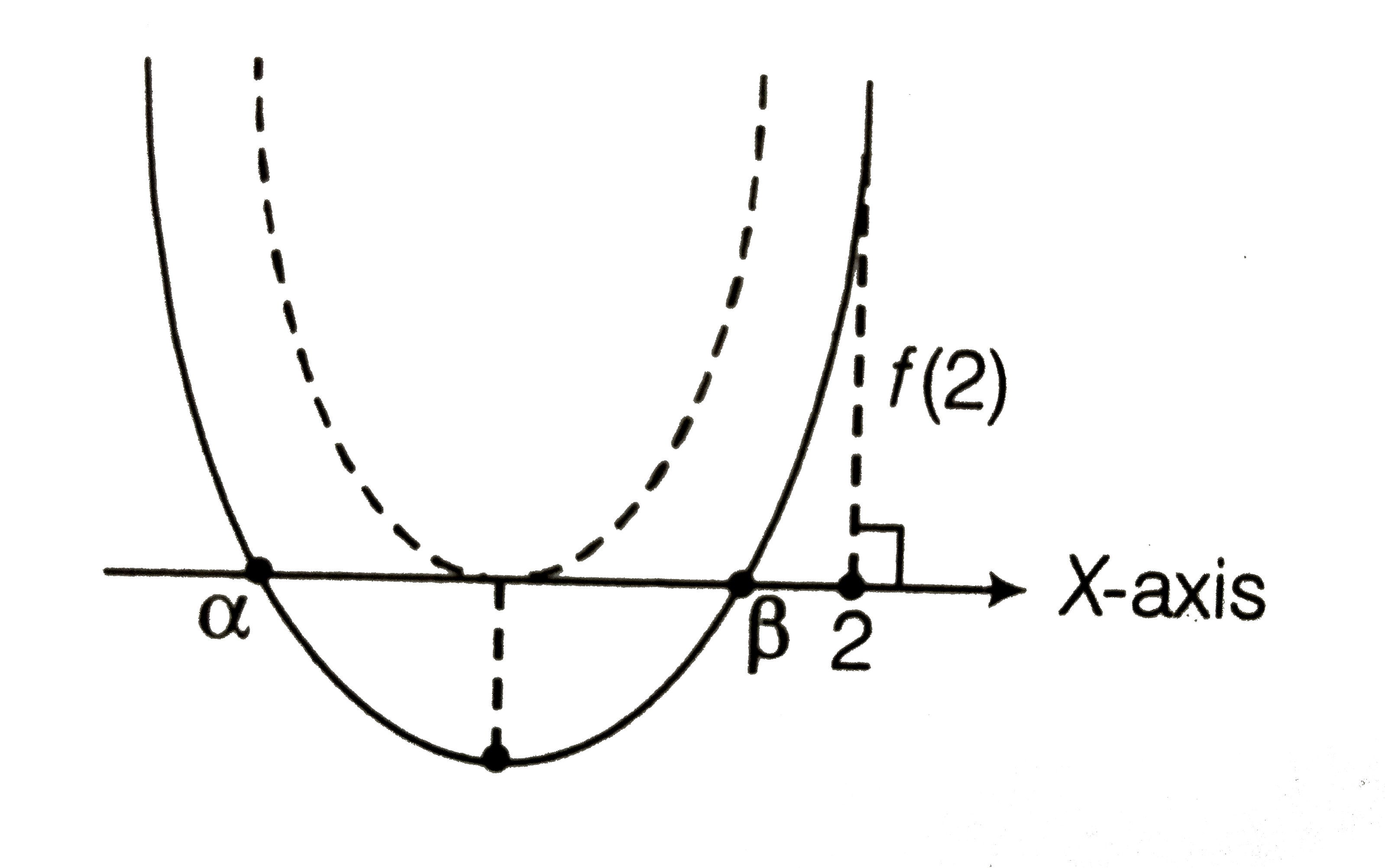
i.e.
............i
i.e.
......ii and - coordinate of vertex
i.e.
iii
On combining Eqs.i,ii, and iii we get
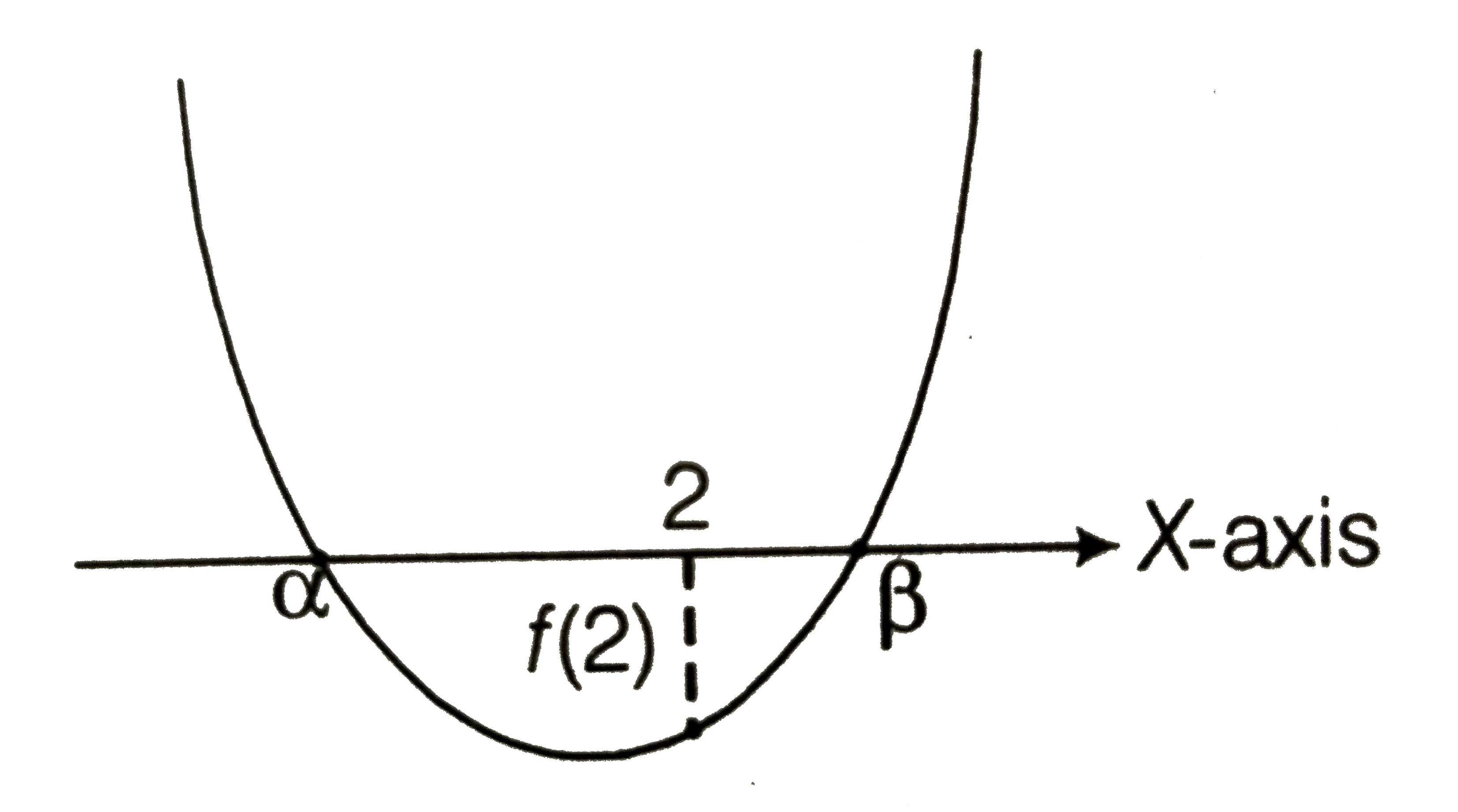
(ii) Both the roots are greater than 2
i.e.
.........i
i.e.
......ii
and x-coordinate of vertex
i.e.
.....iii
On combining Eqs. i, ii and iii we get
(iii) One root is smaller than 2 and the other root is greater than 2
i.e.
....i
i.e
....ii
On combining Eqs i and ii we get
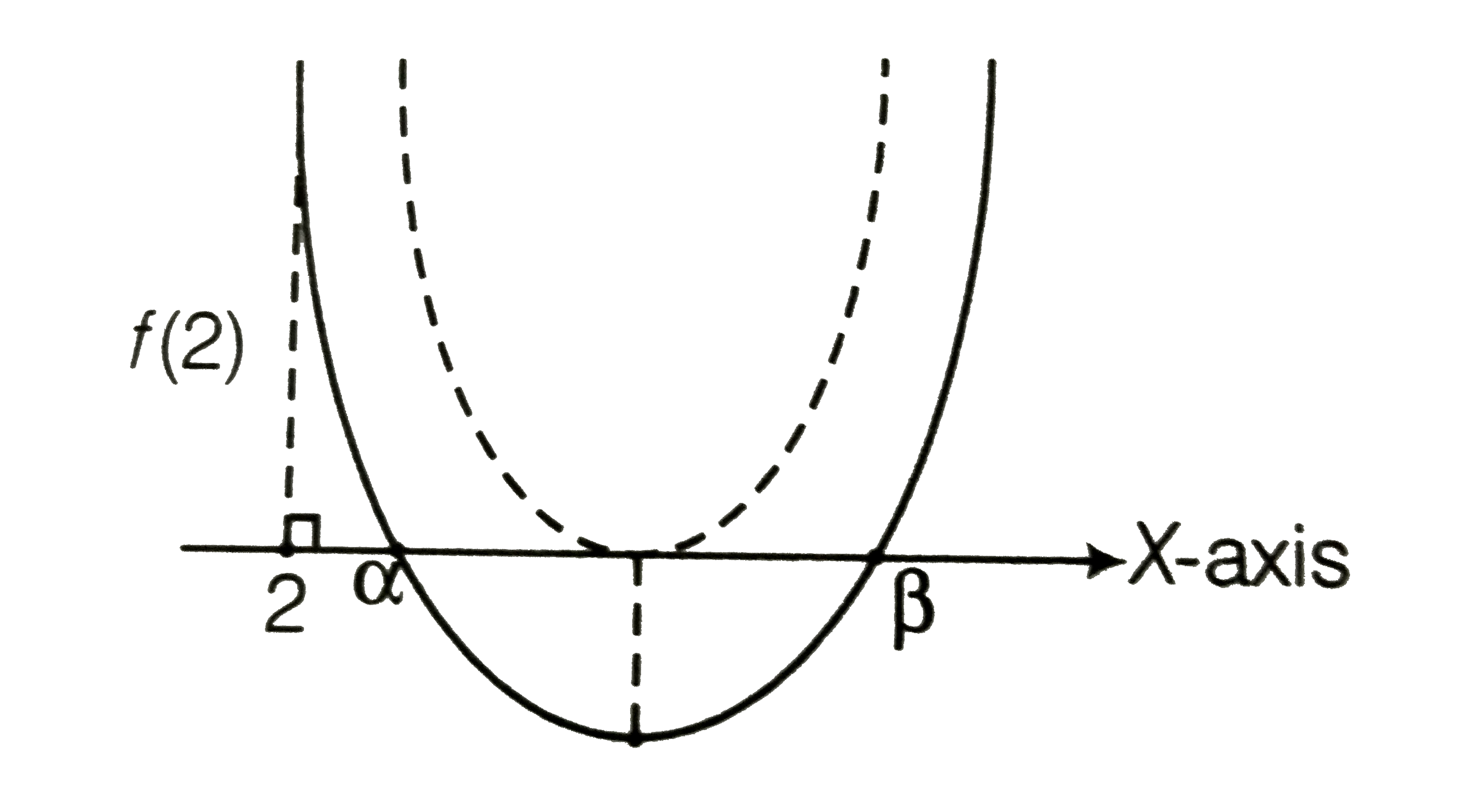
(iv) Exactly one root lies in the interval (1,2)
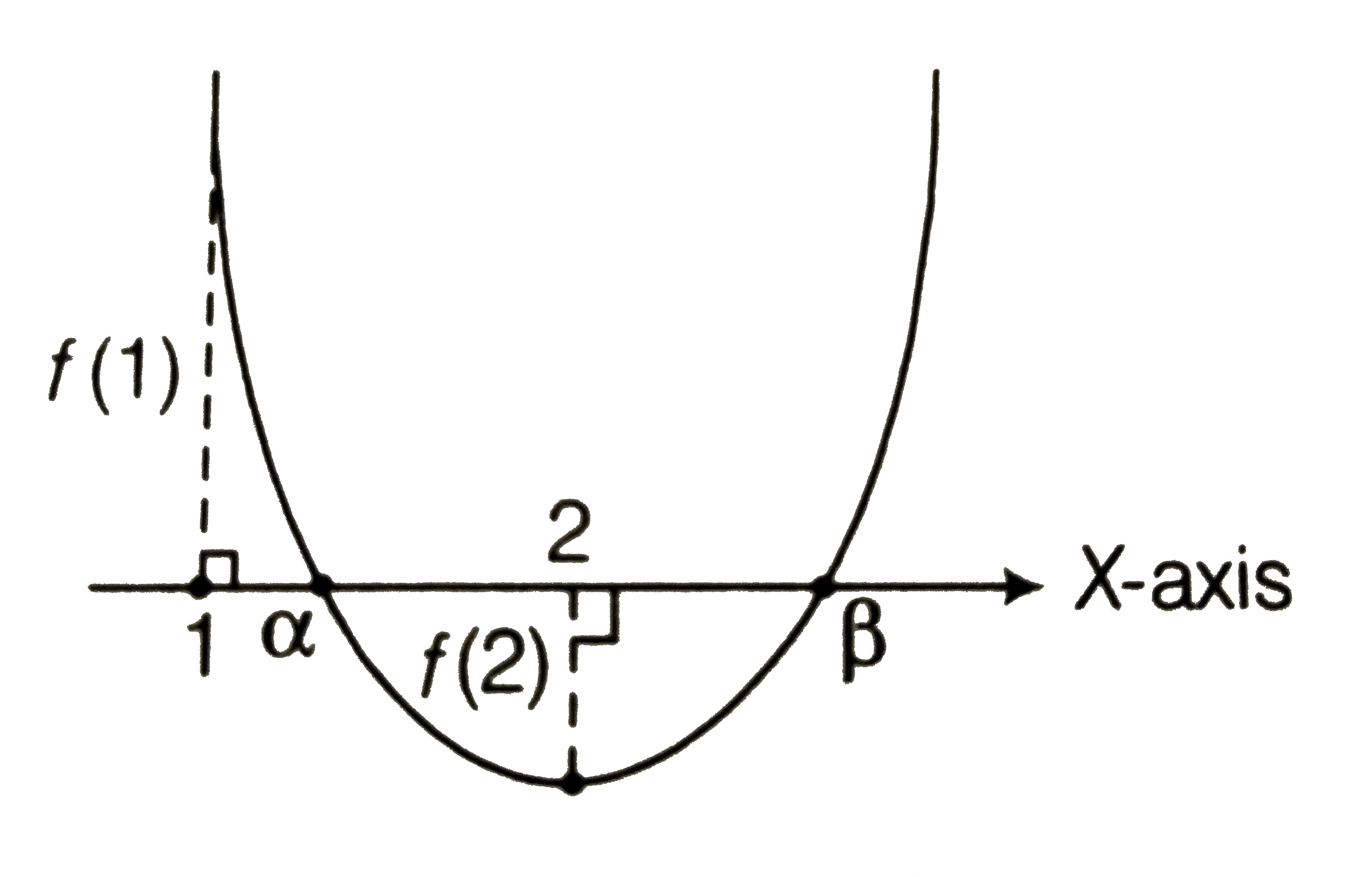
i.e.,
...i
....ii
On combining Eqs (i) and (ii) we get
(v) Both the roots lie in the interval (1,2)
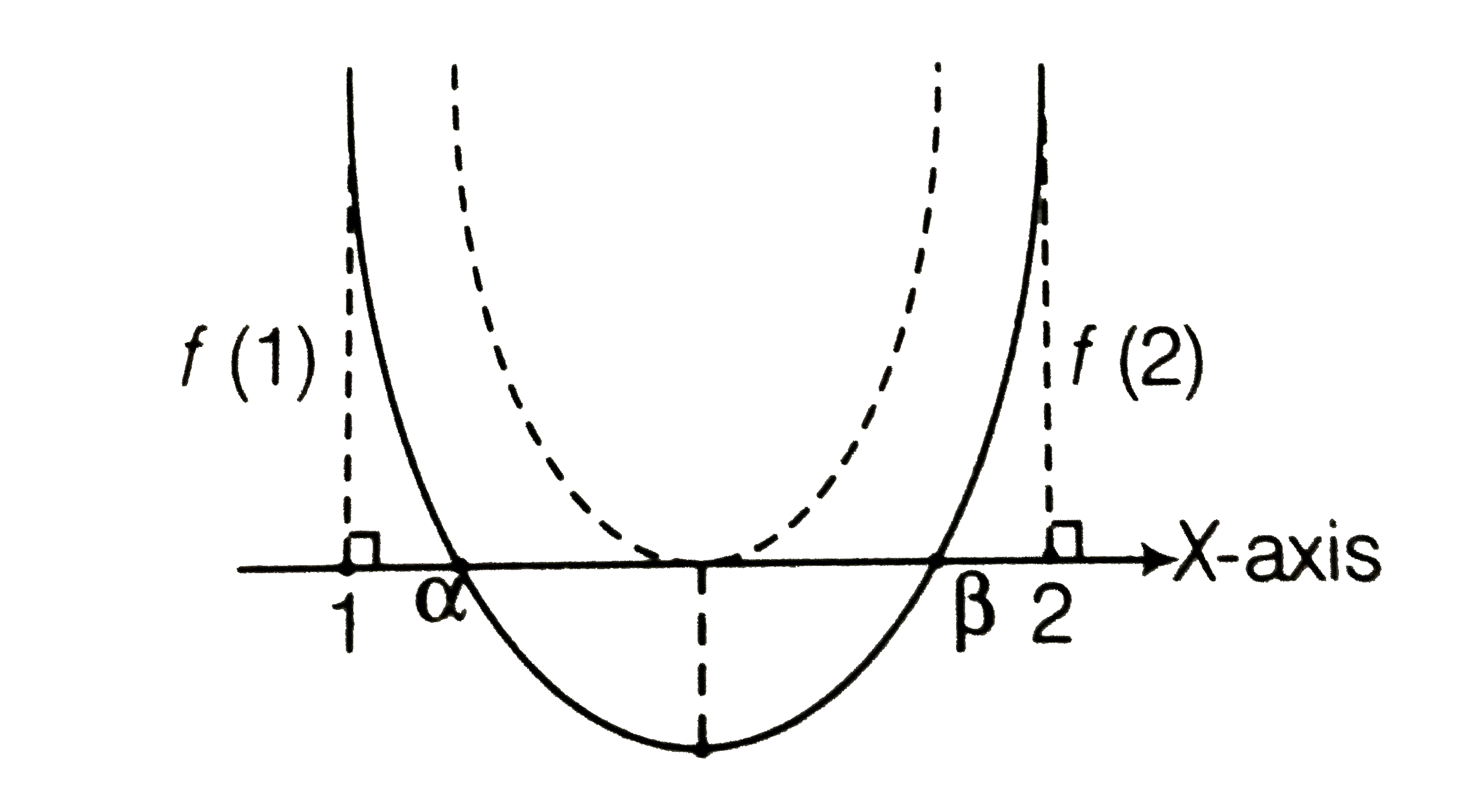
i.e.
...i
i.e.
.ii
i.e.
..iii
coordinate of vertex
i.e.
or
.iv
On combining Eqs. i,ii,iii and iv we get
(vi) One root is greater than 2 and the other root is smaller than
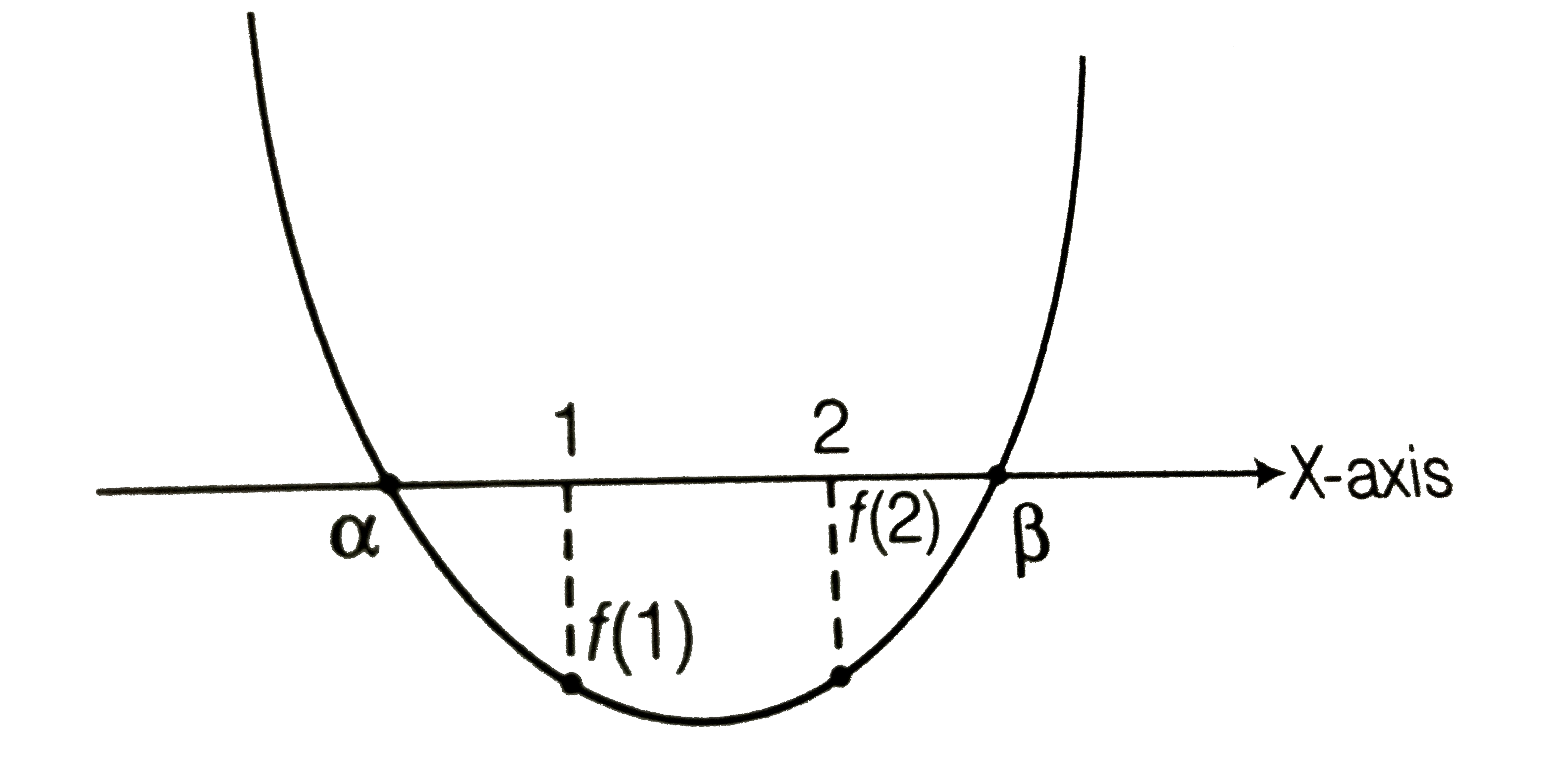
i.e.
.i
i.e. which is not possible.
Thus , no such exists.
(vii) At least one root lie in the interval (1,2)
Case I Exactly one root lies in (1,2)
[from (iv) part]
Case II Both roots lie in the interval (1,2).
(viii) Atleast one root is greater than 2
Case I One root is smaller than 2 and the other root is greater than 2.
Then, [from iii) part]
Case II Both the roots are greaters than 2 then .
Hence atleast one root is greater than 2.
or
Topper's Solved these Questions
- THEORY OF EQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise For Session 1
11 Videos
- THEORY OF EQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise For Session 2
10 Videos
- THE STRAIGHT LINESBOOK - ARIHANT MATHS ENGLISHCHAPTER - THE STRAIGHT LINESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
17 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHS ENGLISHCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Three Dimensional Coordinate System Exercise 12 : Question Asked in Previous Years Exam
2 Videos
Similar Questions
Let x2−2(a−1)x+a−1=0(a∈R) be a quadratic equation, then find the value of 'a' for which
(a) Both the roots are positive
(b)Both the roots are negative
(c) Both the roots are opposite in sign.
(d) Both the roots are greater than 1.
(e)Both the roots are smaller than 1.
(f) One root is small than 1 and the other root is greater than 1.
View SolutionFind the values of a for which one root of equation (a−5)x2−2ax+a−4=0 is smaller than 1 and the other greater than 2.
View SolutionLet x2−2(a−1)x+a−1=0(a∈R) be a quadratic equation, then find the values of "a" for which
(i) Exactly one root lies in (0,1)
(ii) Both roots lies in (0, 1).
(iii) Atleast one root lies in (0,1).
(iv) One root is greater than 1 and other root is smaller than 0.
View SolutionFind the values of m for which roots of equation x2−mx+1=0 are less than unity.
View SolutionLet x2−(m−3)x+m=0(m∈R) be a quadratic equation, then find the values of 'm' for which both
(a) the roots are greater than 2.
(b) both roots are positive,
( c) one root is positive and other is negative.
( d) One root is greater than 2 and other smaller than 1
( e) Roots are equal in magnitude and opposite in sign,
( f) both roots lie in the interval (1, 2)
View SolutionFind the values of the parameter a for which the roots of the quadratic equation x2+2(a−1)x+a+5=0 are
such that one root is greater than 3 and the other root is smaller than 1.
View SolutionIf a, p are the roots of the quadratic equation x2−2p(x−4)−15=0, then the set of values of p for which one root is less than 1 and the other root is greater than 2 is:
View SolutionLet 4x2−4(α−2)x+α−2=0(α∈R) be a quadratic equation. Find the values of 'a' for which
(i) Both roots are real and distinct.
(ii) Both roots are equal.
(iii) Both roots are imaginary
(iv) Both roots are opposite in sign.
(v) Both roots are equal in magnitude but opposite in sign
View SolutionThe values of a for which the equation 2x2−2(2a+1)x+a(a+1)=0 may have one root less them a and other root greater than a are given by
View SolutionIf both the roots of the equation 4x2−2x+m=0 lie in the interval (−1,1), then
View Solution
