Let y=f(x) be a curve passing through (4,3) such that slope of normal at any point lying in the first quadrant is negative and the normal and tangent at any point P cuts the Y-axis at A and B respectively such that the mid-point of AB is origin, then the number of solutions of y=f(x) and f=|5−|x∣∣, is
To solve the problem step by step, we need to analyze the given conditions and derive the necessary equations.
Step 1: Understanding the Problem
We have a curve y=f(x) that passes through the point (4,3). The slope of the normal at any point in the first quadrant is negative, which implies that the slope of the tangent dydx is positive. We also know that the normal and the tangent at any point P cut the y-axis at points A and B respectively, such that the midpoint of AB is the origin (0,0).
Step 2: Setting Up the Equations
Let the slope of the tangent at point P(x,y) be m=dydx. The equation of the tangent line at point P can be expressed as:
y−y1=m(x−x1)(1)
At the y-axis, where x=0:
y=mx+c1⟹y=c1(when x=0)
Thus, point A on the y-axis is (0,c1).
The equation of the normal line at point P is given by:
y−y1=−1m(x−x1)(2)
At the y-axis, where x=0:
y=−1m(0−x1)+y1⟹y=−x1m+y1(when x=0)
Thus, point B on the y-axis is (0,c2).
Step 3: Midpoint Condition
The midpoint of points A(0,c1) and B(0,c2) is given by:
(0,c1+c22)=(0,0)⟹c1+c2=0⟹c1=−c2(3)
Step 4: Relating Tangent and Normal
From equations (1) and (2), we can express c1 and c2:
- From (1): c1=y−mx
- From (2): c2=y+x1m−y1
Using the relationship c1=−c2:
y−mx=−(y+x1m−y1)
This leads to:
y−mx+y+x1m−y1=0
Rearranging gives us a quadratic equation in terms of m.
Step 5: Solving the Quadratic Equation
The quadratic equation derived from the previous step can be solved for m:
m2x−2ym−x=0
Using the quadratic formula:
m=2y±√(2y)2+4x2x
This gives us two possible slopes for the tangent.
Step 6: Finding the Function
Given that y=f(x) and f(x)=|5−|x||, we need to find the intersections of y=f(x) and y=x24−1.
Step 7: Finding Intersections
Set x24−1=|5−|x|| and solve for x. This will yield the points of intersection.
Step 8: Analyzing the Intersections
After solving the equations, we find that there are two points of intersection between the two curves.
Final Answer
Thus, the number of solutions for y=f(x) and f(x)=|5−|x|| is:
2
We have a curve y=f(x) that passes through the point (4,3). The slope of the normal at any point in the first quadrant is negative, which implies that the slope of the tangent dydx is positive. We also know that the normal and the tangent at any point P cut the y-axis at points A and B respectively, such that the midpoint of AB is the origin (0,0).
Let the slope of the tangent at point P(x,y) be m=dydx. The equation of the tangent line at point P can be expressed as:
y−y1=m(x−x1)(1)
At the y-axis, where x=0:
y=mx+c1⟹y=c1(when x=0)
Thus, point A on the y-axis is (0,c1).
y−y1=−1m(x−x1)(2)
At the y-axis, where x=0:
y=−1m(0−x1)+y1⟹y=−x1m+y1(when x=0)
Thus, point B on the y-axis is (0,c2).
The midpoint of points A(0,c1) and B(0,c2) is given by:
(0,c1+c22)=(0,0)⟹c1+c2=0⟹c1=−c2(3)
From equations (1) and (2), we can express c1 and c2:
- From (1): c1=y−mx
- From (2): c2=y+x1m−y1
y−mx=−(y+x1m−y1)
This leads to:
y−mx+y+x1m−y1=0
Rearranging gives us a quadratic equation in terms of m.
The quadratic equation derived from the previous step can be solved for m:
m2x−2ym−x=0
Using the quadratic formula:
m=2y±√(2y)2+4x2x
This gives us two possible slopes for the tangent.
Given that y=f(x) and f(x)=|5−|x||, we need to find the intersections of y=f(x) and y=x24−1.
Set x24−1=|5−|x|| and solve for x. This will yield the points of intersection.
After solving the equations, we find that there are two points of intersection between the two curves.
Thus, the number of solutions for y=f(x) and f(x)=|5−|x|| is:
2
Topper's Solved these Questions
- DIFFERENTIAL EQUATION Book:ARIHANT MATHS ENGLISHChapter:DIFFERENTIAL EQUATION Exercise:Solved Examples
Explore 1 Video
- DIFFERENTIAL EQUATION Book:ARIHANT MATHS ENGLISHChapter:DIFFERENTIAL EQUATION Exercise:Subjective Type Question
Explore 1 Video
- DETERMINANTS Book:ARIHANT MATHS ENGLISHChapter:DETERMINANTS Exercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 18 Videos
- DIFFERENTIATION Book:ARIHANT MATHS ENGLISHChapter:DIFFERENTIATION Exercise:Exercise For Session 10
Explore 4 Videos
Similar Questions
Let y=f(x)be curve passing through (1,√3) such that tangent at any point P on the curve lying in the first quadrant has positive slope and the tangent and the normal at the point P cut the x-axis at A and B respectively so that the mid-point of AB is origin. Find the differential equation of the curve and hence determine f(x).
View SolutionA and B are two points on the x-axis and y-axis respectively. P(2, -3) is the mid point of AB. Find the
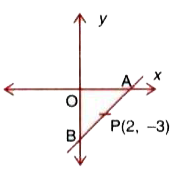
slope of line AB
View SolutionThe tangent at any point P on y2=4x meets x-axis at Q, then locus of mid point of PQ will be
View SolutionFind the equation of a curve passing through (1,1) and whose slope of tangent at a point (x, y) is −xy.
View SolutionThe slope of normal to the curve x3=8a2y,a>0 at a point in the first quadrant is −23 , then point is
View SolutionThe length of normal at any point to the curve, y=ccosh(xc) is
View SolutionFind the equation of a curve, passes through (−2,3) at which the slope of tangent at any point (x,y) is 2xy2.
View SolutionIf a curve passes through the point (1, -2) and has slope of the tangent at any point (x,y) on it as x2−2yx, then the curve also passes through the point
View SolutionFind the eqution of the curve passing through the point (1,1), if the tangent drawn at any point P(x,y) on the curve meets the coordinate axes at A and B such that P is the mid point of AB.
View SolutionA family of curves is such that the slope of normal at any point (x, y) is 2(1-y).
The orthogonal trajectories of the given family of curves is
View Solution