Lists I, II and III contains conics, equation of tangents to the conics and points of contact, respectively.

For if a tangent is drawn to a suitable conic (List I) at the point of contact , which of the following options is the only CORRECT combination for obtaining its equation?
(II) (ii) (Q)
(III) (i) (P)
(I) (i) (P)
(I) (ii) (Q)
The correct Answer is:D
For and point on the curve, the only possible curve is
Equation of tangent is and point of contact is .
Topper's Solved these Questions
- HYPERBOLABook:CENGAGE ENGLISHChapter:HYPERBOLAExercise:NUMERICAL VALUE TYPE
Explore 14 Videos
- HYPERBOLABook:CENGAGE ENGLISHChapter:HYPERBOLAExercise:JEE MAIN
Explore 3 Videos
- HYPERBOLABook:CENGAGE ENGLISHChapter:HYPERBOLAExercise:COMOREHENSION TYPE
Explore 21 Videos
- HIGHT AND DISTANCE Book:CENGAGE ENGLISHChapter:HIGHT AND DISTANCE Exercise:Archives
Explore 3 Videos
- INDEFINITE INTEGRATIONBook:CENGAGE ENGLISHChapter:INDEFINITE INTEGRATIONExercise:Multiple Correct Answer Type
Explore 2 Videos
Similar Questions
Matching the information given in the three columns of the following table column 1, 2 and 3 contain conics, equations of tangents in the conics and points of contact, respectively.
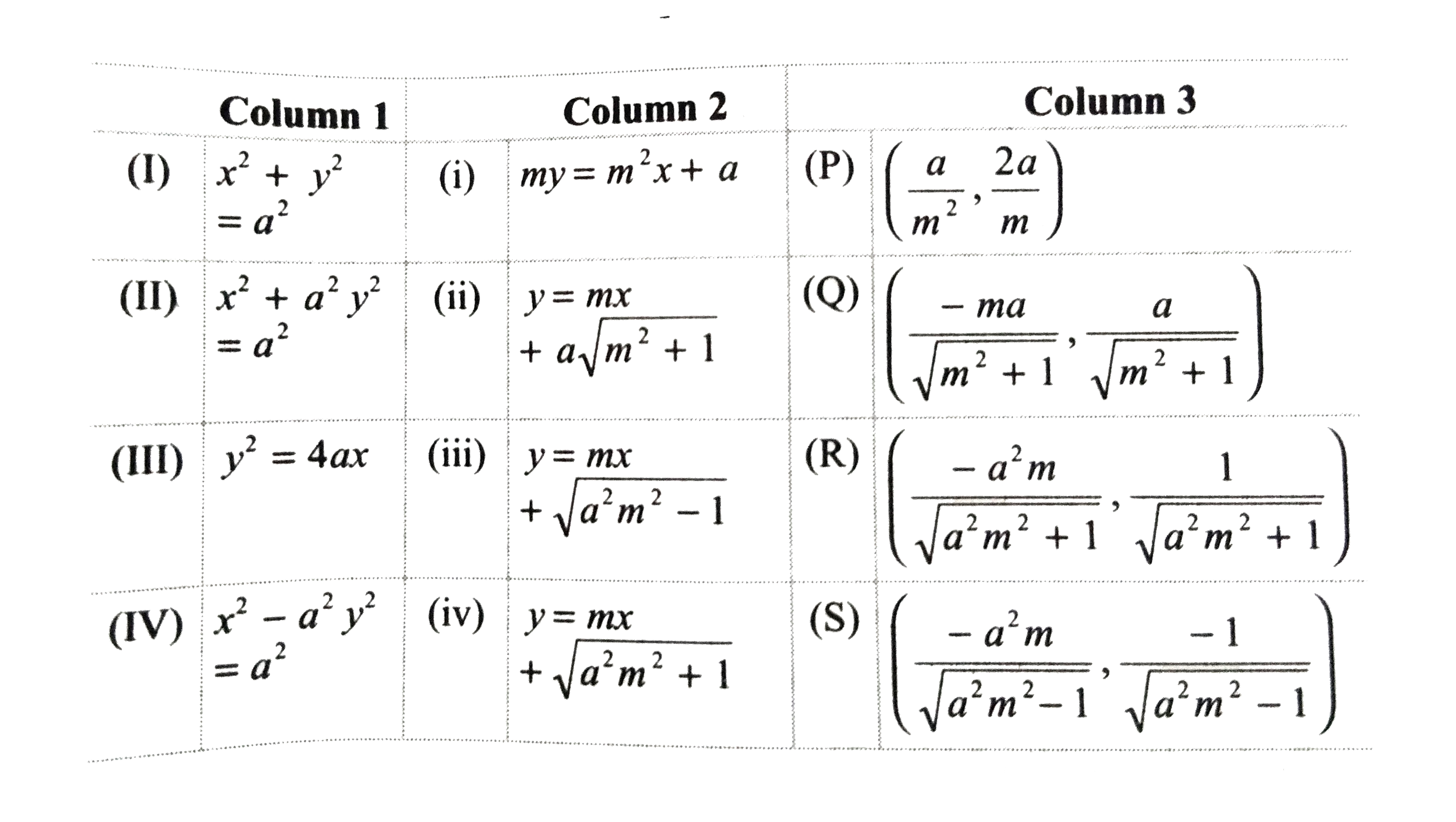
Q. For a=√2), if a tangent is drawn to a suitable conic (Column 1) at the points of contact (-1, 1), then which of the following options is the only correct combination for obtaining its equation?
View SolutionMatching the information given in the three columns of the following table column 1, 2 and 3 contain conics, equations of tangents in the conics and points of contact, respectively.
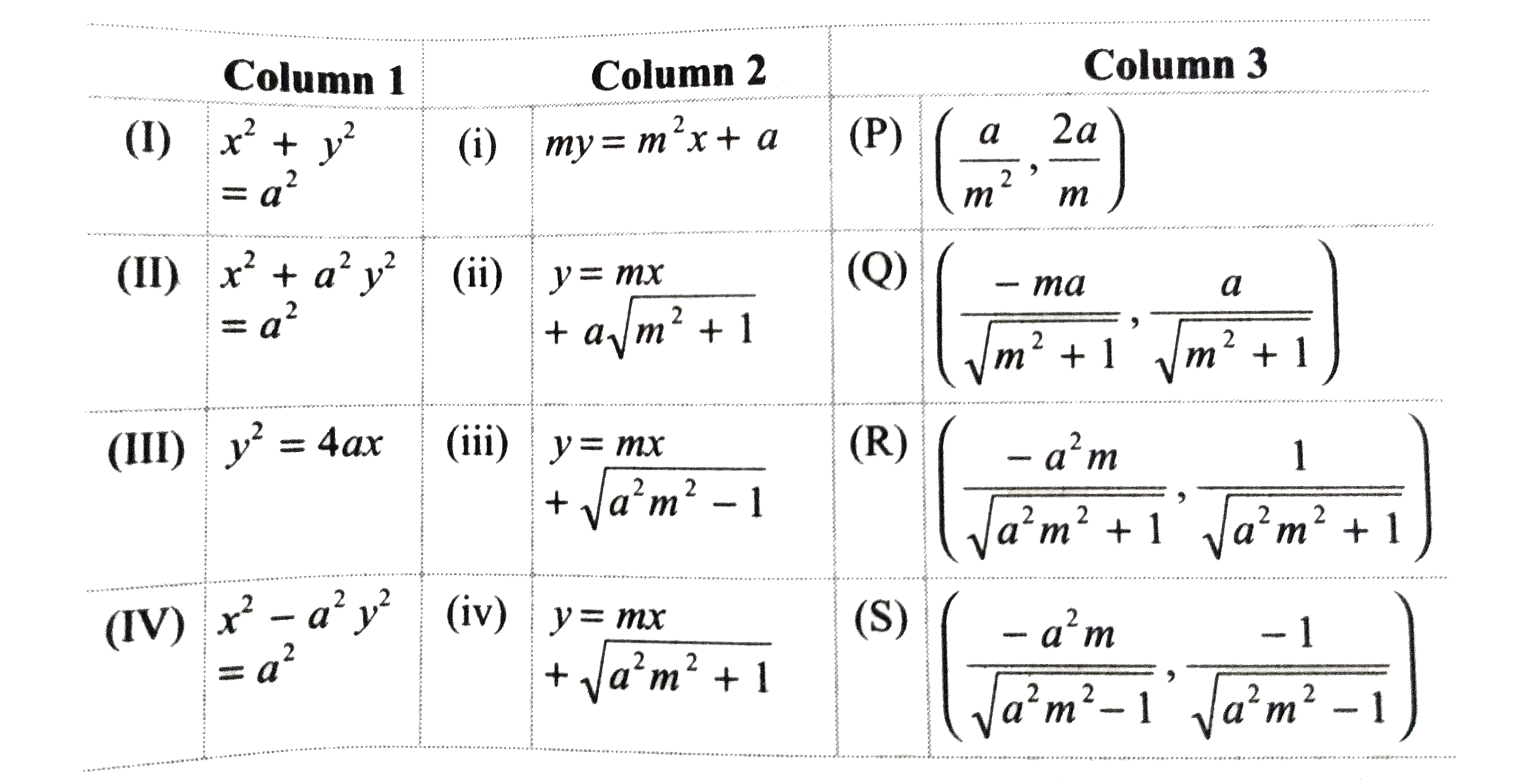
Q. The tangent to a suitable conic (column 1) at (√3,12) is found to be √3x+2y=4, then which of the following options is the correct combination?
View Solution
Knowledge Check
Lists I, II and III contains conics, equation of tangents to the conics and points of contact, respectively.

If a tangent to a suitable conic (List I) is fond to be y = x+8 and its point of contact is (8, 16), then which of the following options I sthe only CORRECT combination?A(II) (i) (P)B(III) (i0) (Q)C(II) (iv) (R)D(I) (ii) (Q)Lists I, II and III contains conics, equation of tangents to the conics and points of contact, respectively.

If the tangent to a suitable conic (List I) at (√312) is found to be √3x+2y=4. then which of the following options is the only CORRECT combination?A(II) (iii) (R)B(IV) (iv) (S)C(IV) (iii) (S)D(II) (iv) (R)
Column 1,2 and 3 contains conics, equations of tangents to the conics and points of contact, respectively. Column I, Column 2, Column 3 I, x2+y2=a , (i), my=m2x+a , (P), (am2,2am) II, x2+a2y2=a , (ii), y=mx+a√m2+1 , (Q), (−ma√m2+1,a√m2+1) III, y2=4ax , (iii), y=mx+√a2m2−1 , (R), (−a2m√a2m2+1,1√a2m2+1) IV, x2−a2y2=a2 , (iv), y=mx+√a2m2+1 , (S), (−a2m√a2m2+1,−1√a2m2+1) For a=√2,if a tangent is drawn to a suitable conic (Column 1) at the point of contact (−1,1), then which of the following options is the only CORRECT combination for obtaining its equation? (I) (ii) (Q) (b) (III) (i) (P) (II) (ii) (Q) (d) (I)(i)(P)
View SolutionFind the equation of tangent to the conic x2−y2−8x+2y+11=0 at (2,1)
View SolutionFind the equation of tangent to the conic x2−y2−8x+2y+11=0 at (2,1).
View SolutionFind the equation of tangent to the conic x2−y2−8x+2y+11=0 at (2,1)
View SolutionLet the midpoint of the chord of contact of tangents drawn from A to the circle x2+y2=4 be M(1,−1) and the points of contact be B and C

View SolutionLet the midpoint of the chord of contact of tangents drawn from A to the circle x2+y2=4 be M(1,−1) and the points of contact be B and C

View Solution
