Match the following

Text Solution
The correct Answer is:N/a
Let the coordinate of point P(x1,y1) on the line x+5y=13i.e.,
P(13−5y1,y1).
∴ Distance of P from the line 12x-5y+26=0,
2=∣∣∣21(13−5y1)−5y1+26√144+25∣∣∣
⇒2=±156−60y1−5y1+2613
⇒−65y1=−156[taking positive sign]
⇒y1=15665=125
,'x1=13−5y1
=13−12=1
So, the coordinate of is P(1,125).
Similarly, the coordinates of Q are (−3,165)[taking negaive sign]
(ii) Let coordinates of the point on the line x+y=4 be (4−y1,y1).
Distance from the line 4x+3y−10=0
1=∣∣∣4(−4y1)+3y1−10√16+9∣∣∣
⇒1=±16−4y1+3y1−105 [taking negative sign]
⇒5=6−y1
⇒y1=1
If y1=1, then x1=3
So, the point is (3,1)
Similarly, taking negative sign the point is (-7,11)
(iii) Given point A(-2,5) and B(3,1)
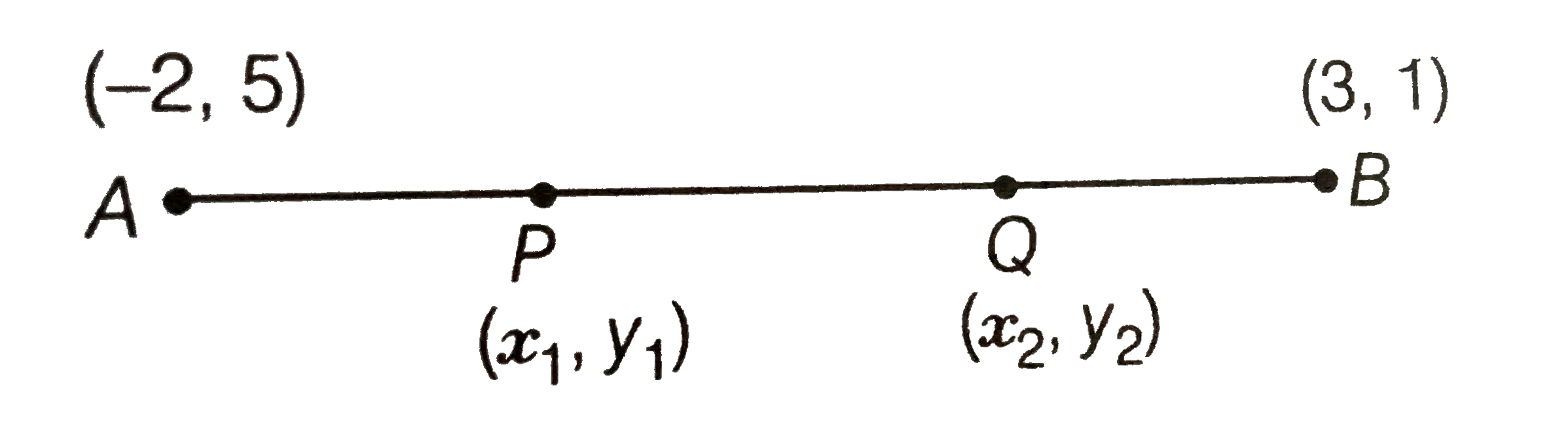
Now, the point P divides line joining the point A and B in 1:2.
∵x1=1.3+2(−2)1+2=3−43=−13
and y1=1.1+2.51+2=113
So, the coordinates of P are (−13,113).
Thus, the point Q divided the line joining A to B in 2:1.
∵x2=2.3+1(−2)2+1=43
and y2=2.1+1.52+1=73
Hence, the coordinates of Q are (43,73).
Hence, the correct matches are (i) → (c ), (ii) → (a), (iii) → (b).
|
Updated on:21/07/2023
