Match the following lists:

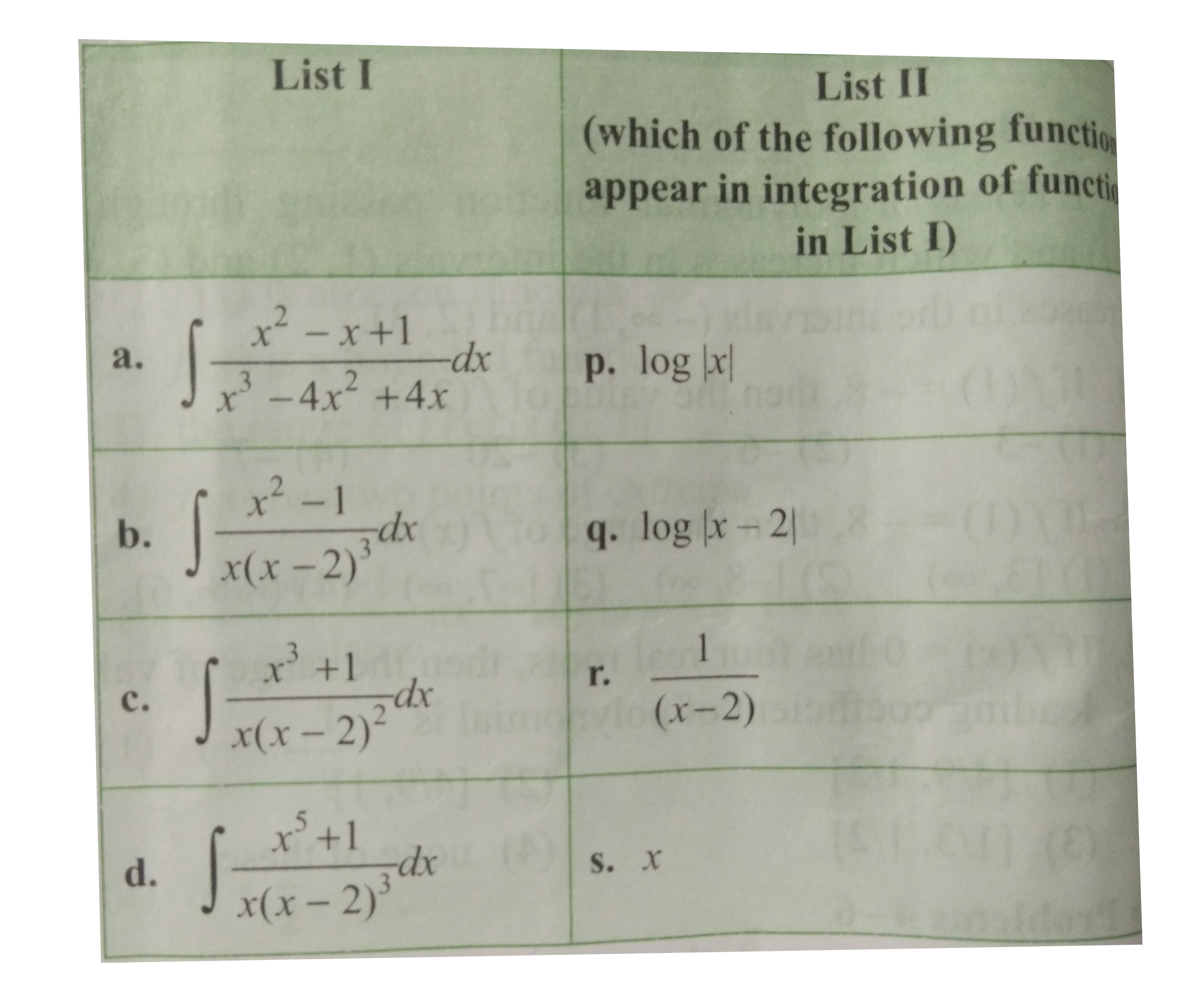
Text Solution

Verified by Experts

(a) Tangent to the ellipe at $P (ϕ)$ is
$\frac{x}{4} \cos ϕ + \frac{y}{2} \sin ϕ = 1$
It must pass through the center of the circle. Hence,
$\frac{4}{4} \cos ϕ + \frac{2}{2} \sin ϕ = 1$
$or \cos ϕ + \sin ϕ = 1$
or $1 + \sin 2 ϕ ϕ = 1$
or $\sin 2 ϕ = 0$
i.e., $2 ϕ = 0 or π$
i.e., $\frac{ϕ}{2} = 0 or \frac{π}{4}$
(b) Consider and point $P (\sqrt{6} \cos, θ, \sqrt{2} \sin θ)$ on the ellipe $\frac{x^{2}}{6} + \frac{y^{2}}{2} = 1$
Given thast P=2. Therefore, $6 \cos^{2} θ + 2 \sin^{2} θ = 0$
or $4 \cos^{2} θ = 2$
or $\cos θ = \pm \frac{1}{\sqrt{2}}$
i.e., $θ = \frac{π}{4} or \frac{5 π}{4}$
(c) Sovling the equation of ellipse and parbola (eliminating $(x^{2}$ )n we have
$y - 1 + 4 y^{2} = 4$
or $4 y^{2} + y - 5 = 0$
$(4 y + 5) (y - 1) = 0$
or y=1, x=0 $ T h e c u r v e s \to u c h a t (0, 1) s o, t h e ∠ o f \int e r \sec t i o n i s 0 . (d) T h e ∥ a ∥ l a t$ P(a cos theta, b sin theta) $i s $ (ax)/(cos thet)-(hx)/(sin theta)=a^(2)-b^(2) $ W h e r e$ a^(2)=14,b^(2)=5 $ I t m e a n s t h e c r u v e a g a \in a t Q$ (2 theta) $, i . e .,$ (a cos 2 theta, b sin 2theta) $ H e c e n, $ (a)/(costheta)a cos 2theta-(b)/(sin theta)(b 2sintheta)=a^(2)-b^(2) $$ or (14)/(cos theta) cos 2theta-(5)/(sin theta)(sin 2 theta)=14-5 $ or$ 28 cos^(2)theta-14-10 sec^(2)theta=9 cos theta $$ or 18 cos^(2)theta-9cos theta-14=0 $$ or (6 cos theta-7)(3 cos theta+2) $ or$ cos theta=-(2)/(3)

Updated on:21/07/2023