Maximise Z=5x+11y
Subject to x+4y≤30,5x+2y≥60and x≥0,y≥0.Solution in Odia
Step by step video & image solution for Maximise Z=5x+11y Subject to x+4yle30,5x+2yge60and xge0,yge0. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- LINEAR PROGRAMMINGBOOK - ARIHANT PRAKASHANCHAPTER - LINEAR PROGRAMMINGEXERCISE - Chapter Test (1 MARK Questions)
7 Videos
- LINEAR PROGRAMMINGBOOK - ARIHANT PRAKASHANCHAPTER - LINEAR PROGRAMMINGEXERCISE - Chapter Test (4 MARKS Questions)
6 Videos
- LINEAR PROGRAMMINGBOOK - ARIHANT PRAKASHANCHAPTER - LINEAR PROGRAMMINGEXERCISE - PRACTICE QUESTIONS (Exams., Textbook.s & Other Imp. Questions) (4 Marks Questions)
9 Videos
- INVERSE TRIGONOMETRIC FUNCTIONS BOOK - ARIHANT PRAKASHANCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONS EXERCISE - Chapter Test (6 MARKS Questions)
3 Videos
- MATRICESBOOK - ARIHANT PRAKASHANCHAPTER - MATRICESEXERCISE - Chapter Test ( 6 Mark Questions)
4 Videos
Similar Questions
Draw the following convex polygons on the graph paper and indicate it by shading and mention the vertices.
x+y≤5,x+y≥2,x,y≥0
View SolutionFind the feasible region of x−2y≥0,3x−6y≤0,x,y≥0.
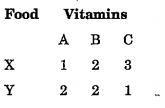
View SolutionTwo types of food X and Y re mixed to prepare a mixture is such a way that the mixture contains at least 10 units of vitamin A, 12 units of vitamin B and 8 units of vitamin X. These vitamines are avialalbe in 1kg of food as per table below. 1kg of food X costs Rs 16 and 1kg of food Y costs Rs 20. Formulate the L. P. P. so as to determine the least cost of the mixture containing the required amound of vitamins.
View SolutionIf sin−1(xa)+sin−1(yb)=αprove thatx2a2+2xyabcosα+y2b2=sin2α
View SolutionThere are two families A and B. There are 4 men, 6 women and 2 children in family A and 2 men, 2 women and 4 children in family B. The recommended daily amount of calories is 2400 for men, 1900 for women and 1800 for children, and 45 g of proteins for men, 55 g for women and 33 g for children. Represent the above information by matrices. Using matrices multiplication, calculate the total requirement of calories and proteins for each of the 2 families.
View SolutionMaximise Z=3x+4y
Subject to the constraints
x+y≤4,x≥0,y≥0
View SolutionMaximise Z=5x+3y
Subject to 3x+5y≤15,5x+2y≤10,x≥0,y≥0.
View SolutionMaximise Z=3x+4y Subject to the constraints x+yle4, xge0, yge0
View SolutionMaximise Z=5x+3y subject to 3x+5yle15, 5x+2yle10, xge0, yge0
View SolutionMaximise Z=3x+4y
Subject to the constraints x+y≤4,x≥0,y≥0
View SolutionMaximise Z=5x+3y
subject to 3x+5y≤15,5x+2y≤10,x≥0,y≥0
View SolutionMaximise Z=3x+4y
Subject to the constraints x+y≤4,x≥0,y≥0
View SolutionSolve the following LPP Graphically,
Maximise, Z=60x+15y
Subject to constriants,
x+y≤50,3x+y≤90,x≥0,y≥0
View SolutionSolve graphically.
Maximise Z=5x+3y
Subject to the constraints
3x+5y≤15,5x+2y≤10,x≥0,y≥0
View SolutionMaximise: Z=600x+400y
Subject to the constraints:
x+2y≤12,2x+y≤12
4x+5y≥20,x≥0,y≥0
Solve the LPP?
View Solution
