Normal to the ellipse x284+y249=1 intersects the major and minor axes at PandQ , respectively. Find the locus of the point dividing segment PQ in the ratio 2:1.Solution in Bengali
The equation of normal is 8xsecthea−7y=15
It meets the exes at
P(158cosθ,0)andQ(0,−157sinθ)
Let M(h,k) divides PQ in ratio 2:1 Thefefore,
3h=158cosθ,3k=−307sinθ
∴Cosθ=8h5,sinθ=−7k10
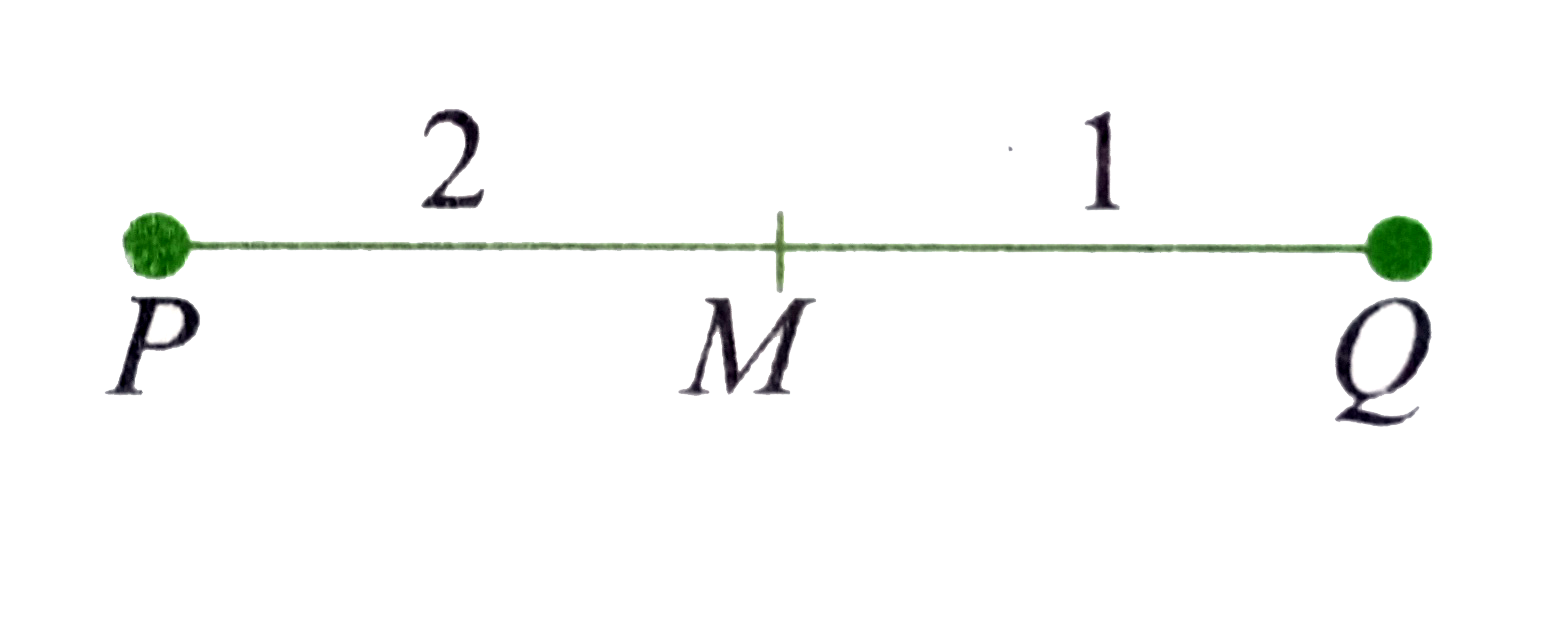
Eliminating θm we get the locus as
64x225+49y2100=1
Similar Questions
A tangent having slope of −43 to the ellipse x218+y232=1 intersects the major and minor axes at points A and B, respectively. If C is the center of the ellipse, then find area of triangle ABC.
View SolutionIf normal at any poin P on the ellipse x2a2+y2b2=1(a>b>0) meets the major and minor axes at Q and R, respectively, so that 3PQ=2PR, then find the eccentricity of ellipse
View SolutionLet P be a point on x2=4y. The segment joining A(0,−1) and P is divided by point Q in the ratio 1:2, then locus of point Q is
View SolutionIf a normal at any point on the ellipse x2a2+y2b2=1(a>b)meet the major and minor axes at Mand N respectively, such that PMPN=23, then the eccentricity =
View SolutionNormal to the ellipse x264+y249=1 intersects the major and minor axes at PandQ , respectively. Find the locus of the point dividing segment PQ in the ratio 2:1.
View SolutionIf normal at any point P on the ellipse (x^(2))/(a^(2))+(y^(2))/(b^(2))=1 (agtbgt0) meets the major and minor axes at Q and R, respectively, so that 3PQ=2PR, then find the eccentricity of ellipse
View SolutionNormal to the ellipse (x^2)/(64)+(y^2)/(49)=1 intersects the major and minor axes at Pa n dQ , respectively. Find the locus of the point dividing segment P Q in the ratio 2:1.
View SolutionA tangent having slope of -4/3 to the ellipse (x^2)/(18)+(y^2)/(32)=1 intersects the major and minor axes at points A and B , respectively. If C is the center of the ellipse, then find area of triangle A B Cdot
View Solution
