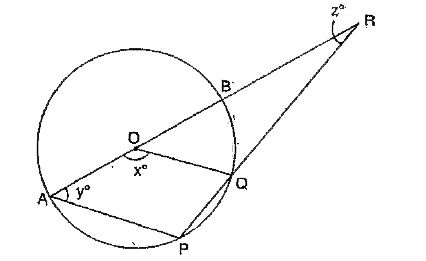
O is the centre of the circle APQB, AOBR, PQR are straight lines. Find x in terms of y and z.
A
x=y+z
B
x=2y+z
C
x=y+2z
D
x=2(y+z)
Video Solution
Text Solution
The correct Answer is:D
|
Answer
Step by step video, text & image solution for O is the centre of the circle APQB, AOBR, PQR are straight lines. Find x in terms of y and z. by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLES BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - CIRCLES EXERCISE - UNIT TEST
20 Videos
- CIRCLES BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - CIRCLES EXERCISE - QUESTION BANK
25 Videos
- AVERAGE BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - AVERAGE EXERCISE - UNIT TEST -3
20 Videos
- CIRCUMFERENCE AND AREA OF A CIRCLE BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - CIRCUMFERENCE AND AREA OF A CIRCLE EXERCISE - SELF ASSESSMENT SHEET - 22
10 Videos
Similar Questions
Knowledge Check
O is the centre of the circle, ABN is a straight line. Find ∠AOC.
 A128∘B132∘C130∘D135∘
A128∘B132∘C130∘D135∘O is the centre of the circle x and y respectively equal.
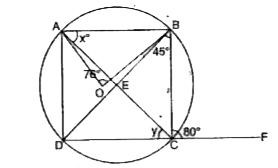 A38∘,45∘B35∘,62∘C62∘,35∘D46∘,38∘
A38∘,45∘B35∘,62∘C62∘,35∘D46∘,38∘In the given figure, O is the centre of the circle, ∠ACB=54∘ and BCF is a straight line. Find x.
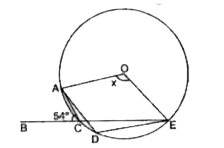 A126∘B54∘C108∘D90∘
A126∘B54∘C108∘D90∘
O is the centre of the circle ∠BCO=30∘ then find x and y
View SolutionIn the given figure ABCD is a cyclic quadrilateral and O is the centre of the circle. If ∠CBQ=60∘ and x=2y, find the values of x,y and z Also find ∠AOC
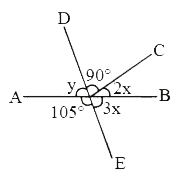
View SolutionIn Figure, O is the centre of the circle, prove that ∠x=∠y+∠z
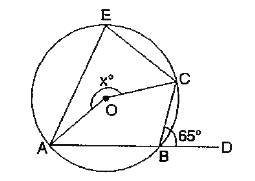
View SolutionIn the given figure, O is the centre of the circle. ABD is a straight line and ∠CBD=65∘. Find reflex ∠AOC (marked x∘).
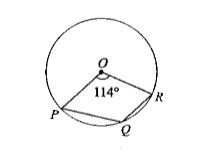
View SolutionIn the figure, O is the centre of the circle . ∠PQR is equal to
View Solution