On a square cardboard sheet of area 784cm2 , four congruent circular plates of maximum size are placed such that each circular plate touches the other two plates and each side of the square sheet is tangent to two circular plates. Find the area of the square not covered by the circular plates.
To solve the problem step by step, we will follow these calculations:
Step 1: Find the side length of the square cardboard sheet.
Given the area of the square cardboard sheet is 784cm2.
The formula for the area of a square is:
Area=side2
Let the side length be A. Therefore:
A2=784
To find A, take the square root of both sides:
A=√784=28cm
Step 2: Determine the diameter and radius of the circular plates.
Since there are four congruent circular plates placed such that each plate touches the other two plates and each side of the square is tangent to two circular plates, the arrangement can be visualized as follows:
- The diameter of each circular plate will be equal to half the side length of the square.
Diameter=282=14cm
Now, to find the radius r:
r=Diameter2=142=7cm
Step 3: Calculate the area of one circular plate.
The area Ac of one circular plate can be calculated using the formula:
Ac=πr2
Substituting the value of r:
Ac=π(72)=π(49)
Using π≈227:
Ac=227×49=154cm2
Step 4: Calculate the total area of the four circular plates.
Since there are four circular plates, the total area Atotal covered by the plates is:
Atotal=4×Ac=4×154=616cm2
Step 5: Find the area of the square not covered by the circular plates.
To find the area of the square that is not covered by the circular plates, subtract the total area of the circular plates from the area of the square:
Area not covered=Area of square−Atotal
Substituting the values:
Area not covered=784−616=168cm2
Final Answer:
The area of the square not covered by the circular plates is 168cm2.
---
Given the area of the square cardboard sheet is 784cm2.
Area=side2
Let the side length be A. Therefore:
A2=784
To find A, take the square root of both sides:
A=√784=28cm
Since there are four congruent circular plates placed such that each plate touches the other two plates and each side of the square is tangent to two circular plates, the arrangement can be visualized as follows:
Diameter=282=14cm
Now, to find the radius r:
r=Diameter2=142=7cm
The area Ac of one circular plate can be calculated using the formula:
Ac=πr2
Substituting the value of r:
Ac=π(72)=π(49)
Using π≈227:
Ac=227×49=154cm2
Since there are four circular plates, the total area Atotal covered by the plates is:
Atotal=4×Ac=4×154=616cm2
To find the area of the square that is not covered by the circular plates, subtract the total area of the circular plates from the area of the square:
Area not covered=Area of square−Atotal
Substituting the values:
Area not covered=784−616=168cm2
The area of the square not covered by the circular plates is 168cm2.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Four circles of equal radii are inscribed in a square of side 56 cm, touching each other such that each side of the square is tangent to two adjacent circles. The area of the square unoccupied by the circles is
A672B505C670D680A circular metal plate of radius 10 cm is given a charge of 20μC. The outward pull on the plate in the vacuum is
A560 NB370 NC360 ND630 NThere is a circularr hole in a square copper plate. If the plate is heated then the radius of the circular hole will
AincreaseBdecreaseCremains sameDincrease or decrease dependeing on size of the square plate
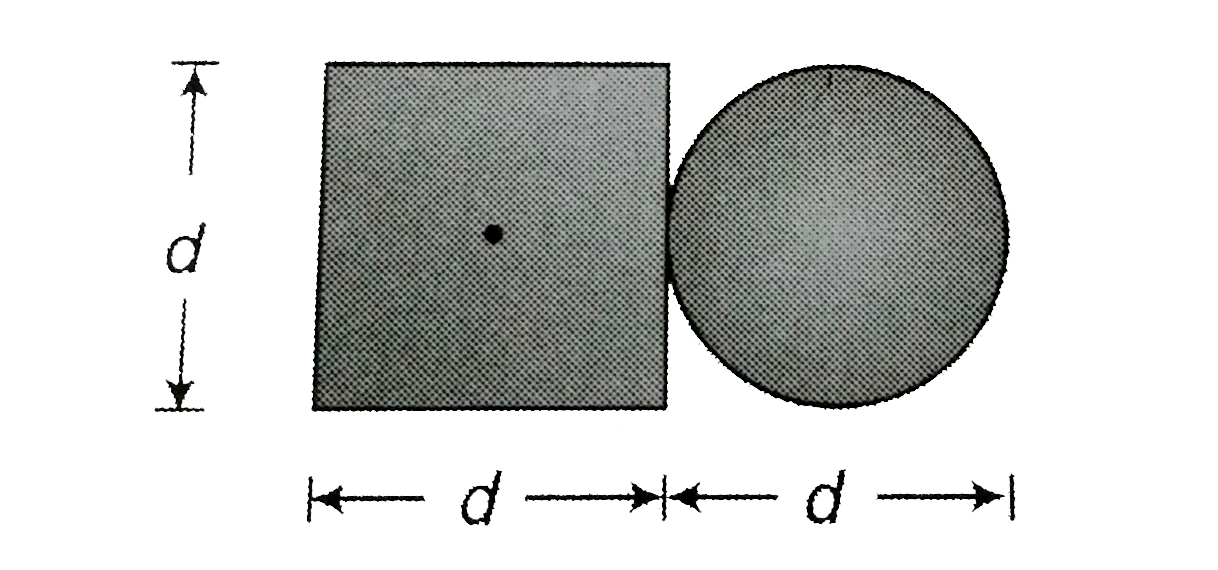
Two plates made of the same material and thickness are joined as shown. One plate is circular and another square in shape. The diameter of circular plate is equal to the side of the square plane. Locate c.m.
View Solution50 circular plates, each of radius 7cm and thickness 0.5cm are placed one above the other to form a solid right circular cylinder. Find the total surface area and the volume of the cylinder so formed.
View SolutionThere are two circular flower beds on two sides of a square lawn ABCD of side 56 m. If the centre of each circular flower bed is the point of intersection of the diagonals of the square lawn, find the sum of the areas of the lawn and the flower beds.
View SolutionConsider a circular plate of radius 5 cm placed on the surface of a water body such that their planes are parallel. Force necessary to pull the circular plate from water surface for which surface tension is 75dynecm−1 is:
View SolutionTwo equal maximum sized circular plates are cut off from a circular paper sheet of circumference 352 cm. Then the circumference of each circular plat is
View Solution
