P is the solution set of 7x−2>4x+1andQ is the solution set of 9x−45≥5(x−5), where x∈R. Represent :
P - Q
To solve the problem, we need to find the solution sets P and Q for the given inequalities and then represent P−Q.
Step 1: Solve for \( P \)
We start with the inequality:
\(
7x - 2 > 4x + 1
\)
Rearranging the inequality:
1. Move \( 4x \) to the left side:
\(
7x - 4x > 1 + 2
\)
2. Simplifying gives:
\(
3x > 3
\)
Dividing both sides by 3:
\(
x > 1
\)
Thus, the solution set \( P \) is:
\(
P: \{ x \in \mathbb{R} \mid x > 1 \}
\)
Step 2: Solve for \( Q \)
Now we solve the second inequality:
\(
9x - 45 \geq 5(x - 5)
\)
Expanding the right side:
\(
9x - 45 \geq 5x - 25
\)
Rearranging the inequality:
1. Move \( 5x \) to the left side:
\(
9x - 5x \geq -25 + 45
\)
2. Simplifying gives:
\(
4x \geq 20
\)
Dividing both sides by 4:
\(
x \geq 5
\)
Thus, the solution set \( Q \) is:
\(
Q: \{ x \in \mathbb{R} \mid x \geq 5 \}
\)
Step 3: Find \( P - Q \)
Now we need to find \( P - Q \), which represents the values in \( P \) that are not in \( Q \).
From the solution sets:
- \( P: x > 1 \)
- \( Q: x \geq 5 \)
Finding \( P - Q \):
- The values of \( P \) are all real numbers greater than 1.
- The values of \( Q \) are all real numbers greater than or equal to 5.
Thus, \( P - Q \) will be the interval:
\(
P - Q: \{ x \in \mathbb{R} \mid 1 < x < 5 \}
\)
Step 4: Represent \( P - Q \) on a Number Line
To represent \( P - Q \) on a number line:
- We will mark the points 1 and 5.
- Since the inequality is strict (not including 5), we will use open circles at 1 and 5.
Number Line Representation:
<−−−⊢−⊢−⊢−⊢−⊢−⊢−⊢−⊢−⊢→−101234567(−−−−−−−−−−−−−−−−−)
Where the parentheses indicate that 1 and 5 are not included in the solution set.
\(
7x - 2 > 4x + 1
\)
1. Move \( 4x \) to the left side:
\(
7x - 4x > 1 + 2
\)
2. Simplifying gives:
\(
3x > 3
\)
\(
x > 1
\)
\(
P: \{ x \in \mathbb{R} \mid x > 1 \}
\)
\(
9x - 45 \geq 5(x - 5)
\)
\(
9x - 45 \geq 5x - 25
\)
1. Move \( 5x \) to the left side:
\(
9x - 5x \geq -25 + 45
\)
2. Simplifying gives:
\(
4x \geq 20
\)
\(
x \geq 5
\)
\(
Q: \{ x \in \mathbb{R} \mid x \geq 5 \}
\)
- \( P: x > 1 \)
- \( Q: x \geq 5 \)
- The values of \( P \) are all real numbers greater than 1.
- The values of \( Q \) are all real numbers greater than or equal to 5.
\(
P - Q: \{ x \in \mathbb{R} \mid 1 < x < 5 \}
\)
- We will mark the points 1 and 5.
- Since the inequality is strict (not including 5), we will use open circles at 1 and 5.
<−−−⊢−⊢−⊢−⊢−⊢−⊢−⊢−⊢−⊢→−101234567(−−−−−−−−−−−−−−−−−)
Where the parentheses indicate that 1 and 5 are not included in the solution set.
Topper's Solved these Questions
- LINEAR INEQUATIONSBOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - QUESTIONS
22 Videos
- LINEAR INEQUATIONSBOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - Multiple Choice Questions
47 Videos
- LINEAR INEQUATIONSBOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - Competency Based Questions
15 Videos
- HEIGHTS AND DISTANCES BOOK - ICSECHAPTER - HEIGHTS AND DISTANCES EXERCISE - Exercise 22 C
28 Videos
- LOCI (LOCUS AND ITS CONSTRUCTIONS)BOOK - ICSECHAPTER - LOCI (LOCUS AND ITS CONSTRUCTIONS)EXERCISE - EXERCISE 16(B)
49 Videos
Similar Questions
Knowledge Check
The solution set of |x−1|x>2 is
A0<x<13Bx<13Cx>13D13<x<1If 1x−1≥2 , then the solution set is
AA. (1,32]BB. [1,32]CC. (1,32)DD. (−∞,−3]∪[12,∞)The solution set of 1≥15−7x>2x−27,x∈N on the number line is
A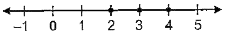 B
B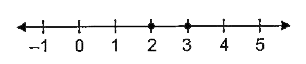 C
C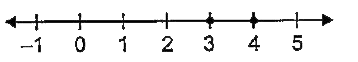 D
D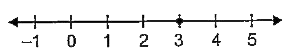
P is solution set of :7x−2>4x+1andQ is the solution set of : 9x−45≥5(x−5): where x∈R.
Represent (i) P - Q and P∩Q on different number lines.
View SolutionSolve and graph the solution set of 3x + 6 ≥9and−5x>−15, where x∈R.
View SolutionFind the solution set of 2x−7<8,x∈N. Represent the solution set on the number line.
View SolutionSolve and graph the solution set of −2<2x−6or−2x+5≥13. Where x∈R.
View SolutionSolve and graph the solution set of :
x+5≥4(x−1)and3−2x<−7,x∈R.
View Solution
