P(a,5a) and Q(4a,a) are two points. Two circles are drawn through these points touching the axis of y.
Centre of these circles are at
(a,a),(2a,3a)
(205a18,29a3),(5a2,3a)
(3a,29a3),(205a9,29a18)
None of these
The correct Answer is:B
Equation of any circle through the given points is:
(x−a)(x−4a)+(y−5a)(y−a)+λ(4x+3y−19a)=0, for some λ∈R.
As it touches the y-axis, putting x=0 and comparing discriminant of resulting quadratic equation in x to 0, we get
∴(−3a+3λ2)2=9a2−19λa
∴λ=0,−40a9.
∴ The required circles are
x2+y2−5ax−6ay+9a2=0
x2+y2−5ax−6ay+9a2−40a9(4x+3y−19a)=0
Hence centres are (5a2,3a) and (205a18,29a3)
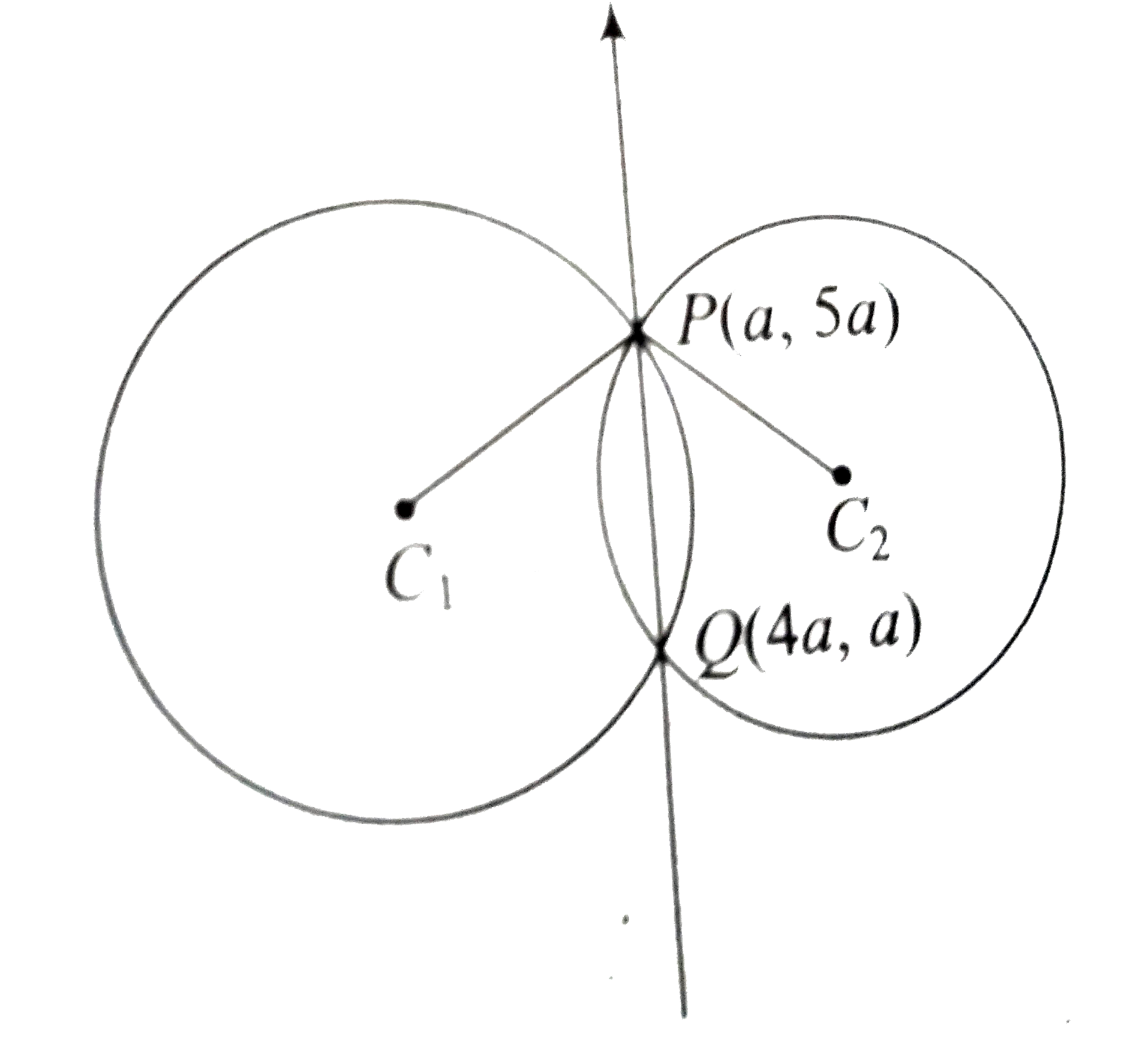
The centres of the given circles are:
C1(205a18,29a3) and C2(5a2,3a)
Now, the angle of intersection θ of these two circles is the angle between the radius vectors a the common point P to the two circles.
i.e., ∠C1PC2=θ
and slope of C2P=5a−3aa−5a2=−43
So, tanθ=84187+431−84187×43=252+748561−336=409
⇒θ=tan−1(409)
Topper's Solved these Questions
- CIRCLESBook:CENGAGEChapter:CIRCLESExercise:Single Correct Answer Type
Explore 58 Videos
- CIRCLESBook:CENGAGEChapter:CIRCLESExercise:Question Bank
Explore 32 Videos
- CIRCLESBook:CENGAGEChapter:CIRCLESExercise:Question Bank
Explore 32 Videos
- CIRCLE Book:CENGAGEChapter:CIRCLE Exercise:JEE Advanced (Single Correct Answer Type)
Explore 14 Videos
- COMPLEX NUMBERSBook:CENGAGEChapter:COMPLEX NUMBERSExercise:Question Bank
Explore 30 Videos
Similar Questions
Knowledge Check
Equation of circle passing through the points (4, 3) and (2,5) and touching the axis of y is
Ax2+y2−20x−22y+121=0Bx2+y2−4x−6y+9=0Cx2+y2−10x−6y+13=0Dx2+y2+4x+6y+9=0Let P(α,β) be a point in the first quadrant. Circles are drawn through P touching the coordinate axes.
Radius of one of the circles isA(√a−√β)2B(√α+√β)2Cα+β−√αβDα+β−√2αβVariable circles are drawn touching two fixed circles externally then locus of centre of variable circle is
AparabolaBellipseChyperbolaDcircle
Centre of the circles passing through the point (−4,3) and touching the lines x+y=2 and x−y=2 is
View SolutionA variable circle passes through the fixed point (2, 0) and touches y-axis then the locus of its centre is
View SolutionFind the equation of the circle passing through the points A(4,3).B(2,5) and touching the axis of y. Also find the point P on the y-axis such that the angle APB has largest magnitude.
View SolutionCentre of a circle passing through point (0,1) and touching the curve y=x2 at (2,4) is
View SolutionP and Q are two points on circle with centre O. The point R is on the minor arcPQ. The tangents from P and Q meet at S. If ∠PSQ=20∘ them ∠PRQ is-
View Solution