Prove that acosA+bcosB+osC≤s.
Video Solution
Text Solution
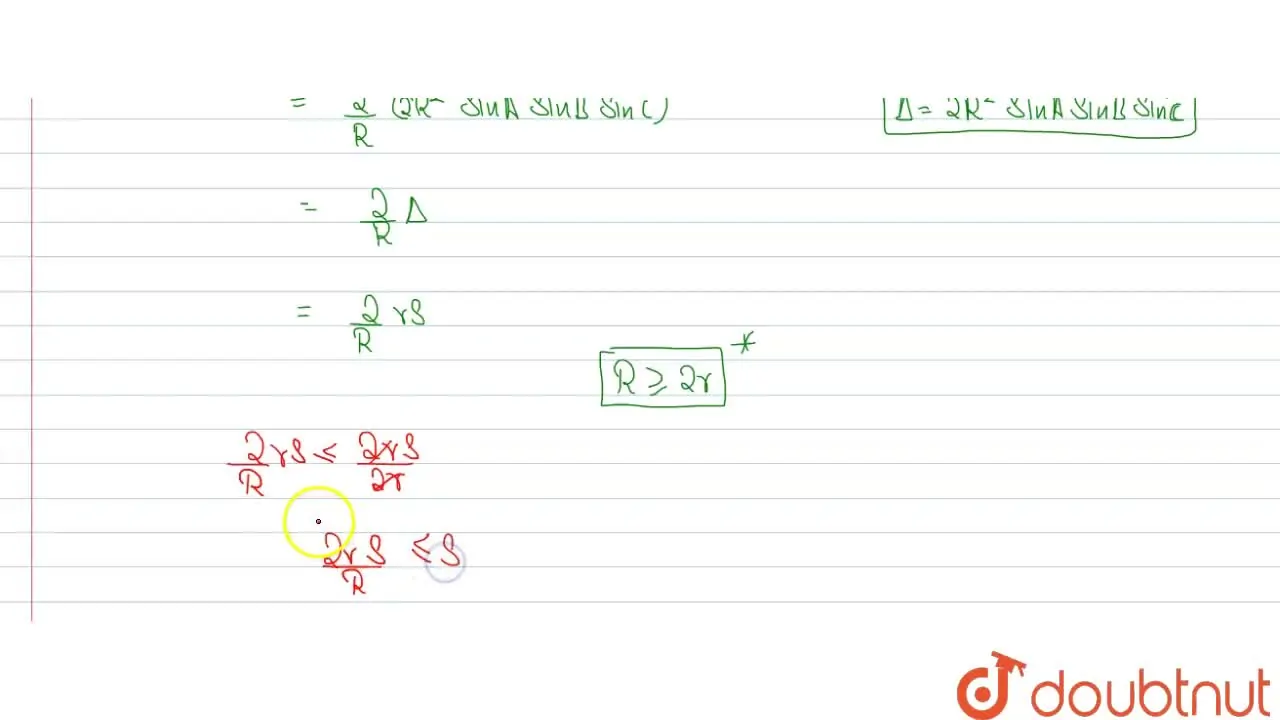
acosa+bcosB+ccosC
=R(2sinAcosA+2sinBcosB+2sinCcosC)
=R(sin2A+sin2B+sin2C)
=4RsinAsinBsinC
=2R(2R2sinAsinBsinC)
=2RΔ
=2rsR≤s [∵R≥2r]
|
Updated on:21/07/2023
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Concept application exercise 5.112videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Concept application exercise 5.28videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (Numerical Value Type)3videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (MATRIX MATCH TYPE )1videos
PROGRESSION AND SERIES
- BOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Archives(Matrix Match Type)1videos
RELATIONS AND FUNCTIONS
Similar Questions
For any triangle ABC, prove that acosA+bcosB+ccosC=2asinBsinC
View SolutionIn any ΔABC, prove that
acosA+bcosB+ccosC=2asinBsinC.View SolutionIn any ΔABC prove that acosA+bcosB+ccosC=8Δ2abc.
View SolutionIn ΔABC, prove that: acosA+bcosB=(c)cos(A−B)
View SolutionProve that
cosAtanA=sinAView SolutionIn any triangle, prove that bcosB+ccosC=acos(B−C).
View SolutionProve that A∪(A∩B)=A
View SolutionProve that : 4 R sinAsinB sinC=acosA+b cosB+ccosC.
View SolutionProve that : 4RsinAsinBsinC=acosA+bcosB+ccosC
View SolutionIn any triangle ABC , prove that following: bcosB+c cos C=a c o s(B−C)
View Solution