Question

Prove that the parallelogram circumscribing a circle is a rhombus.

Explanation

To prove that a parallelogram circumscribing a circle is a rhombus, we will follow a step-by-step approach.

Step 1: Define the Parallelogram
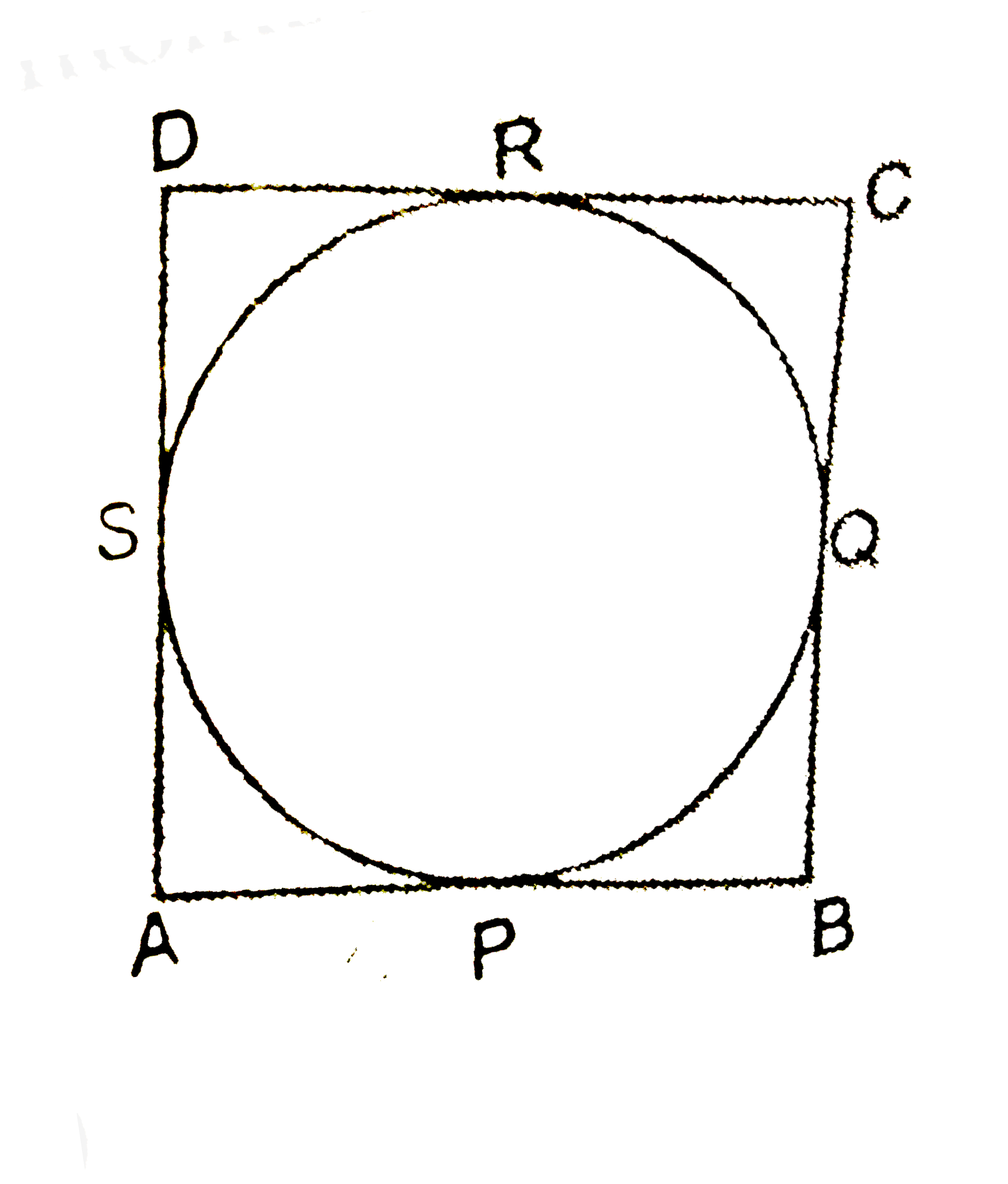
Let \(ABCD\) be a parallelogram that circumscribes a circle. The points where the circle touches the sides of the parallelogram are denoted as \(P, Q, R, S\) on sides \(AB, BC, CD, DA\) respectively.

Step 2: Use the Tangent Properties
According to the properties of tangents from an external point to a circle, the lengths of the tangents drawn from a point outside the circle to the points of tangency are equal. Therefore, we have:
- \(AP = AS\)
- \(BP = BQ\)
- \(CQ = CR\)
- \(DS = DR\)

Step 3: Set the Lengths
Let:
- \(AP = AS = x\)
- \(BP = BQ = y\)
- \(CQ = CR = z\)
- \(DS = DR = w\)

Step 4: Express the Sides of the Parallelogram
Now we can express the lengths of the sides of the parallelogram:
- Side \(AB = AP + BP = x + y\)
- Side \(BC = BQ + CQ = y + z\)
- Side \(CD = CR + DR = z + w\)
- Side \(DA = DS + AS = w + x\)

Step 5: Set Up the Equations
Since \(ABCD\) is a parallelogram, we know that opposite sides are equal:
- \(AB = CD\) and \(AD = BC\)

From the expressions we have:
1. \(x + y = z + w\) (Equation 1)
2. \(w + x = y + z\) (Equation 2)

Step 6: Rearranging the Equations
From Equation 1:
- Rearranging gives us \(x - z = w - y\) (Equation 3)

From Equation 2:
- Rearranging gives us \(w - y = x - z\) (Equation 4)

Step 7: Conclude the Equality of Sides
From Equations 3 and 4, we see that:
- \(x - z = w - y\)
- Thus, we can conclude that \(x + y = z + w\) implies that all four segments \(x, y, z, w\) must be equal.

Step 8: Final Conclusion
Since \(AB = x + y\), \(BC = y + z\), \(CD = z + w\), and \(DA = w + x\) are all equal, we conclude that:
- \(AB = BC = CD = DA\)

This means that the parallelogram \(ABCD\) has all four sides equal, which is the definition of a rhombus.

Final Statement
Therefore, we have proven that a parallelogram that circumscribes a circle is indeed a rhombus.

---