Sam and Jeremy have ages tha are consecutive odd integers. The product of their ages is 783. Which equation could be used to find Jeremy's age, j, if the he is the younger man?
The correct Answer is:C
To find Jeremy's age, we can follow these steps:
1. Define the Variables:
Let Jeremy's age be represented as j. Since Sam is older and their ages are consecutive odd integers, Sam's age can be expressed as j+2.
2. Set Up the Equation:
According to the problem, the product of their ages is 783. Therefore, we can set up the equation:
j×(j+2)=783
3. Expand the Equation:
Expanding the left side gives us:
j2+2j=783
4. Rearrange the Equation:
To form a standard quadratic equation, we can rearrange it as:
j2+2j−783=0
5. Identify the Equation:
The equation we derived, j2+2j−783=0, can be used to find Jeremy's age.
Final Equation:
Thus, the equation that could be used to find Jeremy's age j is:
j2+2j−783=0
Let Jeremy's age be represented as j. Since Sam is older and their ages are consecutive odd integers, Sam's age can be expressed as j+2.
According to the problem, the product of their ages is 783. Therefore, we can set up the equation:
j×(j+2)=783
Expanding the left side gives us:
j2+2j=783
To form a standard quadratic equation, we can rearrange it as:
j2+2j−783=0
The equation we derived, j2+2j−783=0, can be used to find Jeremy's age.
Thus, the equation that could be used to find Jeremy's age j is:
j2+2j−783=0
Topper's Solved these Questions
Similar Questions
The sum of the age of Vivek and his younger brother Amit is 47 years. The product of their ages in years is 550. Find their ages.
View SolutionThe sum of the ages of a man and his son is 45 years. Five years ago, the product of their ages was four times the man’s age at the time. Find their present ages.
View Solution
Knowledge Check
T=2π√Lg
The formula above was created by Italian scientist Galileo Galilei in the early 1600s to demonstrate that the time it takes for a pendulum to complete a swing-called its period, T-can be found using only the length of the pendulum, L, and the force of gravity, g He proved that the mass of the pendulum did not affect its period. Based on the equation above, which of the following equations could be used to find the length of the pendulum given its period ?AL=gT2πBL=gT24π2CL=T24π2gDL=g4π2T2A landscaper buys a new commerial-grade lawn mower that costs $2,800. Based on past experience, he expects it to last about 8 years, and then he can well it for scrap metal with salvage value of about $240. Assuming the value of the lawn mower depreciates at a constant rate, which equation could be used to find its approximate value after x years, given that x<8?
Ay=−8x+2,560By=−240x+2,800Cy=−320x+2,800Dy=240x−2,560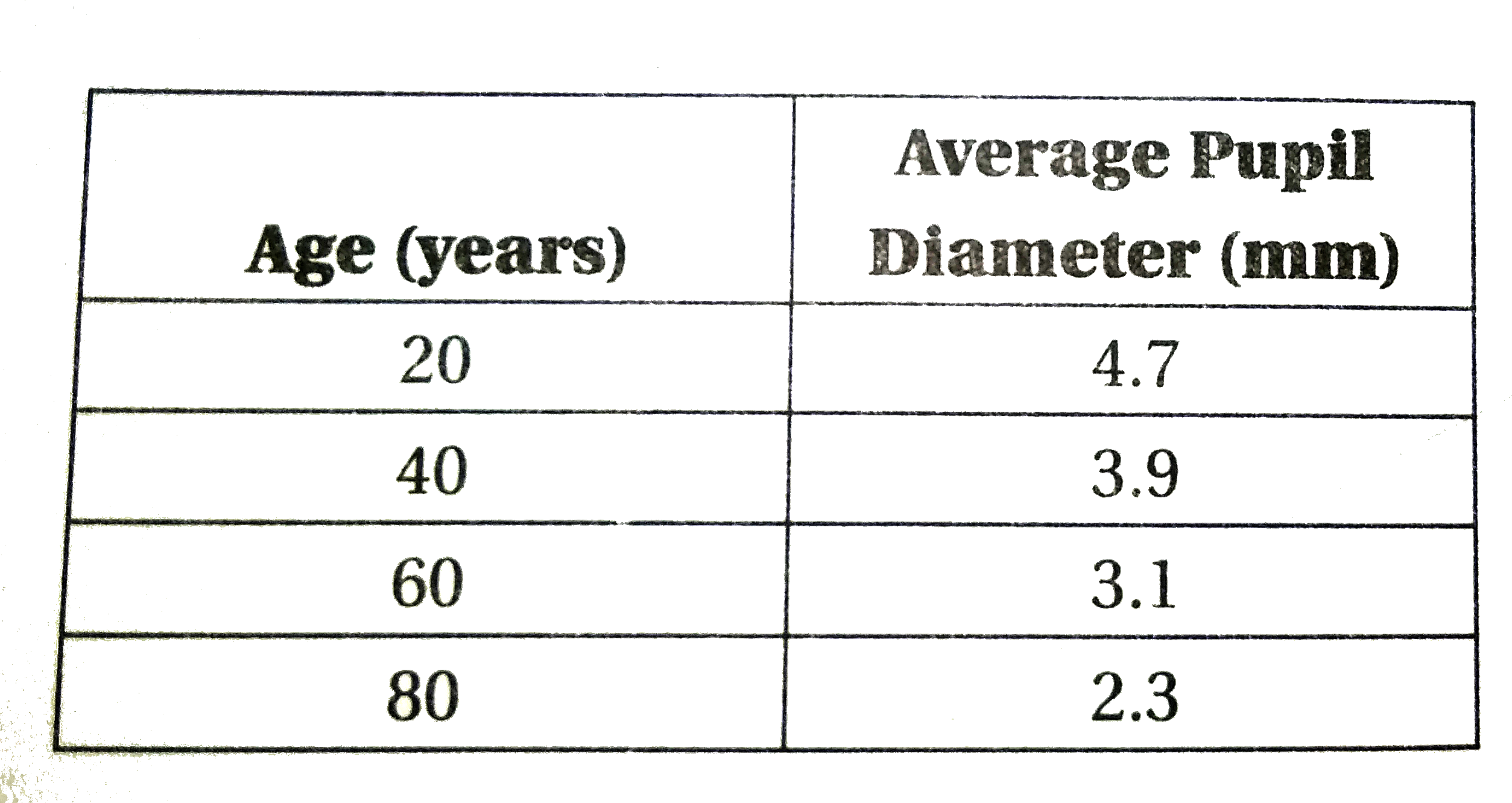
The table above shows the average diameter, in millimeters, of a pupil in a person's eye as she or he grows older from age 20 to age 80. Which equation expresses the relationship between pupil diameter, p, and age a?Ap=−0.04a+5.5Bp=0.04a+3.9Cp=0.04a+34.3Dp=0.235a
Rohan’s mother is 26 years older than him. The product of their ages 3 years from now will be 360. Formulate the quadratic equation to find their ages.
View SolutionRepresent the following situations in the form of quadratic equations :
(i) The area of a rectangular plot is 528 m2. The length of the plot (in metres) is one more than twice its breadth. We need to find the length and breadth of the plot.
(ii) The product of two consecutive positive integers is 306. We need to find the integers.
(iii) Rohan’s mother is 26 years older than him. The product of their ages (in years) 3 years from now will be 360. We would like to find Rohan’s present age.
(iv) A train travels a distance of 480 km at a uniform speed. If the speed had been 8 km/h less, then it would have taken 3 hours more to cover the same distance. We need to find the speed of the train.
View Solutioni) Find the two consecutive positive even integers, the sum of whose square is 340.
ii) Prove that there is a unique pair of consecutive odd positive integers such that sum of their squares is 290 find them.
iii) Find all the numbers which exceeds their square root by 12.
iv) Find the quadratic equation for which sum of the roots is 1 and sum of the squares of the roots is 13.
View SolutionSix years hence a man’s age will be three times the age of his son and three years ago he was nine times as old as his son. Find their present ages.
View SolutionThe more positive the value of Eθ, the greater is the trendency of the species to get reduced. Using the standard electrode potential of redox coples given below find out which of the following is the strongest oxidising agent.
Eθ values: Fe3+/Fe2+=+0.77
I2(s)/I−=+0.54,
Cu2+/Cu=+0.34,Ag+/Ag=0.80V
View Solution
