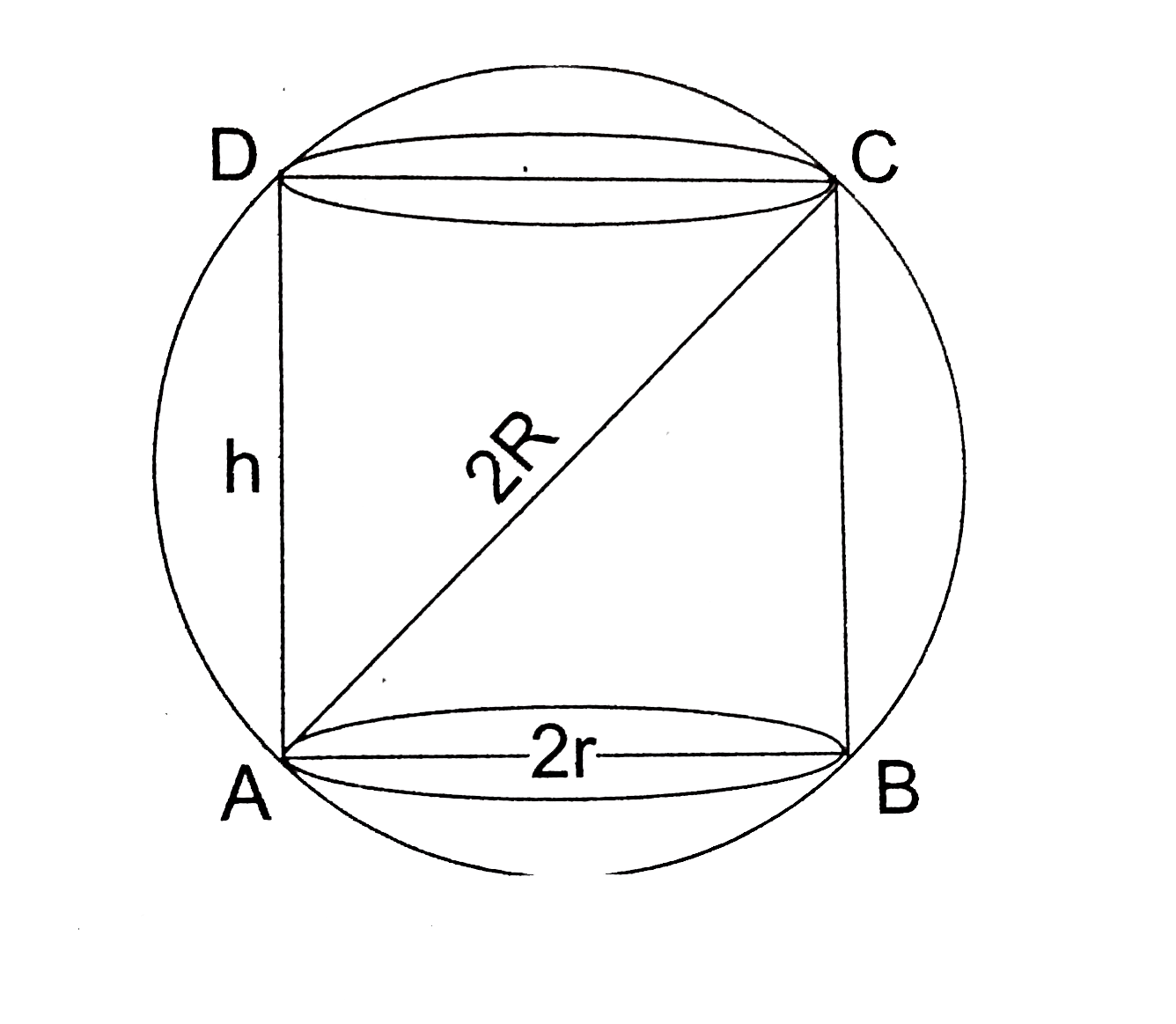
Show that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is $2 \frac{R}{\sqrt{3}}$ . Also find maximum volume.

Video Solution

Text Solution

Verified by Experts

Let r be the radius and h the height of the inscribed cylinder ABCD.
Let V be its volume.
Then, $V = π r^{2} h$ ....(i)
Clearly, $A C = 2 R$
Also, $A C^{2} = A B^{2} + B C^{2}$
$\Rightarrow {(2 R)}^{2} = {(2 r)}^{2} + h^{2}$
$\Rightarrow r^{2} = \frac{1}{4} (4 R^{2} - h^{2})$ ...(ii)
Using (ii) in (i), we get
$V = \frac{π h}{4} (4 R^{2} - h^{2}) \Rightarrow \frac{d V}{d h} = (π R^{2} - \frac{3}{4} π h^{2}) and \frac{d^{2} V}{d h^{2}} = - \frac{3}{2} π h$
For a maxima or minima, we have $(d V / d h) = 0$
Now, $\frac{d V}{d h} = 0 \Rightarrow π R^{2} - \frac{3}{4} π h^{2} = 0 \Rightarrow h = \frac{2 R}{\sqrt{3}}$
$∴ {[\frac{d^{2} V}{d h^{2}}]}_{h = (2 R / \sqrt{3})} = - \frac{3}{2} π \times \frac{2 R}{\sqrt{3}} = - π R \sqrt{3} < 0$
So, V is maximum when $h = \frac{2 R}{\sqrt{3}}$
Hence, the height of the cylinder of maximum volume is $\frac{2 R}{\sqrt{3}}$
Largest volume of the cylinder $= π \times \frac{1}{4} [4 R^{2} - \frac{4 R^{2}}{3}] \times \frac{2 R}{\sqrt{3}} = \frac{4 π R^{3}}{3 \sqrt{3}}$

Updated on:21/07/2023