Show that the opposite sides of a parallelogram are equal.
To show that the opposite sides of a parallelogram are equal, we can use the concept of congruent triangles. Here’s a step-by-step solution:
Step 1: Define the Parallelogram
Let’s consider a parallelogram ABCD, where AB is parallel to DC and AD is parallel to BC.
Step 2: Identify the Triangles
We will focus on triangles ADC and ABC.
Step 3: Show that AC is Common
In both triangles ADC and ABC, the diagonal AC is a common side. Therefore, we can write:
- AC = AC (common side)
Step 4: Establish Parallel Lines and Angles
Since AB is parallel to DC, and AC acts as a transversal, we can say that:
- ∠ACD = ∠CAB (because they are alternate interior angles)
Similarly, since AD is parallel to BC, and AC is again a transversal, we can say that:
- ∠DAC = ∠ABC (also alternate interior angles)
Step 5: Apply the Angle-Angle-Side (AAS) Criterion
Now we have:
1. AC = AC (common side)
2. ∠ACD = ∠CAB (alternate interior angles)
3. ∠DAC = ∠ABC (alternate interior angles)
By the Angle-Angle-Side (AAS) criterion for triangle congruence, we can conclude that:
- Triangle ADC is congruent to triangle ABC.
Step 6: Conclude with Corresponding Parts
Since the triangles are congruent, their corresponding sides are equal. Therefore, we can write:
- AB = CD (opposite sides)
- AD = BC (other pair of opposite sides)
Conclusion
Thus, we have shown that in a parallelogram, the opposite sides are equal: AB = CD and AD = BC.
---
Let’s consider a parallelogram ABCD, where AB is parallel to DC and AD is parallel to BC.
We will focus on triangles ADC and ABC.
In both triangles ADC and ABC, the diagonal AC is a common side. Therefore, we can write:
- AC = AC (common side)
Since AB is parallel to DC, and AC acts as a transversal, we can say that:
- ∠ACD = ∠CAB (because they are alternate interior angles)
- ∠DAC = ∠ABC (also alternate interior angles)
Now we have:
1. AC = AC (common side)
2. ∠ACD = ∠CAB (alternate interior angles)
3. ∠DAC = ∠ABC (alternate interior angles)
- Triangle ADC is congruent to triangle ABC.
Since the triangles are congruent, their corresponding sides are equal. Therefore, we can write:
- AB = CD (opposite sides)
- AD = BC (other pair of opposite sides)
Thus, we have shown that in a parallelogram, the opposite sides are equal: AB = CD and AD = BC.
Topper's Solved these Questions
Similar Questions
The opposite angles of a parallelogram are equal.
View SolutionShow that the points (3, -1, 2), (5, -2, -3), (-2, 4, 1) and (-4, 5, 6) form a parallelogram.
[Hint : Opposite sides of parallelogram are equal.]View SolutionSay True or False :(a) Each angle of a rectangle is a right angle.(b) The opposite sides of a rectangle are equal in length.(c) The diagonals of a square are perpendicular to one another.(d) All the sides of a rhombus are of equal length.(e) All the sides of a parallelogram are of equal length.(f) The opposite sides of a trapezium are parallel.
View SolutionShow that the segment joining the mid-points of a pair of opposite sides of a parallelogram, divides it into two equal parallelograms.
View SolutionX and Y are the mid-points of the opposite sides AB and DC of a parallelogram ABCD. Prove that □AXCY is a parallelogram.
View SolutionP and Q are the mid-point of the opposite sides AB and CD of a parallelogram ABCD. AQ intersects DP at S and BQ intersects CP at R. Show that PQRS is a parallelogram.
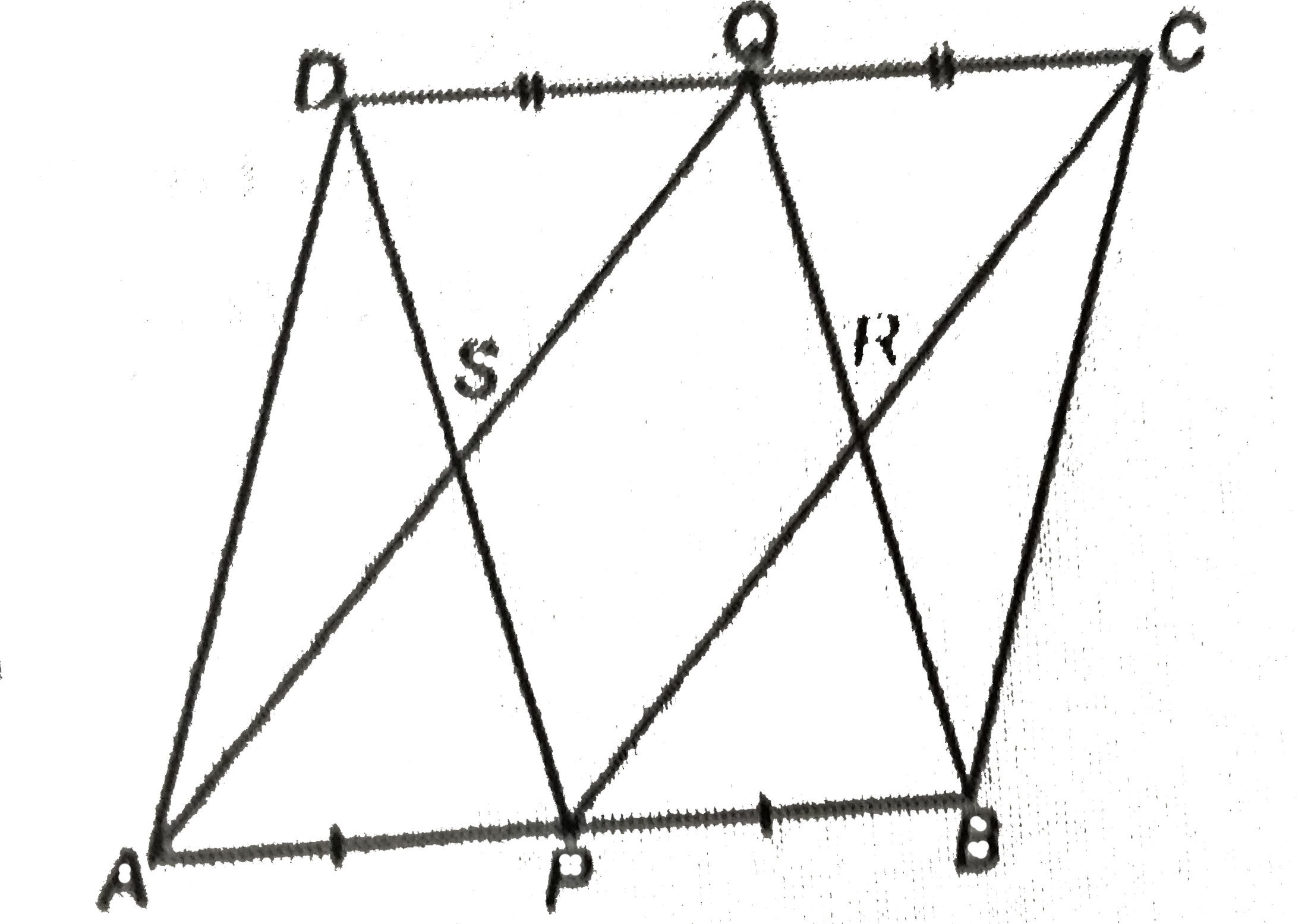 View Solution
View SolutionShow that the segment joining the mid-points of a pair of opposite sides of a parallelogram, divides it into two equal parallelograms. GIVEN : A parallelogram ABCD,E and F are the mid-points of opposite sides AB and CD respectively. TO PROVE : ar(∣∣gmAEFD)=ar(∣∣gmEBCF) CONSTRCUTION : Join EF.
View SolutionState True or False
If two adjacent sides of a parallelogram are equal, it is a rhombusView SolutionProve that the bisectors of opposite angles of a parallelogram are parallel.
View SolutionThe given figure shows parallelograms ABCD and APQR. Show that these parallelograms are equal in area.
[Join B and R]
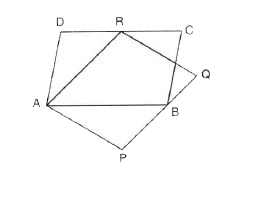 View Solution
View Solution

