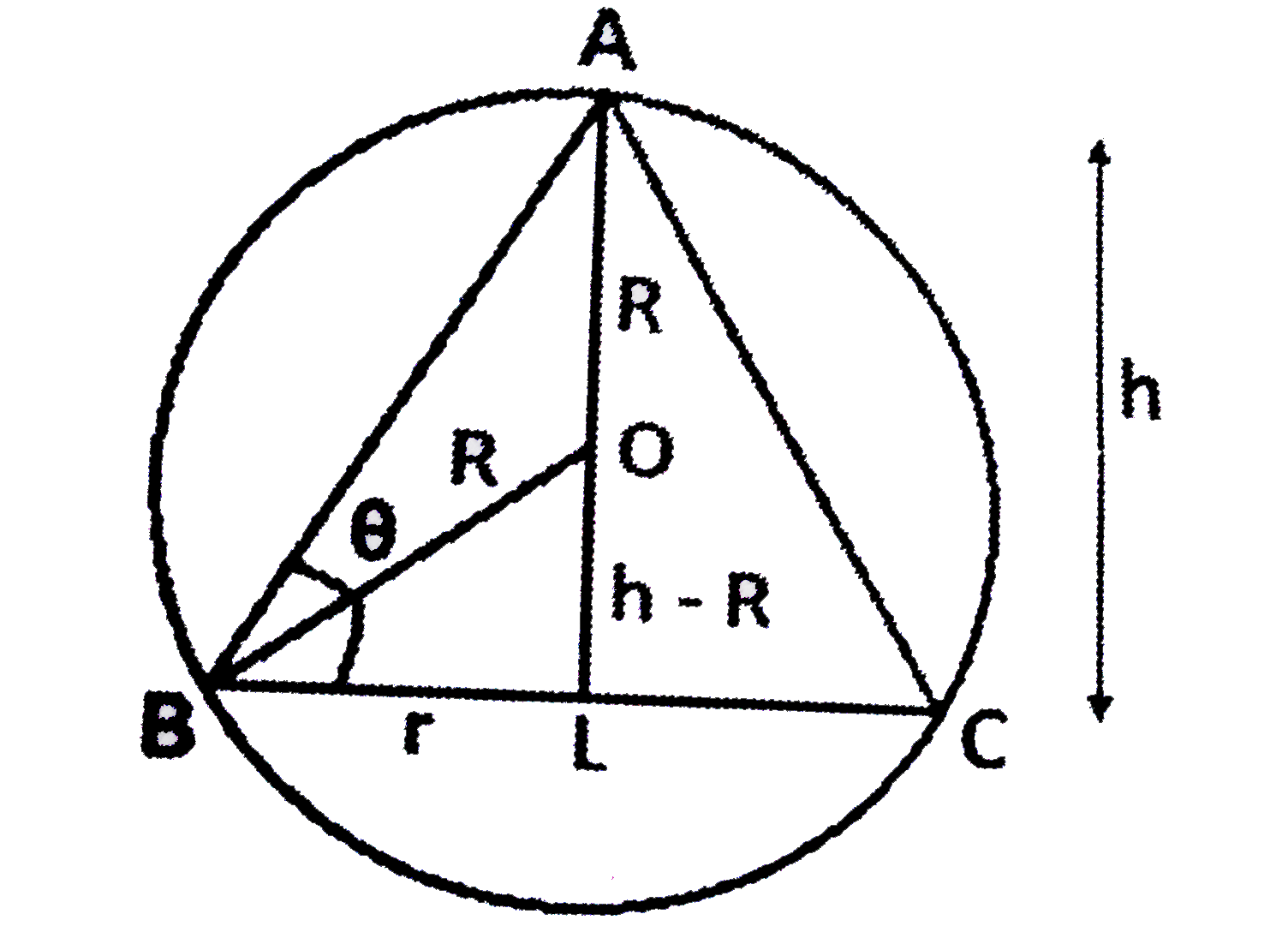
Show that the triangle of maximum area that can be inscribed in a given circle is an equilateral triangle.

Video Solution

Text Solution

Verified by Experts

Let 2r be the base and h be the heightof triangle, which is inscribed in a circle of radius R
Area of triangle= $\frac{1}{2}$ (base)(height)
$A = \frac{1}{2} (2 r) (h) = r h \dots (1)$

Area being positive quantity, A will be maximum or minimum if $A^{2}$ is
maximum or minimum.
$Z = A^{2} = r^{2} h^{2} \dots\dots\dots\dots (2)$
Now In triangle OLB $B L^{2} = O B^{2} - O L^{2}$
In $Δ O B D$
$Z = A^{2} = r^{2} h^{2}, r^{2} = R^{2} - {(h - R)}^{2} \Rightarrow r^{2} = 2 h R - h^{2} put in (2)$
$Z = h^{2} (2 h R - h^{2})$
$\Rightarrow Z = (2 h^{3} R - h^{4})$
$\Rightarrow \frac{d Z}{d h} = 6 h^{2} R - 4 h^{3} ....... (3)$
For maxima/minima $\frac{d Z}{d h} = 0$
$\Rightarrow 6 h^{2} R - 4 h^{3} = 0$
$\Rightarrow 4 R = 4 h (h \neq 0)$
$\Rightarrow h = \frac{3 R}{2}$
differentiating (3) w.r.t . h
$\Rightarrow \frac{d^{2} Z}{d h^{2}} = 12 R - 12 h^{2}$
$\Rightarrow \frac{d^{2} Z}{d h^{2}}]_{h = \frac{3 R}{2}} = 12 (\frac{3 R}{2}) R - 12 {(\frac{3 R}{2})}^{2}$
$= 18^{2} - 27 R^{2} = - v e$
so, $Z = A^{2}$ is maxima when $h = \frac{3 R}{2}$
$\Rightarrow A$ is maximum when $h = \frac{3 R}{2}$
when $h = \frac{3 R}{2}, r^{2} = 2 h R - h^{2} = 2 R . \frac{3 R}{2} - {(\frac{3 R}{2})}^{2}$
$r^{2} = \frac{3 R^{2}}{4}$
$r = \frac{\sqrt{3} R}{2}$
$tan θ = \frac{h}{r} = \frac{\frac{3 R}{2}}{\frac{\sqrt{3} R}{2}} = \sqrt{3} θ = \frac{π}{3}$
Triangle ABC is equilateral triangle

Updated on:21/07/2023

|

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

XII BOARD PREVIOUS YEAR PAPER ENGLISH-SAMPLE PAPER 2019-Section D 35 B

Show that the triangle of maximum area that can be inscribed in a g...
12:42

Home
Ask Doubt
Profile