Solve $(2^{x} - 1) (3^{x} - 9) (sin x - cos x) (5^{x} - 1) < 0, - π / 2 < x < 2 π$ .

Video Solution

Text Solution

Verified by Experts

We have
$(2^{x} - 1) (3^{x} - 9) (sin x - cos x) (5^{x} - 1) < 0, - π / 2 < x < 2 π$ .
$2^{x} - 1 = 0 if x = 0$
$3^{x} - 9 = 0 if x = 2$
$sin x - cos x = 0 if tan x = 1$
$or x = \frac{π}{4}, \frac{5 π}{4}$
$5^{x} - 1 = 0 if x = 0$
The sign scheme of expression $(2^{x} - 1) (3^{x} - 9) (sin x - cos x) (5^{x} - 1)$ is as shown in the following figure.

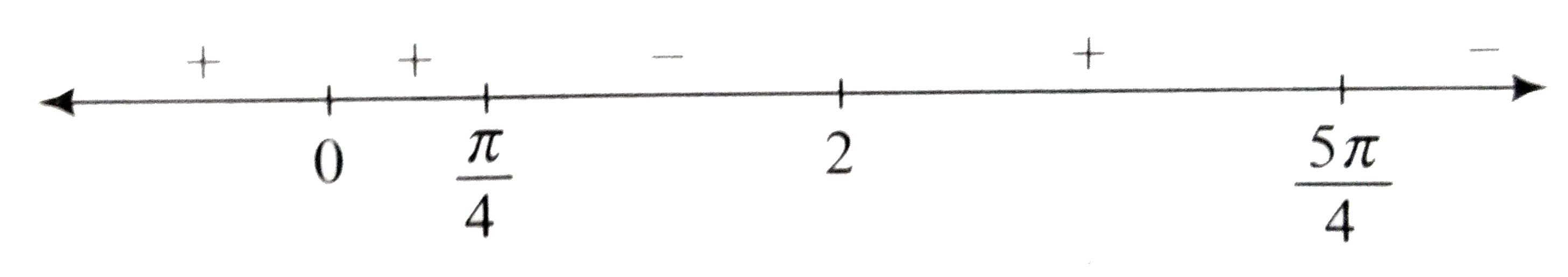
When $x \in (- π / 2, 0),$ each of the factors in the product is negative.
So, expression is positive for $x \in (- π / 2, 0)$ .
At x = 0, sign is not changing as two factors vanishes at $x = 0, 2^{x} - 1$ and $5^{x} - 1$ .
From the sign scheme, we have $(2^{x} - 1) (3^{x} - 9) (sin x - cos x) (5^{x} - 1) < 0$ for
$x \in (π / 4, 2) \cup (5 π / 4, 2 π)$

Updated on:21/07/2023

If $0 < x < \frac{π}{2}$ then
A $cos x > 1 - \frac{2 x}{π}$
B $cos x < - \frac{2 x}{π}$
C $cos x > \frac{2 x}{π}$
D $cos x < \frac{2 x}{x}$
If $0 < x < \frac{π}{2}$ then
A $cos (sin x) > cos x$
B $cos (sin x) < cos x$
C $cos (sin x) = sin (cos x)$
D $cos (sin x) < sin (cos x)$
$I f 0 < x < \frac{π}{2}$ then
A $tan x < x < sin x$
B $x < sin x < tan x$
C $sin x < tan x < x 1$
Dnone of these