Solve (2x+3)(4−3x)3(x−4)(x−2)2x5≤0
Text Solution
The correct Answer is:x∈(−∞,−3/2)∪(0,4/3]∪[4,∞)
(2x+3)(4−3x)3(x−4)(x−2)2x5≤0
or (2x+3)(3x−4)3(x−4)(x−2)2x5≥0
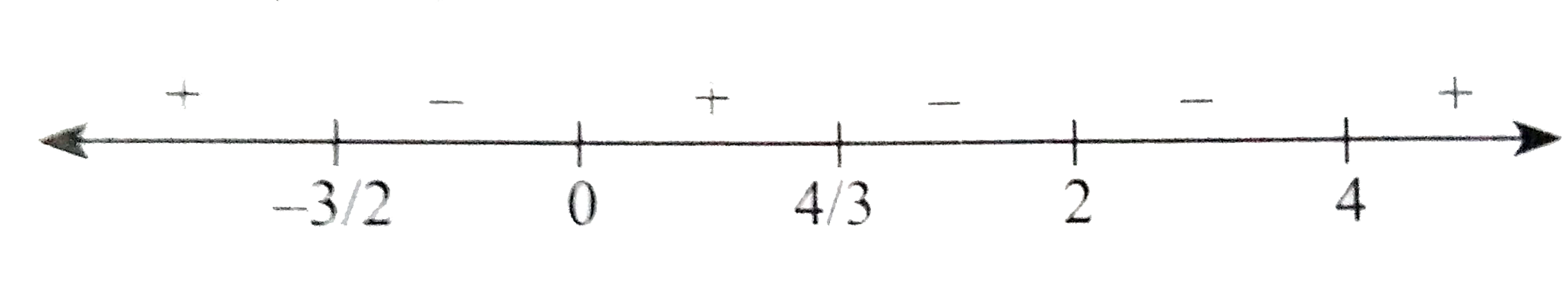
From the sign scheme, we have
x∈(−∞,−3/2)∪(0,4/3]∪[4,∞)
|
Topper's Solved these Questions
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.2
5 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.3
9 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Examples
196 Videos
- Quadratic Equations, Inequalities, Modulus and LogarithmsBOOK - CENGAGECHAPTER - Quadratic Equations, Inequalities, Modulus and LogarithmsEXERCISE - Question Bank
28 Videos
- SCALER TRIPLE PRODUCTS BOOK - CENGAGECHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.3
11 Videos
Similar Questions
Knowledge Check
Sachln Tendulkar has a certain average for 11 innings. In the 12th innings he scores 120 runs and thereby Increases his average by 5 runs. His new average Is:
A62B66C60D65Solve- 3(x−2)5≤5(2−x)3
A(−∞,2)B(−∞,4)C(∞,−2)DNone of these
