Solve : a(x+y)+b(x−y)=a2−ab+b2 a(x+y)−b(x−y)=a2+ab+b2
Video Solution
Text Solution
Given equations can be written as
ax+ay+bx−by=a2−ab+b2
⇒(a+b)x+(a−b)y=a2−ab+b2 ....(1)
and ax+ay−bx+by=a2+ab+b2
⇒(a−b)x+(a+b)y=a2+ab+b2 ....(2)
For equations (1) and (2) by cross multiplication method, we have
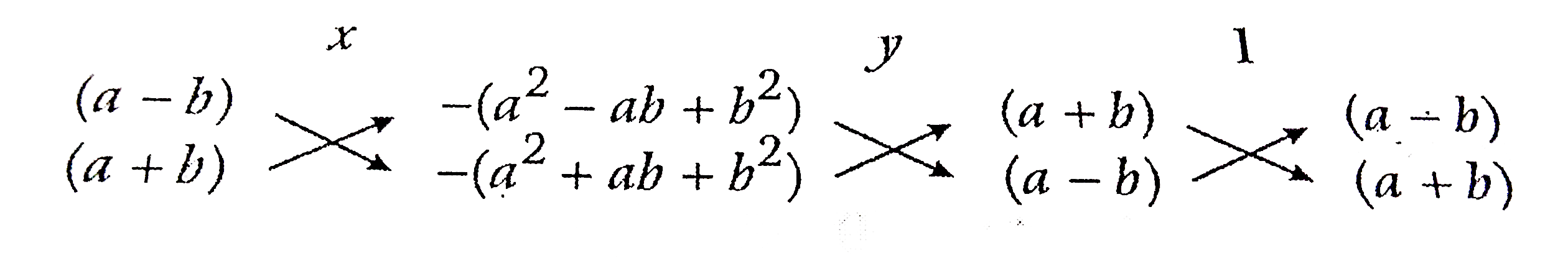
x(a−b)×[−a2+ab+b2]−(a+b)×[−(a2−ab+b2)]
=y[−(a2−ab+b2)]×(a−b)−[−(a2+ab+b2)×(a+b)]=1(a+b)(a+b)−(a−b)(a−b)
implies x−(a3−b3)+(a3+b3)
=y−(a3−a2b+ab2−a2b+ab2−b3)+(a3+a2b+ab2+a2b+ab2+b3)=1(a+b)2−(a−b)2
implies x2b3=y2a2b−2ab2+b3+2a2b+2ab2+b3=14ab
implies x2b3=y4a2b+2b3=14ab
implies x2b3=y2b(2a2+b2)=14ab
when x2b3=14ab⇒x=2b34ab⇒x=b22a
and y2b(2a2+b2)=14ab⇒y=2b(2a2+b2)4ab⇒y=2a2+b22a
Hence, x=b22ay=2a2+b22a⎫⎪⎬⎪⎭ is the required solution.
|
Topper's Solved these Questions
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NAGEEN PRAKASHANCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3a
18 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NAGEEN PRAKASHANCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3b
30 Videos
- INTRODUCTION TO TRIGONOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - INTRODUCTION TO TRIGONOMETRYEXERCISE - Revision Exercise Long Answer Questions
5 Videos
- POLYNOMIALSBOOK - NAGEEN PRAKASHANCHAPTER - POLYNOMIALSEXERCISE - Revision Exercise Long Answer Questions
4 Videos
Similar Questions
Knowledge Check
Solve for x and y.
x+y=a+b
ax−by=a2−b2Ax=b,y=−bBx=b,y=aCx=−a,y=−bDx=a,y=b
Solve: x+y=a+b ax−by=a2−b2
View SolutionSolve for xandy
x+y=a+b,ax−by=a2−b2
View SolutionSolve xb+yb=1 and a(x−a)−b(y+b)=2a2+b2.
View SolutionSolve: a2x+b2y=c2;b2x+a2y=d2
View SolutionSolve: xa+yb=2, ax−by=a2−b2
View SolutionSolve for x and y: x2−y2=xy-ab, (x+y)(ax+by)=2ab(a+b)
View SolutionSolve for 'x' and 'y': (a−b)x+(a+b)y=a2−b2−2ab(a+b)(x+y)=a2+b2
View Solution
