Solve equation is x2−11x+30=0 by using formula.
Video Solution
Text Solution
Given equation is x2−11x+30=0.
The roots of ax2+bx+c=0, are −b±√b2−4ac2a
Here, a=1, b = - 11, and c = 30.
That is,
x=−(−11)±√(−11)2−4(1)(30)2×1=11±√121−1202
x=11±12 ,
x=11+12 and 11−12⇒6 and 5.
∴ The roots are 5 and 6.
|
Topper's Solved these Questions
- QUADRATIC EXPRESSIONS AND EQUATIONSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - QUADRATIC EXPRESSIONS AND EQUATIONSEXERCISE - Very Short
19 Videos
- QUADRATIC EXPRESSIONS AND EQUATIONSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - QUADRATIC EXPRESSIONS AND EQUATIONSEXERCISE - Short Ans
27 Videos
- PROBABILITYBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - PROBABILITYEXERCISE - LEVEL 3
8 Videos
- RATIO, PROPORTION AND VARIATIONBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - RATIO, PROPORTION AND VARIATIONEXERCISE - Leval 3
10 Videos
Similar Questions
Solve the equation:x2−x+2=0
View SolutionSolve the quadratic equation 2x2+5x+2=0 using formula method.
View SolutionSolve using formula :
(i) x2−4x−5=0 (ii) x2−7x−3=0
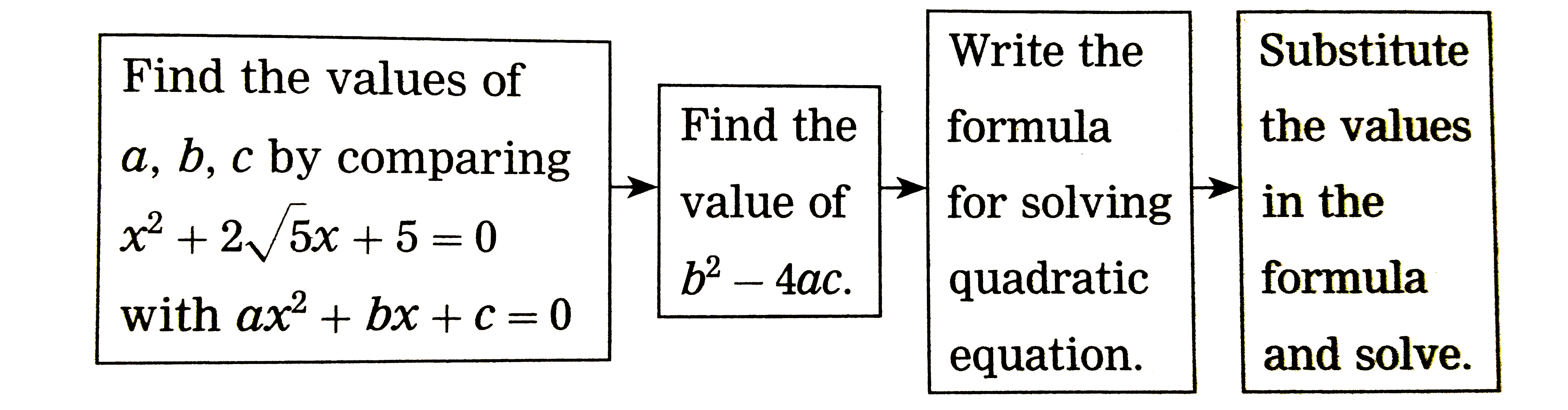
(iii) With the help of flow chart given below solve the equation x2+2√5x+5=0 using the formula :
View SolutionSolve for x:6x2+11x+3=0
View SolutionSolve the equation −x2+x−2=0
View SolutionSolve the equation: 34x2−x−1=0 using formula.
View SolutionSolve the equation 25x2−30x+11=0 by using the general expression for roots quadratic equation ax2+bx+c=0, we get: a=25,b=−30,andc=11 .
View SolutionSolve the quadratic equation 16x2−24x−1=0 by using the quadratic formula.
View SolutionSolve 5x2+11x+6=0
View SolutionWith the help of the flow chart given below, solve the equation x2+2√2x−6=0 using the formula :
View Solution
