Solve: (i) 3x2−4x+203=0.
(ii) x2−2x+23=0.
To solve the given quadratic equations, we will use the quadratic formula:
x=−B±√B2−4AC2A
where A, B, and C are the coefficients from the equation Ax2+Bx+C=0.
(i) Solve 3x2−4x+203=0
1. Identify coefficients:
- A=3
- B=−4
- C=203
2. Calculate the discriminant D=B2−4AC:
D=(−4)2−4⋅3⋅203
D=16−80=−64
3. Since the discriminant is negative, we will have complex roots. Now, apply the quadratic formula:
x=−(−4)±√−642⋅3
x=4±√−646
x=4±8i6
4. Simplify:
x=46±8i6
x=23±4i3
Thus, the solutions for the first equation are:
x=23+4i3,x=23−4i3
(ii) Solve x2−2x+23=0
1. Identify coefficients:
- A=1
- B=−2
- C=23
2. Calculate the discriminant D=B2−4AC:
D=(−2)2−4⋅1⋅23
D=4−83=123−83=43
3. Since the discriminant is positive, we will have real roots. Now, apply the quadratic formula:
x=−(−2)±√432⋅1
x=2±2√32
x=1±1√3
4. To rationalize the denominator:
x=1±√33
Thus, the solutions for the second equation are:
x=1+√33,x=1−√33
Summary of Solutions:
1. For 3x2−4x+203=0:
x=23+4i3,x=23−4i3
2. For x2−2x+23=0:
x=1+√33,x=1−√33
- A=3
- B=−4
- C=203
D=(−4)2−4⋅3⋅203
D=16−80=−64
x=−(−4)±√−642⋅3
x=4±√−646
x=4±8i6
x=46±8i6
x=23±4i3
x=23+4i3,x=23−4i3
- A=1
- B=−2
- C=23
D=(−2)2−4⋅1⋅23
D=4−83=123−83=43
x=−(−2)±√432⋅1
x=2±2√32
x=1±1√3
x=1±√33
x=1+√33,x=1−√33
1. For 3x2−4x+203=0:
x=23+4i3,x=23−4i3
x=1+√33,x=1−√33
Topper's Solved these Questions
- COMPLEX NUMBERSBOOK - MODERN PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - CHECK YOUR UNDERSTANDING
10 Videos
- COMPLEX NUMBERSBOOK - MODERN PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - COMPETITION FILE
17 Videos
- COMPLEX NUMBERSBOOK - MODERN PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE
10 Videos
- BINOMIAL THEOREMBOOK - MODERN PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - COMPETITION FILE (JEE MAIN)
11 Videos
- CONIC SECTIONS BOOK - MODERN PUBLICATIONCHAPTER - CONIC SECTIONS EXERCISE - CHAPTER TEST 11
12 Videos
Similar Questions
Knowledge Check
(i) x2−4x+3=0
(ii) y2−7y+10=0Ax≤yBx<yCx≥yDx>y
Solve: 2x2−4x+3=0
View SolutionSolve x2−4|x|+3<0.
View SolutionSolve for x2−4x+[x]+3=0
View SolutionSolve: (x2−3x)2−16(x2−3x)−36=0
View SolutionSolve (x3−4x)√x2−1=0.
View SolutionSolve using formula :
(i) x2−4x−5=0 (ii) x2−7x−3=0
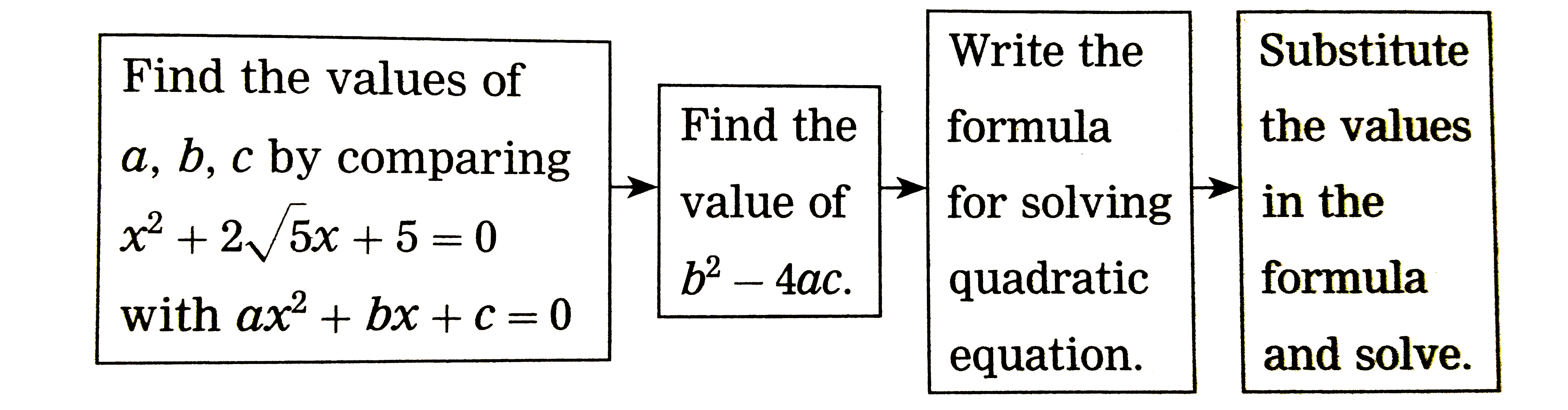
(iii) With the help of flow chart given below solve the equation x2+2√5x+5=0 using the formula :
View SolutionSolve the Equation:
(i) x2+3x+5=0
(ii) x2−x+2=0.
View Solution
