Solve the following linear programming problem graphically:
minimize : $z = x + 2 y$
Subject to: $2 x + y \geq 3$
$x + 2 y \geq 6$
$x \geq 0$
$y \geq 0$
Show that $z$ is minimum at two points.

Video Solution

Text Solution

Verified by Experts

Minimise $Z = x + 2 y$ …………..1
Subject to $2 x + y \geq 3$ ………………..2
$x + 2 y \geq 6$ ……………………..3
$x \geq 0, y \geq 0$ …………….4
First we draw the graph of the line $2 x y = 3$

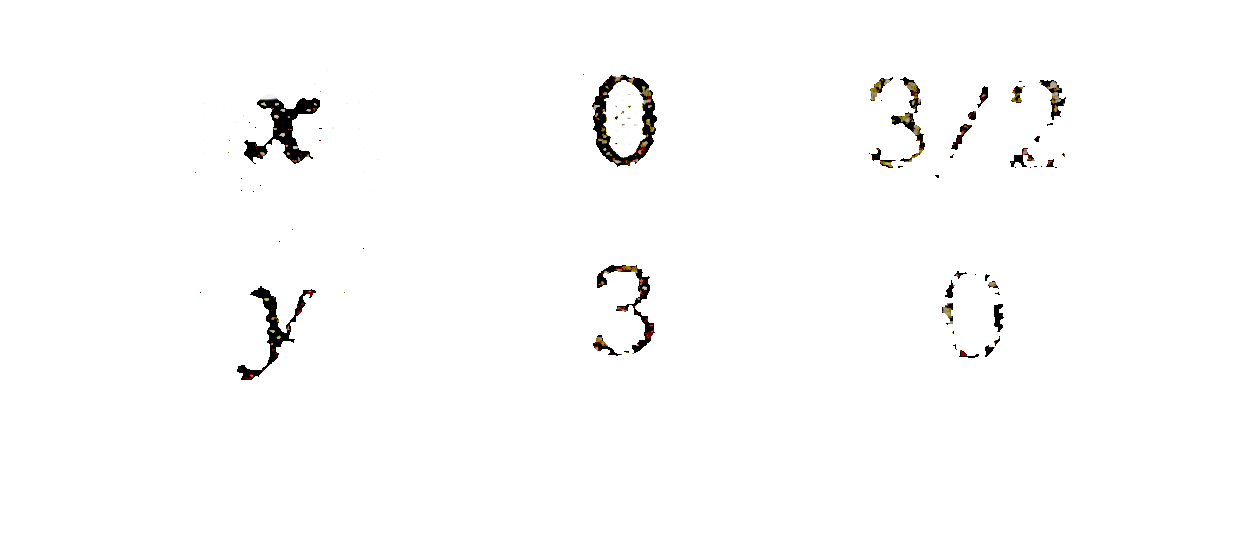
Put $(0, 0)$ in the inequation $2 x + y \geq 3$
$2 \times 0 + 0 \geq 3$
$\Rightarrow 0 \geq 3$ (False)
Therefore, half plane does not contain origin.
Since $x, y \geq 0$
Therefore, feasible region lines in Ist quadrant.
Now, we draw the graph of the line $x + 2 y = 16$

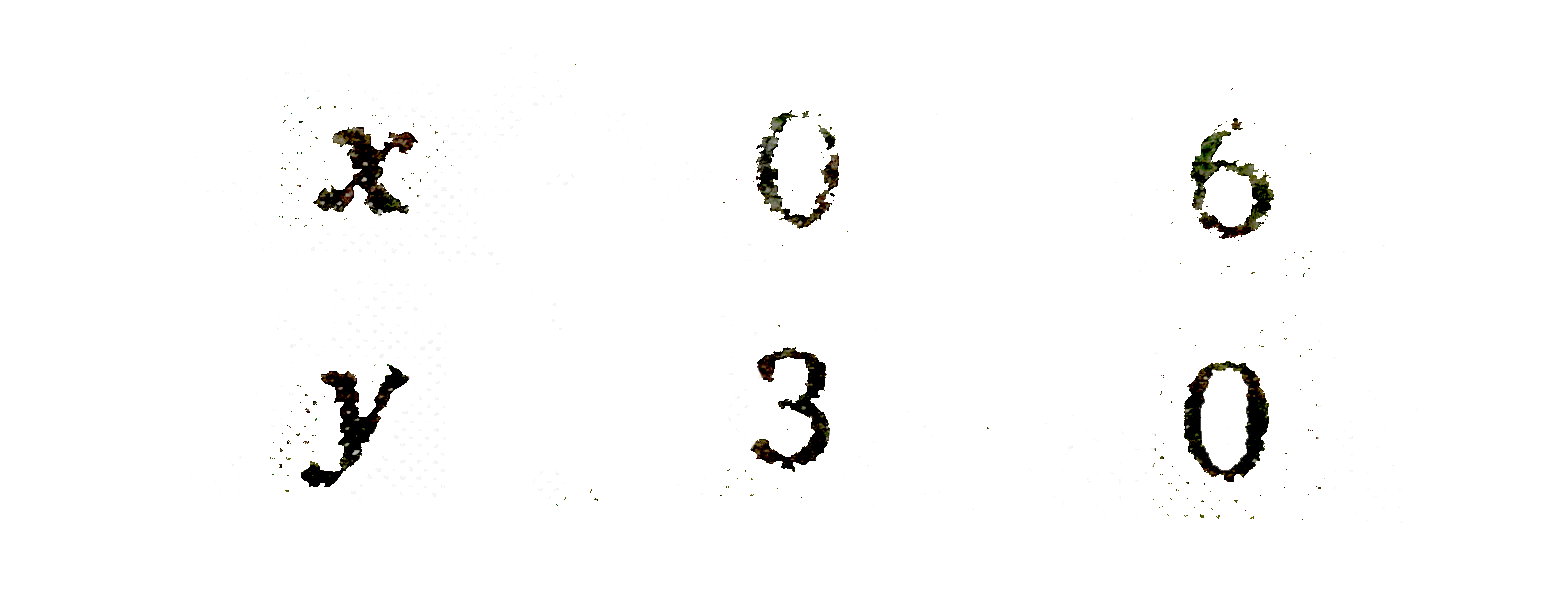
Put $(0, 0)$ in the inequation $x + 2 y \geq 6$ ,
$0 + 2 \times 0 \geq 6 \Rightarrow 2 \geq 6$ (False)
Therefore, half plane does not contain origin.
From the equations $x + 2 y = 6$ and $2 x + y = 3$ , the point of intersection is $B (0, 3)$ .
Thus, vertices of feasible region are $A (6, 0)$ and $B (0, 3)$ . We find the value of $Z$ at these vertices.

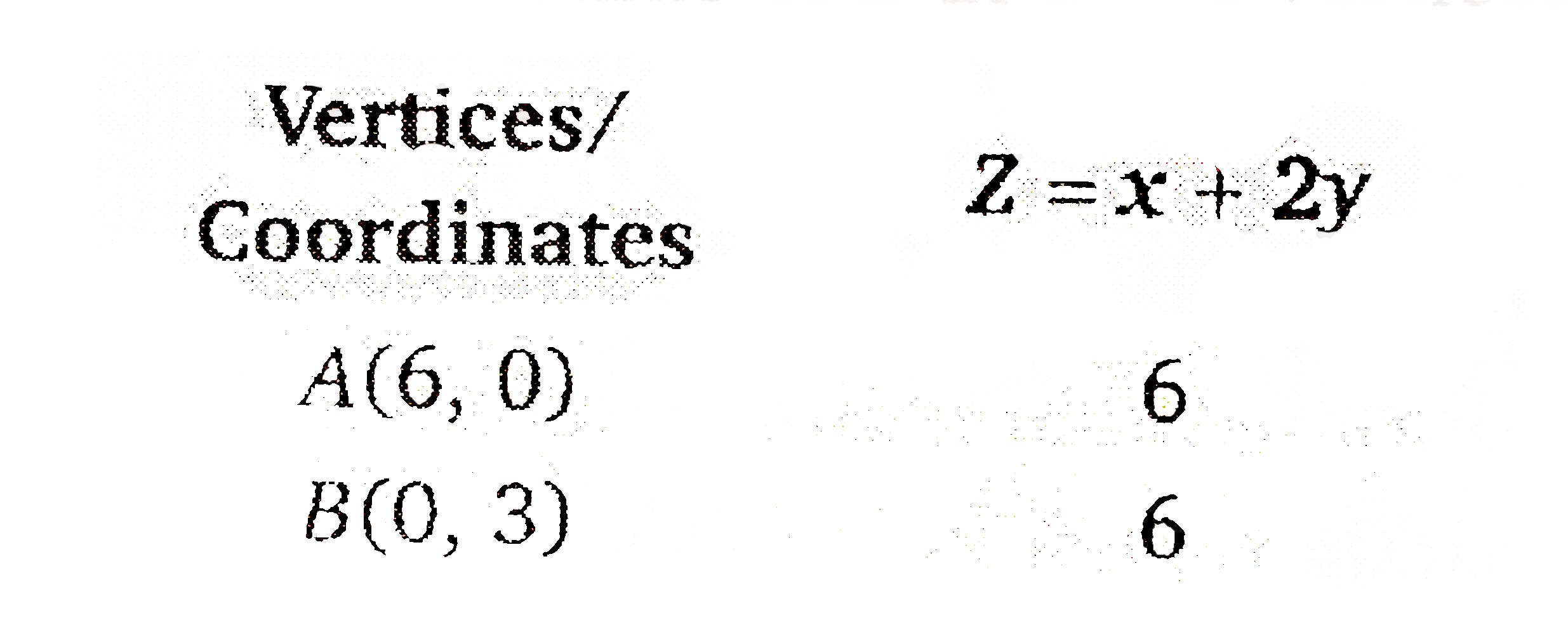
Here, the value of $Z$ at points A and B are same. If we put $(2, 2)$ in the line $x + 2 y = 6$ , we get $Z = 6$ . So the minimum value of $Z$ is obtained at two or more than two points.
Therefore at each point on the line $x + 2 y = 6, Z$ has minimum value.

|

Updated on:21/07/2023

Class 12 MATHS LINEAR PROGRAMMING

Topper's Solved these Questions

Similar Questions

NAGEEN PRAKASHAN ENGLISH-LINEAR PROGRAMMING-Exercise 12.1

Home
Ask Doubt
Profile