Solve the following LPP by using graphical method.
Maximize : $Z = 6 x + 4 y,$
Subject to $x \leq 2, x + y \leq 2, - 2 x + y \leq 1, x \geq 0, y \geq 0 .$ Also find maximum value of Z.

Video Solution

Text Solution

Verified by Experts

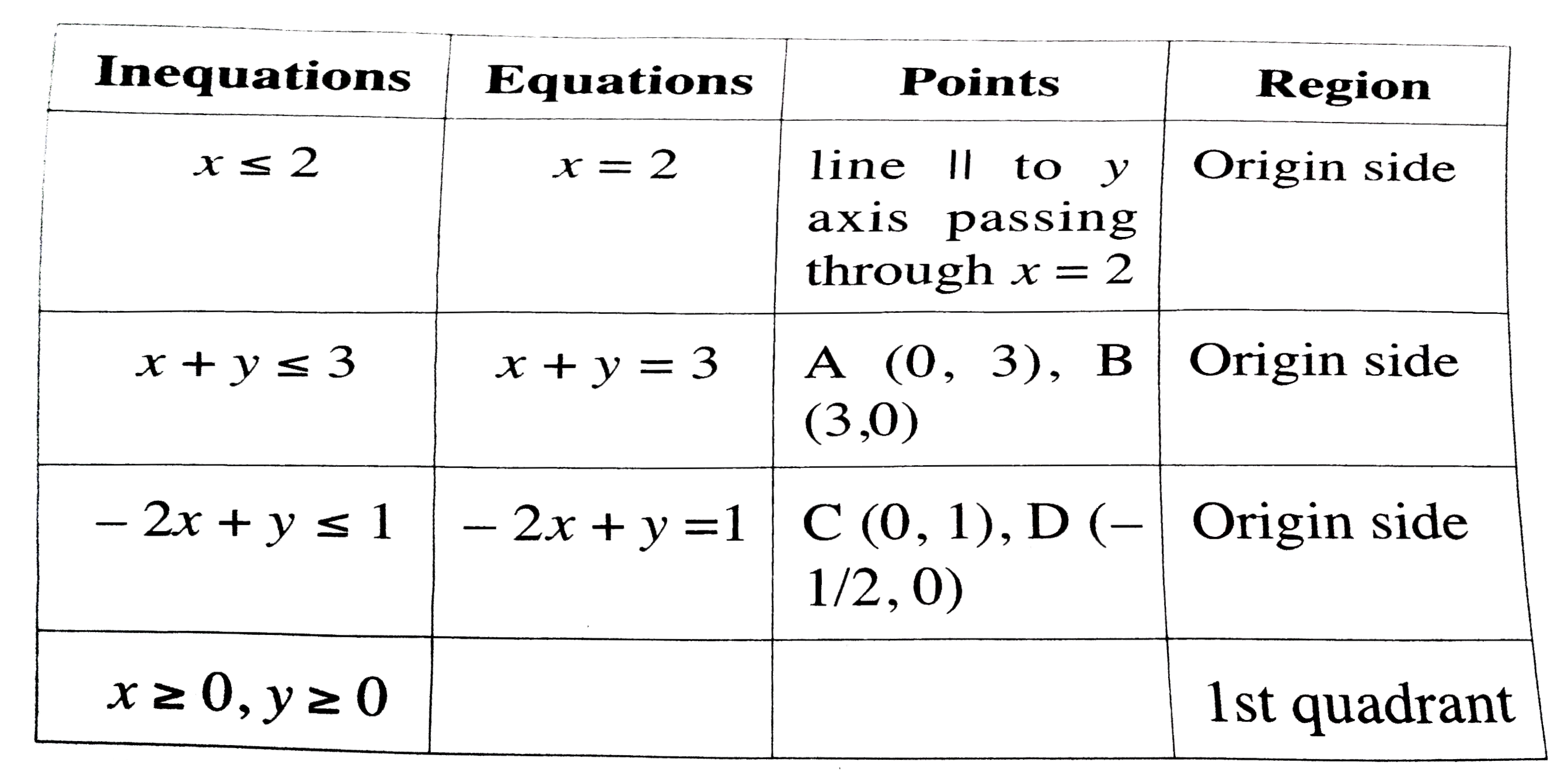
Let E be the point of intersection of lines x=2 and $x + y = 3$ . Solving theses equations we get $E \equiv (2, 1)$
Let F be the point of intersetion of lines $x + y = 3 and - 2 x + y = 1$
Solving theses equations.
$\frac{\begin{matrix} x + y = 3 - 2 x + y = 1 + - - \end{matrix}}{3 x = 2}$
$∴ x = \frac{2}{3}$
$y = 1 + 2 x = 1 + 2 \times \frac{2}{3} = 1 + \frac{4}{3} = \frac{7}{3} ∴ F \equiv (\frac{2}{3}, \frac{7}{3})$
$∴$ Fesiable region is shaded bounded region OGEFCO.
The co-ordinates of the corner points are $0 (0, 0), G (2, 0),, E (2, 1) F (\frac{2}{3}, \frac{7}{3}), C (0, 1)$
Now, $Z = 6 x + 4 y$
$∴ Z (0) = 0 + 0 = 0$
$Z (G) = 6 \times 2 + 0 = 12$
$Z (E) = 6 \times 2 + 4 \times 1 = 12 + 4 = 16$
$Z (F) = 6 \times \frac{2}{3} + 4 \times \frac{7}{3} = 4 + \frac{28}{3} = \frac{40}{3}$

Updated on:21/07/2023

|

Class 12 MATHS MARCH 2017

Topper's Solved these Questions

Similar Questions

Knowledge Check

If Z=2x + y subject to $x \leq 4, y \leq 6, x + y \geq 6, x \geq 0, y \geq 0$ , then the maximum value of Z is
A18
B10
C6
D14

GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERS- MARCH 2017-SECTION-II

Home
Ask Doubt
Profile