Solve the following system of equations
bx + ay = 2ab and ax−by=a2−b2.
Given equations can be written as
bx + ay - 2ab = 0
and ax - by - a2+b2=0
By cross multiplication method, we have
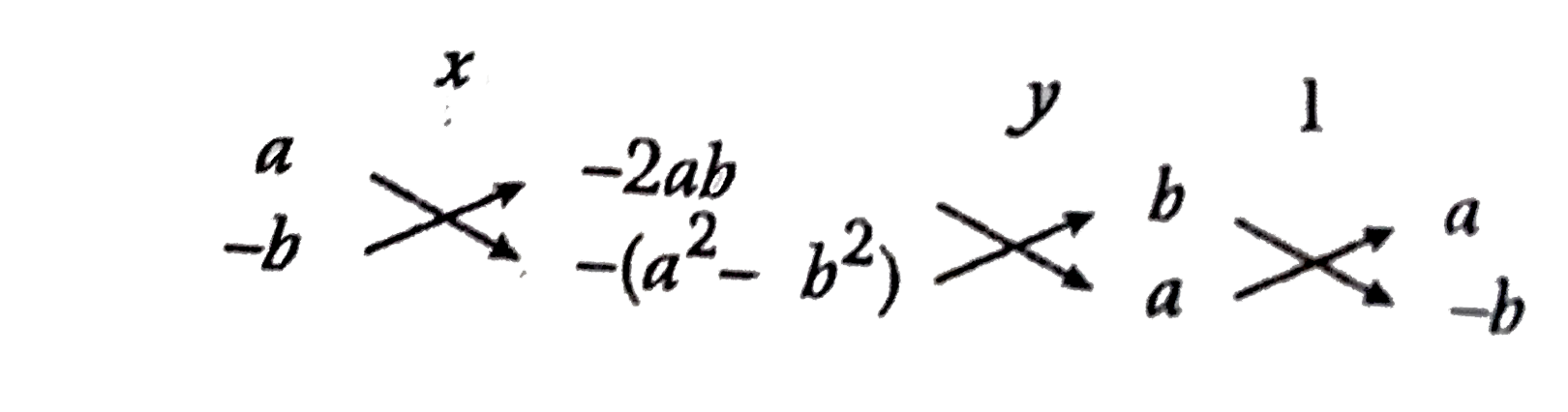
xa×[−(a2−b2)]−(−b)×(−2ab)=y(−2ab)×a−[−(a2−b2)]×b=1b×(−b)−a×a
⇒xa(−a2+b2−2ab2)=y−2a2b+a2b−b3=1−b2−a2
implies xa(−a2−b2)=y−a2b−b3=1−b2−a2
when xa(−a2−b2)=1−b2−a2
⇒x=−a(a2+b2)−1(b2+a2)orx=a
and y−a2b−b3=1−b2−a2
implies y=−b(a2+b2)−1(b2+a2)ory=b
Hence, x=ay=b} is the required solution.
Topper's Solved these Questions
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3a
18 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3A
8 Videos
- INTRODUCTION TO TRIGONOMETRYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - INTRODUCTION TO TRIGONOMETRYEXERCISE - Revision Exercise Long Answer Questions
5 Videos
- POLYNOMIALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - POLYNOMIALSEXERCISE - Revision Exercise Long Answer Questions
4 Videos
Similar Questions
Solve the following system of equations: x+y=a+b, ax−by=a2−b2
View SolutionSolve the following system of equations in xandy ax+by=1 bx+ay=(a+b)2a2+b2−1or,bx+ay=2aba2+b2
View SolutionSolve each of the following system of equation in R:|x+2|x<2
View SolutionSolve the following system of equations by method of cross-multiplication: ax+by=a2, bx+ay=b2
View SolutionSolve the following system of equations by method of cross-multiplication: x(a−b+aba−b)=y(a+b−aba+b), x+y=2a2
View SolutionSolve the following system of equations by using the method of substitution: 2xa+yb=2, xa−yb=4
View SolutionSolve the following system of equations by method of cross-multiplication: bax+aby=a2+b2, x+y=2ab
View SolutionSolve the following system of equations by method of cross-multiplication: xa+yb=a+b, xa2+yb2=2
View SolutionIf a≠b, then the system of equation ax+by+bz=0 bx+ay+bz=0 and bx+by+az=0 will have a non-trivial solution, if
View SolutionSolve the following system of equations by method of cross-multiplication: xa=yb, ax+by=a2+b2
View Solution