Solve the inequality: ${log}_{x} 2 . {log}_{2 x} 2 . {log}_{2} 4 x > 1$

A

$(1, 2^{\sqrt{2}})$

B

$(1 / \sqrt{2}, 1)$

C

$(2^{\sqrt{- 2}}, 1 / 2)$

D

$(2^{\sqrt{- 2}}, 1 / 2) \cup (2, \infty)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Various terms in the given inequation are meaningful iff $x > 0$ and $x \neq 1$ .
Now, $({log}_{x} 2) ({log}_{2 x} 2) ({log}_{2} 4 x) > 1$
$\Rightarrow \frac{{log}_{2} 4 x}{{log}_{2} x . {log}_{2} 2 x} > 1$
$\Rightarrow \frac{(2 + log x)}{{log}_{2} x (1 + {log}_{2} x)} - 1 > 0$
$\Rightarrow \frac{({log}_{2} x + 2) - {log}_{2} x (1 + {log}_{2} x)}{{log}_{2} x (1 + {log}_{2} x)} > 0$
$\Rightarrow \frac{{({log}_{2} x)}^{2} - 2}{{log}_{2} x ({log}_{2} x + 1)} < 0$
$\Rightarrow \frac{({log}_{2} x + \sqrt{2}) ({log}_{2} x - \sqrt{2})}{{log}_{2} x ({log}_{2} x + 1)} < 0$
The signs of the expression
$\frac{({log}_{2} x + \sqrt{2}) ({log}_{2} x - \sqrt{2})}{{log}_{2} x ({log}_{2} x + 1)}$ for different values of ${log}_{2} x$ are shown in Fig.10.

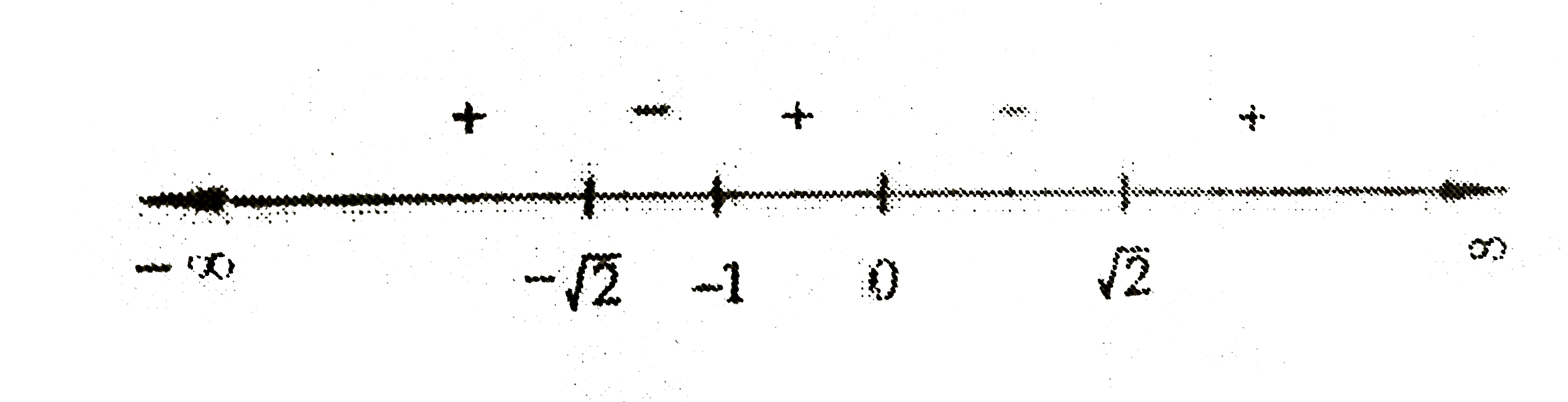
$∴ \frac{({log}_{2} x + 2) - {log}_{2} x (1 + {log}_{2} x)}{{log}_{2} x (1 + {log}_{2} x)} < 0$
$\Rightarrow - \sqrt{2} < {log}_{2} x < - 1 or 0 < {log}_{2} x < \sqrt{2}$
$\Rightarrow 2^{- \sqrt{2}} < x < \frac{1}{2} or, 1 < x < 2^{\sqrt{2}}$
$x \in (2^{- \sqrt{2}}, 1 / 2) \cup (1, 2^{\sqrt{2}})$

|

Updated on:21/07/2023

Class 12 MATHS MISCELLANEOUS EQUATIONS AND INEQUATIONS

Topper's Solved these Questions

Similar Questions

OBJECTIVE RD SHARMA-MISCELLANEOUS EQUATIONS AND INEQUATIONS -Chapter Test

Home
Ask Doubt
Profile