Solve the system of equation in x,yandz satisfying the following equations: x+[y]+{z}=3.1 {x}+y+[z]=4.3 [x]+{y}+z=5.4 (where [] denotes the greatest integer function and {} denotes the fractional part function.)Solution in Bengali
Adding all the three equations, we get
2(x+y+z)=12.8 or x+y+z=6.4 (1)
Adding the first two equations, we get
x+y+z+[y]+{x}=7.4 (2)
Adding the second and third equations, we get
x+y+z+[z]+{y}=9.7 (3)
Adding the first and third equations, we get
x+y+z+[x]+{z}=8.5 (4)
From (1) and (2), [y]+{x}=1.
From (1) and (3), [z]+{y}=3.3.
From (1) and (4), [x]+{z}=2.1. So,
[x]=2,[y]=1,[z]=3,
{x}=0,{y}=0.3, and {z}=0.1
∴x=2,y=1.3,z=3.1
Topper's Solved these Questions
Similar Questions
Let ABCandABC' be two non-congruent triangles with sides AB=4,AC=AC′=2√2 and angle B=300 . The absolute value of the difference between the areas of these triangles is
02:12View SolutionNumber of integral value(s) of λ for which vectors x2ˆi−ˆj+xˆk,(λ−1)ˆi−2λˆj−ˆk and ˆi−ˆj+ˆk, in the order form right-handed system ∀x ∈R, is
(a) 0 (b) 2 (c) 4 (d) 602:53View SolutionThe sum of all the values of x which satisfy the equation 7{x}=2x+[x], where {.} denotes fractional part function and[..denotes greatest integer function
02:18View SolutionSolve the following system of linear equations by Cramers rule: x+y=5, y+z=3, x+z=4
04:30View SolutionIf 0≤[x]<2,−1≤[y]<1and1≤[z]<3, where [⋅] denotes the greatest integer function, then the maximum value of the determinant
∣∣ ∣ ∣∣[x]+1[y][z][x][y]+1[z][x][y][z]+1∣∣ ∣ ∣∣ is -11:47View SolutionSolve : 4{x}=x+[x](where [⋅] denotes the greatest integer function and {⋅} denotes the fractional part function.
View SolutionSolve the following system of equations by matrix inversion method:
2x+3y−4z=−3,1x+2y+6z=2,3x−1y+2z=5View SolutionFind the value of [a] if the lines
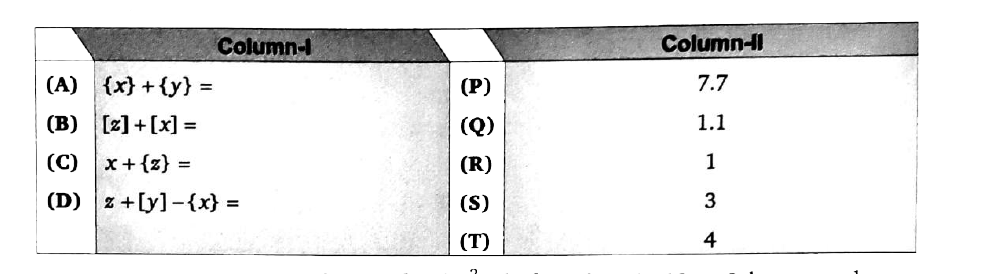
x−23=y+42=z−15 & x+1−2=y−13=z−a4 are coplanar (where [] denotes greatest integer function)05:33View SolutionIf x,y,z∈R satisfies the system of equations x+(y)+(s)=12.7,[x]+{y}+z=4.1and{x}+y+[z]=2
where {.} and [.] denotes the fractional and integral parts respectively) then match the following
 07:27View Solution
07:27View SolutionUsing matrices, solve the following system of equations for x,y and z.
x+y+z=3, y+3z=4, x−2y+z=007:13View SolutionSolve the following system of equations :
1x−1y+2z=73x+4y−5z=−52x−1y+3z=1202:28View SolutionUse matrix method, solve the system of linear equations :
x+y=5, y+z=3, x+z=4.06:56View SolutionSolve : 4{x}=x+[x](where [⋅] denotes the greatest integer function and {⋅} denotes the fractional part function.
03:16View SolutionSolve the following system of linear equations by Cramers rule: x+y=5, y+z=3, x+z=4
08:08View SolutionFind the value of [a] if the lines
x−23=y+42=z−15 & x+1−2=y−13=z−a4 are coplanar (where [] denotes greatest integer function)11:31View SolutionIf x,y,z∈R satisfies the system of equations x+(y)+(s)=12.7,[x]+{y}+z=4.1and{x}+y+[z]=2
where {.} and [.] denotes the fractional and integral parts respectively) then match the following
 05:19View Solution
05:19View Solution

